† Corresponding author. E-mail:
‡ Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant Nos. 11204192 and 11174214) and Jointly supported by the National Natural Science Foundation of China and the China Academy of Engineering Physics (NSAF) (Grant No. U1430117).
The phase transition, elastic and electronic properties of three phases (phase I, II, and III) of Sb2Te3 are investigated by using the generalized gradient approximation (GGA) with the PBESOL exchange–correlation functional in the framework of density-functional theory. Some basic physical parameters, such as lattice constants, bulk modulus, shear modulus, Young’s modulus, Poisson’s ratio, acoustic velocity, and Debye temperature Θ are calculated. The obtained lattice parameters under various pressures are consistent with experimental data. Phase transition pressures are 9.4 GPa (I → II) and 14.1 GPa (II → III), which are in agreement with the experimental results. According to calculated elastic constants, we also discuss the ductile or brittle characters and elastic anisotropies of three phases. Phases I and III are brittle, while phase II is ductile. Of the three phases, phase II has the most serious degree of elastic anisotropy and phase III has the slightest one. Finally, we investigate the partial densities of states (PDOSs) of three phases and find that the three phases possess some covalent features.
Antimony telluride (Sb2Te3) is a member of the (Bi, Sb)2(Te, Se)3 family with a narrow band gap. These layered chalcogenides are typical thermoelectric materials.[1–3] Hence these materials and their alloys are widely studied. Compared with bulk Bi2Te3 alloy, the thin-film thermoelectric material demonstrates a significant enhancement in the figure of merit at 300 K.[4] First-principle calculations show that a uniaxial pressure applied perpendicularly to the plane of the layered structure substantially improves the power factor of Sb2Te3.[5] Calculations also show that under a uniaxial pressure of 1.5 GPa, multiple valence band maximal value occurs which may enhance thermoelectric properties.[6] Owing to the fast speed, excellent endurance, and low-programming energy, Sb2Te3 is a promising candidate for phase change random access memories (PRAMs).[7] Recently, chalcogenides (Sb2Te3, Bi2Te3, and Bi2Se3) have received attention again, because they are predicted to be three-dimensional (3D) topological insulators.[8–10] The topological insulator is a new class of material, which features single Dirac cone at the Γ point.[11]
Pressure can reduce interatomic distances and tune material properties, so high pressure study is helpful to understand properties of materials. Studies of these layered chalcogenides have shown that each of these chalcogenides presents a pressure-induced superconductivity.[12,13] Under ambient conditions, Sb2Te3 has a rhombohedral structure (R
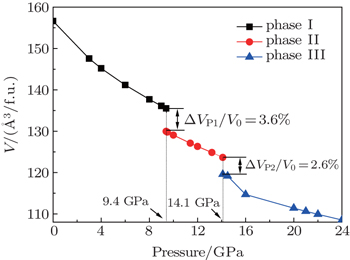
Using XRD experimental technique, Souza et al.[19] observed two high pressure phases at pressures of 9.8 GPa and 15.2 GPa, respectively. They determined that the first high pressure phase was of a monoclinic C2/m structure and the second was of an ordered C2/c structure. Recently, Ma et al.[20] also explored the high pressure behaviors of Sb2Te3 at pressures of up to 52.7 GPa through angle-dispersive synchrotron x-ray powder diffraction (XRPD) technique in a diamond anvil cell and their experimental results showed that the phase (R
In our present work, using the plane-wave pseudopotential density functional theory (DFT) method as implemented in the Cambridge Serial Total Energy Package (CASTEP) code,[22,23] we investigate the phase transition and elastic properties of Sb2Te3 under pressure. Since the calculations of the disordered bcc phase are not possible in the CASTEP code, we just discuss the phases I, II, and III in our following calculations. The remaining parts of this paper are organized as follows. In Section 2, the computation details are presented. The results and discussion are shown in Section 3. Then electronic properties are discussed in Section 4. Finally, a summary of our results is given in Section 5.
Equilibrium properties, such as bulk moduli, phonon frequencies and magnetisms are sensitive to lattice constants. Generally speaking, the lattice constants predicted from PBESOL[24,25] exchange–correlation functional were better than those predicted from the local spin density approximation (LSDA), the Perdew–Burke–Ernzerh (PBE) version of the generalized gradient approximation (GGA) or the Tao–Perdew–Staroverov–Scuseria (TPSS) meta-GGA. Thus the PBESOL was employed for describing the exchange–correlation energy of electrons. The ultrasoft “on the fly” pseudopotential[26] was chosen for the interaction of the electrons with the ion cores, and pseudo atomic calculations were performed for Sb-5s25p3 and Te-5s25p4. The electronic wave functions were expanded in a plane wave basis set with an energy cut-off of 260 eV. For the Brillouin-zone sampling, we used the Monkhorst–Pack meshs[27] 8×8×8, 8×8×3 and 3×3×2 for phases I, II, and III, respectively. In the geometry optimization, criteria of convergence were set to be 5×10−6 eV/atom for energy, 0.01 eV/Å for force, 5×10−4 Å for ionic displacement, and 0.02 GPa for stress. In calculations of elastic properties, criteria of convergence were set to be 1×10−6 eV/atom for energy, 0.002 eV/Å for the maximum force, 1×10−4 for the maximum ionic displacement, and 0.003 GPa for the maximum strain amplitude. These parameters are carefully tested and sufficient to lead to a well converged total energy.
The experimental atomic positions for all phases are presented as follows. The atomic positions of phase I are Sb occupied 6c Wyckoff site (0,0,0.3988), Te1 located at 3a Wyckoff site (0,0,0), and Te2 located at 6c Wyckoff site (0,0,0.7872)[14] under ambient conditions. At 13.2 GPa, the atomic positions of phase II are Sb1 with 4i Wyckoff site (0.1971,0,0.2079), Sb2 with 4i Wyckoff site (0.4599,0,0.2340), Te1 with 4i Wyckoff site (0.2321,0,0.3965), Te2 with 4i Wyckoff site (0.0451,0,0.6106), and Te3 with 4i Wyckoff site (0.3470,0,0.9853).[19] The atomic positions of phase III are Sb with 8 f Wyckoff site (0.278,0.1429,0.8396), Te1 with 8f Wyckoff site (0.5668,0.3853,0.9363), and Te2 with 4e Wyckoff site (0.5,-0.0921,0.75)[20] at 20.5 GPa. These structures are also shown in Fig.
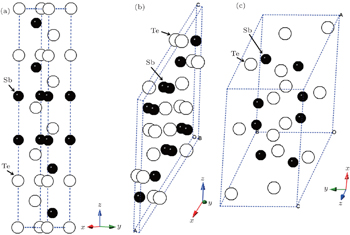 | Fig. 1. Unit cells for (a) phase I at 0 GPa, (b) phase II at 13.2 GPa, and (c) phase III at 20.5 GPa. Phase I is the hexagonal representation. |
| Table 1. The relaxed lattice constants of unit cell of phases I, II, and III under different pressures, together with some experimental results and calculated data. Note that our results of hexagon or unit cells are transformed from the calculated data of rhombohedron or primitive cells. . |
The values of lattice constant a obtained from our calculations accord well with the experimental values[14,16,19] with the errors less than 0.2% and the errors of lattice constant c are about −2.4%. For comparison between the theoretical and experimental results, we present the lattice parameters of phase II at 11.4 GPa and 13.2 GPa and phase III at 20.5 GPa. All these calculated parameters are relatively consistent with the former experimental and theoretical results, which indicate that the selected exchange–correlation functional and pseudopotential are reasonable as well.
To investigate the phase transition of Sb2Te3, Gibbs free energy GGibbs = Einternal+PV −TS will be used, where Einternal, P, V, T, and S are internal energy, pressure, volume, temperature, and vibrational entropy, respectively. At a given pressure P and temperature T, the thermodynamic stable phase has the lowest Gibbs free energy in candidates. In our work, all the calculations are performed at zero K. Hence, the Gibbs free energy is equal to enthalpy H = Einternal+PV and the transition pressure is the value which the crosspoint between enthalpies of two competitive structures corresponds to. To find out transition pressures from phase I to phases II and III, values of enthalpy of three structures are calculated under the pressures in a range of 0 GPa–28 GPa in steps of 4 GPa. Pressure dependences of enthalpy are plotted in Fig.
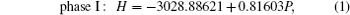
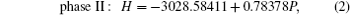
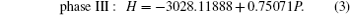
Variations of enthalpy with pressure (a) for phase I to phase II, (b) for phase II to III.
Elastic constant that relates stress to strain is of great importance for a material and helpful for understanding the acoustic velocity, bulk modulus and shear modulus. Therefore, we investigate the elastic constants of all three phases (0 GPa for phase I, 10 GPa for phase II and 15 GPa for phase III). As shown in Table
| Table 2. Calculated elastic constants Cij (GPa) of Sb2Te3 with phase I at 0 GPa, phase II at 10 GPa, and phase III at 15 GPa. . |
From the calculated elastic constants, we can obtain the Voigt average for bulk modulus (BV) and shear modulus (GV), together with the Reuss average for bulk modulus (BR) and shear modulus (GR).[28,29] According to the Voigt–Reuss–Hill approximations,[30] the bulk modulus B and shear modulus G are defined as the arithmetic average of Reuss and Voight values: B = (BR + BV)/2, G = (GR + GV)/2. As shown in Table
| Table 3. Calculated values of bulk modulus B (GPa), shear modulus G (GPa) and B/G. . |
Young’s modulus E can measure stiffness of solid. The larger the value of E, the stiffer the material is. Poisson’s ratio σ gives information about the feature of bonding forces and Debye temperature Θ is a useful parameter and related to many physical properties, such as specific heat and melting temperature. Also it is usually believed that the quantum effect of material is remarkable below Debye temperature Θ, while it can be ignored above Debye temperature Θ. The Young’s modulus E, Poisson’s ratio σ, and Debye temperature Θ can be obtained from[32]
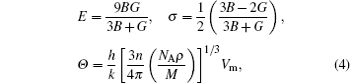
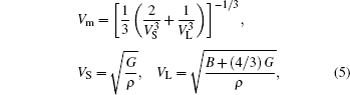
| Table 4. Values of longitudinal acoustic velocity VL (km/s), shear acoustic velocity VS (km/s), average acoustic velocity Vm (km/s), Debye temperature Θ (K), Young’s modulus E (GPa), Poisson’s ratio σ, and universal elastic anisotropy index Au of Sb2Te3. . |
Practically all elastic single crystals are anisotropic to a certain degree. Hence Au, a universal anisotropy index,[35,36] is employed to estimate the elastic anisotropy of Sb2Te3 and expressed as

The partial densities of states (PDOSs) of three phases, i.e., phase I at 0 GPa, phase II at 10 GPa and phase III at 15 GPa are shown in Fig.
In this work, we study the phase transitions, elastic and electronic properties of α-Sb2Te3 (R
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 | |
| 11 | |
| 12 | |
| 13 | |
| 14 | |
| 15 | |
| 16 | |
| 17 | |
| 18 | |
| 19 | |
| 20 | |
| 21 | |
| 22 | |
| 23 | |
| 24 | |
| 25 | |
| 26 | |
| 27 | |
| 28 | |
| 29 | |
| 30 | |
| 31 | |
| 32 | |
| 33 | |
| 34 | |
| 35 | |
| 36 |