Ghost imaging (GI), also known as correlated imaging, is a nonlocal imaging technology which retrieves an unknown object by measuring the second-order intensity correlation function between the test light field and the reference light field. The test light field goes to the object and then is collected by a bucket detector without spatial resolution, and the reference light field is detected by a spatial resolving detector without interacting with the object. The spatially point-to-point correlation of both entangled light source^[1,2] and the thermal light^[3–8] have been applied to achieve GI. GI also touches the fundamental principles of physics and aroused heated debates and discussions.^[9–12] In addition, many different aspects of the GI have been studied, such as high-order GI,^[13–15] compressed sensing GI,^[16–20] differential ghost imaging (DGI),^[21] normalized ghost imaging (NGI),^[22] and so on.^[23–30] In recent years, spatial light modulator (SLM) and digital micro-mirror device (DMD) are being developed, and they have also been employed in GI, for instance in the computational ghost imaging (CGI).^[31,32] Based on the CGI, three-dimensional image and full-color image of the object have been obtained.^[33,34] Furthermore, the influence of atmospheric turbulence, turbid media, or scattering media on GI have also been investigated.^[35–42]

The lensless ghost imaging (LGI), where there is no lens in the optical paths, has drawn much attention in recent years.^[43–50] In x-ray or γ-ray imaging, LGI possesses many advantages since the corresponding optical components, e.g., lenses, are very scarce, and are vulnerable to radiation damage. The longitudinal coherence length of LGI was studied in Refs. [51]–[55]. Zhang et al. studied the LGI of a phase object.^[56] LGIs by measuring reflected photons were reported in Refs. [57] and [58]. For practical applications of LGI, the effects of atmospheric turbulence, turbid media, or scattering media in the optical paths must be studied.^[59] In 2009, Cheng analytically derived a formula for LGI, taking into account of atmospheric turbulence, and showed that the turbulence can result in the degradation of the imaging quality.^[60] In 2011, Shih et al. proposed a turbulence-free GI with non-factorizable, point-to-point correlation of thermal light.^[61] Later, Bina et al. presented a scheme to achieve the backscattering DGI through the turbid media.^[62]

It is desirable to study the influences of the strongly scattering medium on LGI. Ground glass is usually used as a scattering medium in optical systems.^[63–65] Therefore, we use a pane of ground glass disk, which brings strong scatter and even attenuation, as the scattering medium in this work. We report the first (to the best of our knowledge) experimental investigation of the robustness of LGI in a scattering medium.

The paper is organized as follows. In Section 2, we present the experimental results and theory analysis of LGI system. Section 3 encompasses the study of the robust property of LGI when the scattering medium presents in different optical paths. The discussion and summary are given in Section 4.

The experimental setup is depicted in Fig. 1. A laser beam working at λ = 780 nm illuminates a rotating ground glass with an angular frequency at 0.1 rotation per minute (RPM) to produce a pseudo-thermal light beam which is spatially incoherent. Then the pseudo-thermal light beam is divided into two beams by a beam splitter (BS). The test beam interacts with an object and is collected by CCD1 which simulates a bucket detector while the reference beam propagates freely to CCD2. d and d₁ are the respective distances from the light source to the object and to CCD2. The distance from the object to the photosensitive array of CCD1 is d₂. In our experiment we choose d = d₁ = 30 cm and d₂ = 2 cm. The object is a mask of letters THU depicted in Fig. 2(a). To study the robust property of the system, a strongly scattering medium (a pane of 2-mm thick ground glass disk) are placed in three different positions marked A, B, and C which are sketched respectively in Fig. 1. Position A is in the optical path from the object to CCD1, and position B or C is in the optical path from the light source to the object or CCD2. For simplicity, positions A and B are very close to the object and position C is very close to CCD2.

Fig. 1.

Figure Option ViewDownloadNew Window

Fig. 1. Schematic diagram of the lensless ghost imaging system. BS: beam splitter. CCD1, CCD2: charge-coupled device camera (Mintron MTV-1881EX). The ground glass disk is placed in three different positions: (a) behind the object, (b) in front of the object, and (c) in front of CCD2. x1, x2 and y are the transverse coordinates on the test plane, the reference plane and the object plane, respectively.

Fig. 2.

	Figure Option ViewDownloadNew Window
	Fig. 2. (a) The object which is a mask of letters THU. (b) The image captured by CCD1. (c) The ghost image of THU retrieved by calculating the second-order correlation function between CCD1 and CCD2 after averaging over N = 2 × 104 iterations.

In the classical interpretation of GI,^[51] the normalized second-order correlation function between the two spatial points of test and reference beams plays a central role and reads

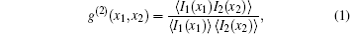
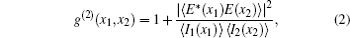
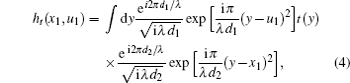
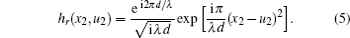
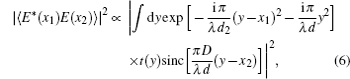
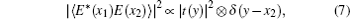
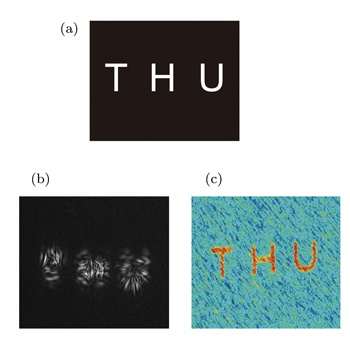
In this experiment, the traditional ghost imaging algorithm, i.e., normalized iteration algorithm is employed to retrieve the image of the object in the LGI,

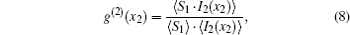
The classical image of THU is directly captured by CCD1 as depicted in Fig. 2(b), and the image is blurry due to the diffraction of the light field. As showed in Fig. 2(c), the image of THU is retrieved by calculating the second-order correlation function between the test and the reference beam according to Eq. (8), after averaging over N = 2 × 10⁴ iterations. It can be seen from the Figs. 2(b) and 2(c) that the LGI with pseudo-thermal light is robust against the diffraction in the optical path from the object to CCD1 which is consistent with Eqs. (6) and (7).

In the following, we report the investigation of the robust property for the LGI system when the strongly scattering medium (a 2-cm thick pane of ground glass disk) is put in the optical path. Firstly, the strongly scattering medium is placed in position A. Then, CCD1 is totally sheltered by the medium. The picture directly captured by CCD1 is showed in Fig. 3(a), so the classical image of THU is thoroughly destroyed by the strongly scattering medium. However, the ghost image of THU can be clearly retrieved by the LGI system as illustrated in Fig. 3(b). Figures 2(c) and 3(b) make it clear that, in position A, the LGI system with a pseudo-thermal light source is robust against the strongly scattering medium. Moreover, even when the ground glass is rotating with different angular frequencies, 0.01 RPM, 0.1 RPM, and 1 RPM, respectively, the quality of the image for the LGI can also be maintained with total iteration number N = 2 × 10⁴ as presented in Fig. 4.

Fig. 3.

	Figure Option ViewDownloadNew Window
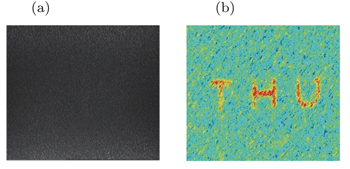	Fig. 3. The strongly scattering medium present in position A: (a) image captured by CCD1 and (b) the ghost image of the THU with N = 2 × 104 iterations.

The robust property against stationary strongly scattering medium in position A of the LGI system is easy to be understood. According to Eq. (8), when the light field has propagated through the object, only the total intensity of CCD1 camera S₁ is useful. Thus, even if the strongly scattering medium exists in position A and causes the diffuse transmission of the test light field, S₁ only needs to be effectively multiplied by a coefficient factor β < 1. Then, equation (8) changes to

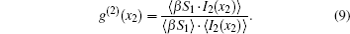
It is seen that β can be reduced, therefore, the LGI system with pseudo-thermal light is robust against the stationary strongly scattering medium in position A. Although CCD1 cannot collect all of the light scattered by the ground glass disk like the real bucket detector, part of the total light intensity is enough to retrieve the image of the object as shown in Fig. 3(b). If the ground glass rotates with a certain angular frequency, the β will change with time: β(t) = β + δ(t), where β is the attenuation term and δ(t) is the fluctuation due to the rotating of the medium. In Eq. (9), β(t) cannot be reduced directly because δ(t) introducing a new noise to the second-order correlation function. However, as long as the fluctuation of β(t) is small and cannot annihilate the fluctuation property of the bucket signal S₁, the LGI system will still work as presented in Fig. 4. In other words, if the roughness of the surface for the strongly scattering medium (ground glass) is distributed evenly, LGI is also robust against the rotating strongly scattering medium in position A.

Fig. 4.

	Figure Option ViewDownloadNew Window
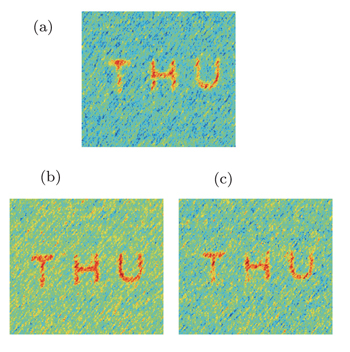	Fig. 4. The ghost image of THU with the ground glass in position A which rotates in different angular frequencies of (a) 0.01 RPM (b) 0.1 RPM, and (c) 1 RPM respectively.

Furthermore, when the strongly scattering medium is presented in other positions of this part of optical path from the object to CCD1, the only change is the value of β when the ground glass disk is stationary or the mean value of the β in the case that the ground glass disk is rotating. Hence, we can infer that not only position A but also the whole optical path from the object to CCD1 are robust against the strongly scattering medium for the LGI system with pseudo-thermal light.

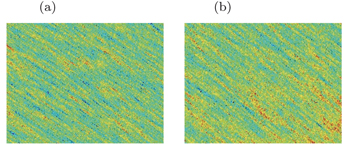
When the strongly scattering medium is located in position B, no effective image could be retrieved from the LGI system as depicted in Fig. 5(a). The reason is that the PSF h(x,u) from the light source to the object cannot maintain the form of Eq. (4) due to the presence of the scattering medium. Therefore, the second-order correlation function between two points of the test and reference beam will not be a sinc or δ function and the spatially point-to-point correlation is not satisfied. Even through the mean value of S₁ in the case of position B is nearly equal to that in the case of position A, the LGI cannot work anymore. When the ground glass disk is very close to the object, the ghost imaging system will retrieve t₁(y) = t(y) × t_glass(y), instead of t(y), where t_glass(y) is the transmission function of the ground glass disk, leading to the original t(y) of the object is hard to obtain. Thus, the LGI system is not robust against the strongly scattering medium in position B. When the strongly scattering medium is located in position C, the result is similar to position B as shown in Fig. 5(b). The PSF h(x,u) from the light source to CCD2 will be radically changed by the strongly scattering medium and the spatially point-to-point second-order correlation will also be destroyed, yielding the collapse of the GI. We also could infer that when the strongly scattering medium is present in the other positions of optical paths from the light source to the object or CCD2, LGI will not work.

Fig. 5.

	Figure Option ViewDownloadNew Window
	Fig. 5. The ghost imaging of THU with N = 2 × 104 iterations when the ground glass disk (a) in position B and (b) in position C.

In summary, we have studied the robustness of the LGI system with a pseudo-thermal light source using a pane of ground glass as the strongly scattering medium. When such a medium is located at position A, the LGI is robust against both the stationary and rotating strongly scattering medium. In the case that the strongly scattering medium is stationary, the total intensity of the test beam S₁ only needs to be multiplied by an attenuation factor of β < 1 which could be reduced in the normalized second-order correlation function. When the strongly scattering medium is rotating, the attenuation factor β will vary with time, leading to β (t) = β + δ(t). However, as long as the roughness of the surface of the strongly scattering medium is distributed evenly, which means that the fluctuation of δ(t) is small, the fluctuation of the bucket signal will not be destroyed and the image quality of the LGI system could be maintained. When the strongly scattering medium is present in position B or C, the image of the object cannot be retrieved by the LGI system because the spatially point-to-point correlation function between the test and the reference beams has been destroyed by the strongly scattering medium.

In addition to the three positions reported here, we have also investigated other positions experimentally, finding that the results are similar to these three cases. These show that in the LGI system with a pseudo-thermal light source, the optical path from the object to CCD1 is of good robustness against the strongly scattering medium while the paths from the light source to the object and to CCD2 are not robust against the strongly scattering medium. This work can help us deepen the understanding of the LGI and will be of great benefit to the application of the ghost imaging.