The thermodynamics of phase transition may be used to estimate whether one kind of phase transition reaction can occur or not. Usually, transformations between different ordering structures or from ordered to disordered structure occur during the process of phase transition. Phase transitions were first classified by Paul Ehrenfest according to the continuity of thermodynamic potential as well as its derivative. Thermodynamic potential can be defined as Γ = F + PV , where F is free energy. The phase transitions during the electrochemical process in LIBs due to Li ⁺ extraction/intercalation have been investigated widely. For this kind of phase transition, Gibbs free energy is adopted. The most direct expression of thermodynamics can be described using the Nernst equation as follows:

One is first-order phase transition, which typically appears in such electrode materials as LiFePO ₄ . Its charge– discharge curves can usually be presented as “L” shapes, indicating a clear potential plateau during Li ⁺ extraction/ intercalation due to formation of a distinct new structural phase. The voltage can be calculated as follows:

The second behaves as a second-order phase transition. A typical charge–discharge “S” shape curve is usually observed, meaning a continuous change in electrochemical potential due to continuous formation of solid solution phases. It can be seen in most of the range of Li ⁺ extraction/intercalation in LiCoO ₂ . The voltage can be calculated as follows:

Phase transition can occur according to the thermodynamics, but this does not mean that the phase transition reaction can really be observed, due to the kinetics limitation. It is associated with the speed of the phase transition reaction. The speed of the electrochemical reaction during the process of Li ⁺ extraction/intercalation is not only related to intrinsic ionic and electronic transfer of materials, but is also related to the interface between electrode and electrolyte. It is a complex and continuous reaction process. The inferior kinetics during the process of Li ⁺ extraction/intercalation can cause huge polarization and thus an incomplete electrochemical reaction or even a disappearance of reaction. In order to improve the phase transition reaction speed of electrode materials, researchers have put forward many solutions, such as decreasing the size of the primary particle ^{[ 3 ]} and foreign element doping, ^{[ 4 ]} to improve the ionic and electronic conductivity of the electrode materials. However, the nanocrystallization method cannot solve all the polarization problems. ^{[ 5 ]} Until now, most of the research work on phase transition during the process of Li ⁺ extraction/intercalation has been performed from the aspect of thermodynamics. With the rapid development of modern advanced characterization tools, especially for micro-, nano- and in situ techniques, kinetic investigations of phase transition reactions are being realized gradually. Therefore, advanced characterization tools become more and more important for thoroughly understanding the nature of phase transition reactions.

The first commercialized cathode material of LIBs is the layered LiCoO ₂ , which still dominates the portable electric device market due to high volumetric density. ^{[ 15 , 16 ]} LiCoO ₂ prepared by a conventional high-temperature solid state reaction method has an O3 structure in which the oxygen anion has a cubic close-packed arrangement in the form of ABCABC. During the electrochemical process, many complex phase transitions, especially in the lithium extraction stage are involved. In the past 30 years, plenty of investigations have focused on these phase transitions to understand the reactions and structural evolution mechanisms. The main investigations with respect to thermodynamics and dynamics are summarized here.

The pioneering work on identification of phase transitions during Li ⁺ insertion/extraction process was carried out by Dahn and Ohzuku et al. ^{[ 17 – 19 ]} As x varies from 1.0 to 0.3, Li _x CoO ₂ evolves mainly in successive solid solution forms, which are considered to be second-order phase transitions. However, during the course, three first-order phase transitions are also clearly observed, as shown in Fig.  2 . Two first-order phase transitions occur at around x = 0.5, which are related to transformation between hexagonal II and monoclinic phases. The driving force for the phase transitions ( x = 0.5) was found to come from the order/disorder transition of Li ⁺ . ^{[ 17 , 20 ]} The third first-order phase transition happens in the range of 0.75 ≤ x ≤ 0.93, which is ascribed to the electronic effect of transitional metal ions. ^{[ 21 , 22 ]} The experimental results show that the two phases were both of hexagonal crystal structure with similar lattice constants, based on the repeated XRD analysis of Li _x CoO ₂ (0.75 ≤ x ≤ 0.93). ^{[ 19 ]} Delmas et al. ^{[ 21 ]} demonstrated that the two phases coexist for 0.75 ≤ x ≤ 0.93, ascribed to a metal–insulator transition rather than structure. The metal–insulator transition was attributed to the electric effect of variation of Co ³⁺ to Co ⁴⁺ , from ⁷ Li MAS NMR results. Afterward, Ceder et al. ^{[ 22 ]} identified the mechanism of this previously anomalous metal–insulator transition as a Mott transition of impurities, via density functional theory (DFT) calculations on large supercells. DFT calculations reveal that for dilute Li-vacancy concentrations the vacancy binds a hole and forms impurity states, yielding a Mott insulator.

Fig. 2.

	Figure Option View Download New Window
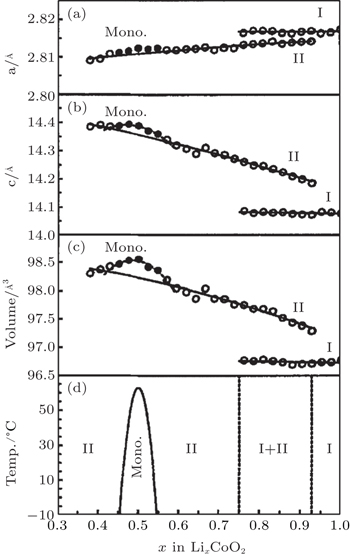	Fig. 2. Unit cell constants (a), (b) and cell volume (c) as functions of lithium concentration x in Li x CoO 2 , as well as a global phase diagram (d) based on the results (a)–(c) for Li x CoO 2 . [ 17 ]

The phenomenon of phase transitions occurring in Li _x CoO ₂ in the low Li content range (0 ≤ x ≤ 0.5) are somewhat difficult to make clear according to the reports. ^{[ 17 – 28 ]} The earlier works by Ohzuku and Ueda ^{[ 19 ]} indicate that the reaction proceeds in a topotactic manner, i.e., two-phase reactions (0 < x < 1/4 and 3/4 < x < 1) and a single-phase reaction (1/4 < x < 3/4) in Li _x CoO ₂ . Another monoclinic phase ( a = 4.91 Å, b = 2.82 Å, c = 5.02 Å, and ß = 111.4°) was observed in 1/4 < x < 0 in addition to that at about x = 0.55 ( a = 4.90 Å, b = 2.81 Å, c = 5.05 Å, ß = 108.3°). Possible lithium ordering at x = 1/4 and 3/4 for this type of material was described in terms of a [2×2] superlattice in a triangular lattice of sites. In 1996, structural characterization of Li _x CoO ₂ with x values was studied for the first time via in situ x-ray diffraction. ^{[ 23 ]} The analysis reveals that as x approaches 0 an increase in crystallographic quality occurs, rather than the expected destruction of the core structure of Li _x CoO ₂ by a drastic increase in structural disorder. For the first time, the end member CoO ₂ phase was isolated and detected. This CoO ₂ phase was certified to be a hexagonal single-layered phase (O1 stacking, Fig.  3(a) ) and had lattice parameters of a = 2.822 Å and c = 4.29 Å. These results are well supported by theoretical calculations from Ceder’s group. ^{[ 24 ]} Ceder et al. investigated phase transitions in the layered oxide Li _x CoO ₂ in a larger range ( x ≤ 0.4) by first principles calculation, giving such different structural types as O1, O3, and H1-3 according to oxygen stacking manner and Li occupation sites, as seen in Figs.  3(a) – 3(c) . As to common LiCoO ₂ , the oxygen planes are stacked according to an ABCABC arrangement, and the host is conventionally referred to as O3 (Fig.  3(b) ). Amatucci et al. ^{[ 23 ]} have verified that O3 is unstable, as it is completely deintercalated, and the hexagonal form of CoO ₂ (referred to as O1) with an ABAB oxygen stacking is obtained, which is consistent with calculated results. ^{[ 24 ]} A hybrid of O3 and O1, named H1-3 (Fig.  3(c) ), is also deduced to be a stable phase. A predicted phase diagram of Li _x CoO ₂ on the basis of a series of phase transitions during Li ⁺ extraction/insertion process is suggested, as displayed in Fig.  3(d) . For Li concentrations between x = 0 and 0.12, the O1 form of CoO ₂ coexists with H1-3 having a Li concentration of x = 0.12. While Li concentrations vary between 0.12 and 0.19, H1-3 is stable as a single phase. In the concentration range from 0.19 to 0.33, H1-3 coexists with O3 phase. When x is over 0.33, only O3 phase appears. However, with a continuous increase of x , other new phases based on the O3 structure can be observed, due to Li ⁺ order/disorder or electronic effects. ^{[ 17 – 22 ]}

Fig. 3.

Figure Option View Download New Window

Fig. 3. Schematic illustration of the three host structures of Li x CoO 2 : O1 (a), O3 (b), and H1-3 (c). The vertices of the octahedra correspond to oxygen ions. Upper case letters describe the stacking of the close-packed oxygen layers. (d) Free energy curves as a function of Li concentration at T = 30°; regions separated by vertical dashed lines indicate the concentration intervals in which different host structures are stable. [ 24 ]

In addition to thermodynamically stable O3 host structures for LiCoO ₂ , a thermodynamically metastable O2- structured LiCoO ₂ was also demonstrated to exist by experimental and theoretical studies. ^{[ 25 – 27 ]} Unlike the O3 structure, the O2 structure must be prepared by the ion-exchange route. In the O2 structure, such new phases as T#2, T#2’ and O6 have been proposed. Usually, a phase transition between O3 and O2 structures is believed to be impossible. Nevertheless, Lu et al. ^{[ 28 ]} first observed O2-Li _x CoO ₂ in the phase diagram of O3-LiCoO ₂ using spherical aberration-corrected scanning transmission electron microscopy (STEM) with high-angle annular-dark-field (HAADF) and annular-bright-field (ABF) techniques. Furthermore, in Lu’s work, O1, O2, and O3 structures were all detected clearly on the basis of HAADF micrographs, as indicated in Fig.  4 . Some different information on phase transitions for Li _x CoO ₂ in the range of 0.5 ≤ x ≤ 1 were presented. The detailed phase transformation of Li _x CoO ₂ (0.5 ≤ x ≤ 1) is revealed in Fig.  4(e) . Recently, direct observation of delithiated structures of Li _x CoO ₂ at atomic scale has become available due to the rapid development of STEM with HAADF and ABF techniques. The phase and structure evolution in a highly delithiated state Li _x CoO ₂ ( x ≤ 0.5) is more important than that of x ≥ 0.5 for achieving higher capacity and energy density to meet the increasing demands for LIBs. Advanced STEM with HAADF and ABF techniques is a new method for researching lithium storage and structure evolution mechanisms in this important LIB cathode material.

Fig. 4.

Figure Option View Download New Window

Fig. 4. STEM-HAADF images of surface structure: (a) pristine LiCoO 2 , (b) LiCoO 2 charged to 4.2 V, (c) charged to 4.5 V, and (d) discharged to 3.0 V at the [010] zone axis. (e) Phase diagram of Li x CoO 2 (1 ≤ x ≤ 0.50) nanoparticle. Numerical symbols 0-4 represent the sample points for STEM observation: (0) pristine LiCoO 2 , (1) charged to 3.9 V, (2) charged to 4.2 V, (3) charged to 4.5 V, and (4) back to 3.0 V. [ 28 ]

We have mainly discussed the phase transitions from the thermodynamics view. However, whether the phase transition can be observed clearly or even occur at all sometimes depends strongly on kinetic factors such as electric conductivity and Li ⁺ chemical diffusion coefficient versus Li ⁺ concentration in Li _x CoO ₂ . Some research activities in those aspects have been performed. Brake et al. ^{[ 29 ]} studied the variation of the Li ⁺ diffusion coefficients for Li _x CoO ₂ as a function of the lithium concentration during the lithium insertion reaction. Kinetics investigation reveals that the average diffusion coefficient for the composite Li _x CoO ₂ is in the range of 10 ⁻⁹  cm ² ·S ⁻¹ , indicating relatively uncomplicated reaction kinetics for the lithium insertion reaction. The variation of the diffusion coefficient in the insertion range 0.3 < x < 0.85 is consistent with filling/removal of Li ⁺ from a single site within the host lattice over this particular composition range. At a composition of around x = 0.65, the diffusion coefficient reaches a local minimum. Within the compositional range described, there exists a two-phase system with the phase transition. ^{[ 17 ]} The structural rearrangement therefore takes place as lithium is inserted or extracted from lattice sites. Subsequently, Ven et al. ^{[ 30 ]} investigated the mechanisms of Li ⁺ diffusion in Li _x CoO ₂ via first principles and found that Li ⁺ diffusion occurs predominantly by a divacancy mechanism. The calculation of the diffusion coefficient at 400 K with kinetic Monte Carlo simulations shows that diffusion efficiency initially increased by several orders of magnitude with x as a result of the decrease in activation barrier with x , as indicated in Fig.  5 . Li ⁺ ordering at x = 0.5 produces a dip in the diffusion coefficient. As x increases beyond 0.65, the diffusion coefficient drops, owing to the decreasing concentration of divacancies.

Fig. 5.

	Figure Option View Download New Window
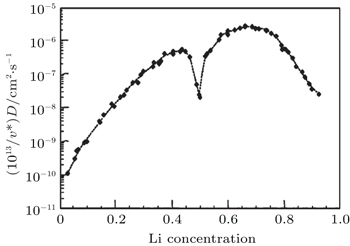	Fig. 5. Calculated diffusion coefficient (D) with lithium concentration, x in Li x CoO 2 . [ 30 ]

Olivine-type LiFePO ₄ , proposed by Goodenough et al. ^{[ 31 , 32 ]} in 1997, has achieved considerable attention due to the chemical stability, safety characteristics, environmentfriendliness and low cost. The crystal structure of LiFePO ₄ can be viewed as an ABAB oxygen sublattice with Li, Fe, and P occupying a subset of octahedral and tetrahedral interstitial sites respectively, having the space group of Pmnb with the following unit-cell parameters: a = 6.011(1) Å, b = 10.338(1) Å, c = 4.695(1) Å. ^{[ 33 – 36 ]} Improving the rate capability of LiFePO ₄ is scientifically interesting and practically significant because of its electronic insulator and mediocre ionic transport property. ^{[ 37 – 42 ]} Next, recent achievements with regard to phase separation and structural evolution mechanisms of LiFePO ₄ involving thermodynamics and kinetics during the Li ⁺ extraction/intercalation process are summarized.

To our knowledge, in terms of the electrode materials, the large variations of Li ⁺ concentration always lead to phase transformations during the charge and discharge processes, such as order–disorder transitions, crystallographic changes and two-phase reactions. ^{[ 43 ]} When cycling at room temperature for LiFePO ₄ , the Li ⁺ extraction from a lithiated triphylite phase, LiFePO ₄ , occurs through a first-order phase transformation to a delithiated heterosite phase, FePO ₄ . ^{[ 44 ]} Phase transformation research has focused on the LiFePO ₄ /FePO ₄ two-phase transition and the existence of Li _α FePO ₄ and Li _{1− β} PO ₄ solid solutions. ^{[ 45 ]} In order to understand the twophase reaction in LiFePO ₄ , Andersson et al. ^{[ 46 ]} introduced two models: “radial model” and “mosaic model”. Both models pinpoint the inactive LiFePO ₄ unconverted in the charge process as the essence of lost capacity in the system. Srinivasan et al. ^{[ 47 ]} developed a “shrinking core” mathematical model to study the cause of low power capacity as shown in Fig.  6(a) . During the charge and discharge processes, the single-phase core (e.g., fully charged FePO ₄ ) was covered with the second-phase shell (e.g., discharge LiFePO ₄ ) with transportation of Li ⁺ , accompanying the phase boundary movement. The ‘domino-cascade model’ in Fig.  6(b) was experimentally observed by Delmas et al. through layer-bylayer intercalation in LiFePO ₄ and used to illuminate the reasons for the high rate performance of the batteries with the poor electronic and ionic conductors, FePO ₄ and LiFePO ₄ . ^{[ 48 ]} These models are introduced on the basis of steady state. The interpretations of the phase transformations vary according to particle shape and size, the (de)lithiation method, and the characterization method. ^{[ 49 ]}

Fig. 6.

	Figure Option View Download New Window
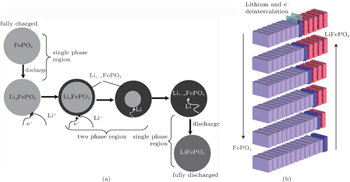	Fig. 6. Illustration of (a) shrinking-core model with the two phases and the movement of the phase boundary. [ 47 ] (b) Layered view of the ‘domino-cascade’ mechanism of the lithium deintercalation/intercalation mechanism in a LiFePO 4 crystallite. [ 48 ]

In contrast to the well-documented two-phase nature of Li _x FePO ₄ at room temperature, Delacourt et al. ^{[ 50 ]} and Dodd et al. ^{[ 51 ]} summarized the phase diagrams shown in Fig.  7 via temperature-controlled XRD, respectively. A solid solution Li _x FePO ₄ (0 ≤ x ≤ 1) at an elevated temperature transforms into a two-phase mixture between LiFePO ₄ and FePO ₄ as the temperature cools down. Beyond the contributions of temperature, particle size, geometry and composition, ^{[ 49 ]} the coherency strains can also be responsible for stabilizing intermediate solid solutions of Li _x FePO ₄ . ^{[ 52 ]}

Fig. 7.

	Figure Option View Download New Window
	Fig. 7. (a) Phase distribution diagrams of Li x FePO 4 (0 ≤ x ≤ 1) established from temperature-controlled XRD data. [ 50 ] (b) Phase diagram of LiFePO 4 (T, for triphylite), FePO 4 (H, for heterosite), and the merging new solid solution (D, for disordered). [ 51 ]

Chen et al. ^{[ 53 ]} developed a phase evolution mechanism in which LiFePO ₄ is transformed into isostructural FePO ₄ , using electron microscopic methods on chemically delithiated LiFePO ₄ . The results show that the lithium is extracted at narrow, disordered transition zones on the ac crystal surface and a substantial lattice mismatch caused cracks to form in the bc plane, leading to a newly created FePO ₄ phase, as shown in Fig.  8(a) . A microscopic mechanism of the phase transition from LiFePO ₄ to FePO ₄ was proposed by Gu et al . ^{[ 54 ]} Lithium cations in LiFePO ₄ were directly detected at atomic resolution by an aberration-corrected annular-bright-field (ABF) scanning transmission electron microscopy (STEM). The pristine, fully charged and half charged states refer to LiFePO ₄ , FePO ₄ and Li ₀ .5FePO ₄ , respectively, as seen in Fig.  8(b1) – 8(b3) . Subsequently, Orikasa ^{[ 55 ]} provided the first experimental observation of a Li _x FePO ₄ metastable phase using time-resolved XRD, as shown in Fig.  9(a) . This indicates that the new Li _x FePO ₄ ( x = 0.6–0.75) transient phase with the estimated lifetime of about 30 min rapidly transforms to the stable LiFePO ₄ and FePO ₄ . The high rate performance of LiFePO ₄ can be attributed to the metastable phase transformation path displayed in Fig.  9(b) , which suggests a fast phase transition. Compared with the model based on relaxed states (white arrow path in Fig.  9(c) ), the mechanism of Li _x FePO ₄ is gradually transformed into the thermodynamically stable FePO ₄ phase as further charged (black arrow path in Fig.  9(c) ). ^{[ 56 ]}

Fig. 8.

Figure Option View Download New Window

Fig. 8. (a) HRTEM image of the disordered region between two phases in Li 0 .5FePO 4 crystal with Fourier transforms of the indicated areas. [ 53 ] ABF micrographs of (b1) pristine (LiFePO 4 ), (b2) fully charged (FePO 4 ) and (b3) half charged (Li 1− x FePO 4 , x = 0.5) materials with the corresponding atomic structure shown in inset. Li sites are marked by yellow circles, and the delithiated sites are marked by orange circles. [ 54 ]

Fig. 9.

	Figure Option View Download New Window
	Fig. 9. (a) Detailed XRD patterns and the first discharge and second charge curves (right). (b) Time-resolved XRD patterns at the end of the first discharge state at different current densities. “Relaxed” represents the pattern of the sample relaxed for 1 day after 10 C charge and discharge cycles. [ 55 ] (c) Phase transition model of Li x FePO 4 . [ 56 ]

We have reviewed some phase transition mechanisms in LiFePO ₄ . However, the occurrence of the phase-transition mechanisms also strongly depends on the limitation of the diffusion of Li ⁺ between the two-phase interfaces. This is related to electrochemical kinetics. Thus, the crystal structure of the compound often plays an essential role in determining the shape of the voltage profile as a function of Li concentration, which is related to the kinetic behavior of the material. Firstprinciples statistical mechanical approaches can be applied to study the chemistry and crystal structures that give rise to kinetic properties. ^{[ 57 ]} A flat voltage plateau (LiFePO ₄ , at 3.45 V versus Li/Li ⁺ ) caused by two-phase electrochemical reaction can be verified from the Gibbs free energy according to the suggestions of Van der Ven et al. ^{[ 58 ]}

Sun et al. ^{[ 59 ]} employed DFT calculations to demonstrate that the stage-II configuration in delithiated LiFePO ₄ is a thermodynamically metastable but kinetically controlled state. A dual-interface model was introduced to describe the delithiation mechanism of LiFePO ₄ upon charging (Fig.  10 ).

Fig. 10.

	Figure Option View Download New Window
	Fig. 10. Schematic view of possible models for the electrochemical delithiation process of LiFePO 4 . [ 59 ]

Yu et al . ^{[ 60 ]} investigated the rapid delithiation behavior of LiFePO ₄ by quick x-ray absorption spectroscopy and dynamically monitored the two-phase transition behavior. As depicted in Fig.  11(c) , 90% of LiFePO ₄ has been converted to FePO ₄ after 120 seconds of chemical delithiation. Electrochemical delithiation of LiFePO ₄ should be similar to chemical delithiation if the electron transport in both LiFePO ₄ and FePO ₄ is faster than ion transport. Compared with Fig.  11(e) , linear combination fitting (LCF) results indicate that more than 60% of Li ⁺ can be extracted in either the chemical or electrochemical delithiation reactions. This similar kinetic property verifies that the Li ⁺ transport is the main governing factor for both reactions. Besides, the Johnson–Mehl–Avrami– Kolmogorov (JMAK) equation f = 1 −exp(− kt ) ⁿ ( f : the fraction of FePO ₄ , k is a rate constant, and n relies on the phase growth geometry) can be used to analyze the kinetics of the phase transition from LiFePO ₄ to FePO ₄ .

The two-phase transformation from LiFePO ₄ to FePO ₄ can be studied in samples with different morphology, particle size and surface coatings, and compared in either chemical or electrochemical delithiation reaction time, and so on. ^{[ 61 ]}

Fig. 11.

Figure Option View Download New Window

Fig. 11. (a) Fe K-edge XANES spectra of pristine LiFePO 4 and after 120 seconds of chemical delithiation; (b) the evolution and (c) linear combination fitting of Fe K-edge XANES spectra. (d) Comparison of fast delithiation of LiFePO 4 between in situ chemical delithiation and 4.3 V constant voltage charging; solid square symbols represent the FePO 4 content, the red solid line is the charge capacity of 4.3 V constant voltage charging. Inset: determination of the Avrami exponent with the Johnson–Mehl–Avrami–Kolmogorov equation; (e) charge curve of LiFePO 4 at different C rates. [ 60 ]

Fig. 12.

	Figure Option View Download New Window
	Fig. 12. Phase diagram of lithium–silicon system. [ 63 ]

Due to their high specific capacity, some anode materials such as Si, Ge, and Sn (as well as alloys containing these elements) attract much attention. Silicon is considered to be the next-generation anode material because of having the highest theoretical capacity (4200 mAh/g) as well as being environmentally benign, naturally abundant, and so on.

A series of alloys of Si and Li were developed, ^{[ 62 – 66 ]} which often resulted in a typical Li–Si diagram as shown in Fig.  12 . In 2000, Li et al. ^{[ 67 ]} investigated the mechanism of Li ⁺ storage in silicon at room temperature, using silicon particles and nanowires. They found that crystalline silicon gradually changes to an amorphous silicon–lithium alloy with insertion of Li ⁺ , and subsequent extraction of Li ⁺ can promote recrystallization of amorphous Si. In 2003, Chiang et al. further reported an electrochemical mechanism driving crystalline silicon to amorphous phase during the insertion of Li ⁺ . ^{[ 68 ]} In 2004, Obrovac et al. used ex situ XRD to demonstrate an amorphous phase arising from crystalline Si on one hand; on the other hand, they also observed the further formation of Li ₁₅ Si ₄ crystalline phase as the discharge voltage lowers to 50 mV. ^{[ 69 ]} In the same year, Dahn et al. adopted in situ XRD methods to study structural change of amorphous silicon films in the charge and discharge process and also found the formation of Li ₁₅ Si ₄ crystalline phase. ^{[ 70 ]} This result was also verified by Grey using nuclear magnetic resonance (NMR). ^{[ 71 , 72 ]} Additionally, Mao et al. used in situ transmission electron microscopy to find the movement of the phase boundary between amorphous silicon (a-Si) and amorphous Li _x Si ( x ∼ 2.5). ^{[ 8 ]} In summary, the specific reaction mechanism of crystalline Si can be described as follows: ^{[ 73 ]} during the initial discharge process:

Carbon coating has been investigated to improve the electrochemical performance of silicon anodes. Generally, it is believed that a carbon coating layer can restrain the merging or agglomeration of silicon nanoparticles and accelerate the lithiation rate, due to improving electron and ion transport. ^{[ 74 – 76 ]} Additionally, it is well known that upon lithiation, both crystalline and amorphous silicon transform to an amorphous Li _x Si phase, which subsequently crystallizes to (Li, Si) crystalline compounds, either Li ₁₅ Si ₄ or Li ₂₂ Si ₅ . ^{[ 76 ]} The latter phase transition from amorphous Li _x Si to crystalline phase results in the huge volume expansion, which is responsible for the electrochemical degradation of silicon anodes. As to the carboncoated silicon, the carbon layer is likely to partly restrain the formation of a crystalline compound, either Li ₁₅ Si ₄ or Li ₂₂ Si ₅ , in that the toughness of carbon can prevent extreme volume change in a Si anode. In other words, the carbon coating layer helps suppress the phase transition from amorphous Li _x Si to a crystalline compound.

Most references report such strategies as carbon-coating or forming composite materials to be effective in improving the electrochemical performance of Si anodes. In our opinion, a double structured silicon anode, core-shell or yolk-shell structured, in which the interior section is porous coated silicon nanoparticles or a Si/C composite and the shell is a superior layer of Li ⁺ and an electron conductor with a stable structure, may be proposed to better enhance the electrochemical properties of silicon anodes.

Another interesting phase transition occurs during the electrochemical lithium extraction/insertion of the anode material β -SnSb alloy. ^{[ 77 , 78 ]} Li et al. synthesized nanosized β -SnSb and found that the lithium first reacted with Sb atoms to form Li ₂ Sb and Li ₃ Sb, and then the remaining Sn atoms were aggregated. After all the Sb changed into Li ₃ Sb, the Li–Sn alloy appeared. In the delithiation process, the reverse steps occur. This special mechanism was clarified by ex situ XRD.