The light-pumped atomic magnetometer is one of the most important high sensitivity magnetometers, ^{[ 1 – 5 ]} it has a sensitivity orders of magnitude better than the fluxgate magnetometer and magnetometers based on the Hall effect ^{[ 6 ]} and the magnet GMR ^{[ 7 ]} effect. Besides the advantage of sensitivity, the atomic magnetometer does not require cryogenic cooling as the superconducting quantum interference device (SQUID) magnetometer, and offers great potential for miniaturization. ^{[ 8 ]}

In the last decade, the spin-exchange relaxation free (SERF) atomic magnetometer ^{[ 9 , 10 ]} has significantly improved the sensitivity of the atomic magnetometers. With the reported sensitivity of 0.16 f·THz ^{−1/2 [ 11 ]} and the projected fundamental limits below 2 a·THz ^−1/2 , ^{[ 12 ]} the sensitivity of the SERF magnetometer rivals and even surpasses that of the best low temperature SQUID magnetometer. ^{[ 13 ]} In recent years, significant progress has been witnessed in the development of the SERF magnetometer. ^{[ 14 , 16 – 20 ]} As a result, the SERF magnetometer has found many important applications where ultra high sensitive magnetic field measurements are required. For example, the precision measurement of the electron electric dipole moments (EDM) can provide critical constraints of parameters for theories beyond the standard model, ^{[ 21 ]} the investigation of the tiny magnetization field in ancient rocks is very important for the paleomagnetism research, ^{[ 11 ]} and the geomagnetic anomalies measurement is a powerful tool in mining and the anti-submarine warfare. ^{[ 22 ]} Furthermore, the SERF magnetometer is well suited for biomagnetic measurements, there are already successful applications of the SERF magnetometer in magnetoencephalography (MEG) ^{[ 23 ]} and magnetocardiography (MCG). ^{[ 24 ]}

In this paper, we present our experimental results of the potassium SERF magnetometer. We will first discuss the SERF mechanism, then we will describe the details of our experimental setup. Because it is very important to identify the SERF and non-SERF regimes, we will next present a pump– probe experiment for this purpose. Finally, we will show our magnetometer signal and the noise spectrum, which indicate a single channel sensitivity of 8 f·THz ^−1/2 .

The sensitivity of the atomic magnetometer is limited by the coherence time of the atomic ensemble, and one of the most important decoherence mechanisms is the spin-exchange collisions. The spin-exchange collisions are the collisions between the alkali atoms which conserve the total spin of the colliding atoms but flip the electron spin of each of the atoms. The spin-exchange collisions lead to random transfers between the two ground state hyperfine levels of the atoms, which have the opposite directions of Larmor precession. When the atoms precess in different directions and at different angular speeds with each other, the total spin of the atomic ensemble become smaller, which leads to the spin relaxation of the atomic ensemble.

Demonstrated by Happer et al ., ^{[ 25 , 26 ]} the effects of the spin-exchange relaxation can be suppressed in the SERF regime, when the spin-exchange rate is much larger than the Larmor precession frequency. In the SERF regime, the atoms go through many spin-exchange collisions during one cycle of Larmor precession, being quickly switched between different ground states, each atom will go through the same average precession rate, so they remain synchronized in the precession process and do not suffer from the decoherence any more.

The experimental setup of the potassium SERF magnetometer is shown in Fig.  1 . The potassium atoms are contained in a cubic glass cell, the size of the cell is 2.5 cm. The cell is filled with 450 torr of ⁴ He gas and 60 torr of N ₂ gas. The ⁴ He gas works as the buffer gas, the buffer gas makes the atoms go through random walks in the glass cell and significantly reduces the wall collisions of the atoms. Since the wall collisions change the atomic spin, the use of the buffer gas can greatly reduce the decoherence rate of the atoms. The N ₂ gas works as the quenching gas to absorb the energy of the states excited by the optical pumping light, so that they will not randomly emit photons which can destroy the polarization of the atomic ensemble; this is very important in an atomic ensemble with a very large optical depth because photons can be trapped in the ensemble and affect multiple atoms before they leave the ensemble. The cell is placed in an oven made by borazon and heated to 150 °C by resistive heating of a 22 kHz AC current. At this temperature, the density of the potassium gas is much higher than that at the room temperature, which leads to a much higher spin-exchange rate and ensures the working condition for the SERF magnetometer. Fig. 1.

Experimental implementation of the K magnetometer. Transverse polarization is detected using optical rotation.

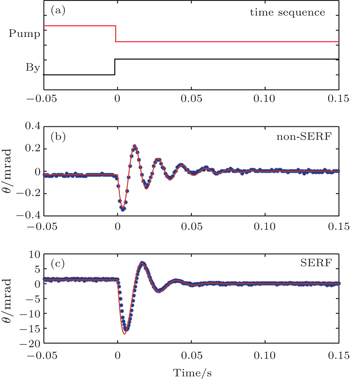
It is very important to clarify whether the magnetometer is running in the SERF regime or not in the experiment. Usually, people can find some evidence from the signal of the magnetometer such as those shown in Fig.  2 , because in the SERF regime, suppression of the spin-exchange decoherence leads to a narrower linewidth. But the linewidth, which is determined by T ₂ , is related to many other experimental conditions, which makes the experimental debugging process much more complicated. Sometimes, it is hard to find which factor makes the greatest contribution to T ₂ . For example, decoherence due to the wall collisions can dominate T ₂ when the buffer gas pressure is inappropriate, the size of the cell is very small, or the coating of the cell does not work. The density of the atomic vapor can be different by orders of magnitude when the temperature of the glass cell changes for just tens of degrees, which will change both the spin-exchange decoherence rate and the spin-destruction decoherence rate by orders of magnitude. The impurities in the atomic gas can lead to significant broadening of the linewidth. Large ambient magnetic field noise can also cause a much broader magnetometer linewidth. So judging whether the atomic magnetometer is running in the SERF regime from the linewidth is not an ideal approach.

In this paper, we use a pump–probe approach to study the spin dynamics in the magnetometer. By observing the time-dependent damped precession signal, we can find direct evidence for whether the magnetometer works in the SERF regime or not. The time sequence of the experiment is shown in Fig.  3(a) . We first apply a 50 ms pump beam to polarize the atom spins along the z direction, then a 9 nT magnetic field is turned on in the y direction, the atomic ensemble would go through free precession in this field. The linear polarized probe beam can measure the spin projection of the atomic ensemble in the x direction, just as done in the SERF magnetometer. The time-dependent solution to the Bloch equation is ^{[ 30 ]}

Fig. 3.

Figure Option View Download New Window

Fig. 3. The pump–probe signal. (a) Atomic spin is first polarized by a 50 ms pump laser pulse and then goes through free precession in a 9 nT bias field. The polarization rotation angles of the detection beam at vapor cell temperatures of (b) 85 °C and (c) 150 °C. The red lines are fitted lines with the Bloch equation. From the fit, the precession frequencies are 63 Hz and 42 Hz, and the relaxation rates are 54 s −1 and 77 s −1 in panels (b) and (c), respectively.

The difference of the spin precession rate in the SERF and non-SERF regimes gives the best evidence of SERF regime in the experiment, because the precession rate is only related to the nuclear spin of the atoms which is precisely known, and the external magnetic field which can be easily measured. The experiment can also be done in the SERF and non-SERF regimes under the same external magnetic field by changing only the temperature of the vapor cell, the ratio of the precession rate is thus not related to the magnetic field, making the result even clearer.

In our experiment, we measure the spin precession rate at the temperatures of 85 °C (Fig.  3(b) ) and 150 °C (Fig.  3(c) ), and the time-dependent signal is fitted to Eq. ( 2 ) to obtain the precession rate and the decay time.

From the fit, in the presence of 9 nT magnetic field, the precession frequency is 63 Hz at 85 °C, which is in good agreement with the Larmor precess frequency of free atoms, but at 150 °C, the precession frequency is 42 Hz, which is clearly slower than the former and in good agreement with the prediction from the SERF theory (Eq. ( 2 )). This is direct evidence for the magnetometer running in the SERF regime. The relaxation rate of the spin polarization does not have a significant difference from 85 °C to 150 °C, even when the spin-exchange rate is increased by 75 times. For n _k ≈ 1.8 × 10 ¹³  cm ⁻³ at 150 °C, obtained from the saturated vapor pressure curve, the spin-exchange rate is about R _SE = 15818 s ⁻¹ , which corresponds to a lifetime of 63 μs. From Fig.  3(c) , we obtain a relaxation rate of 77 s ⁻¹ and a lifetime of T ₂ = 21 ms, which indicate that the spin-exchange relaxation effect is eliminated.

In order to calibrate the sensitivity of the SERF magnetometer, a sinusoidal calibration field of B _rms = 120 pT at 20 Hz is applied, the sensitivity data are obtained by recording the output of the magnetometer for 100 s, performing a fast Fourier transform (FFT) without windowing, and calculating the r.m.s. amplitudes in 1 Hz bins, ^{[ 9 ]} as shown in Fig.  4 . Fig. 4.

Noise spectrum of the magnetometer signal with B _y = 120 pT applied at 20 Hz, giving a sensitivity of 8 f·THz ^−1/2 .

In summary, we have successfully set up a potassium atomic SERF magnetometer and achieved a magnetometer sensitivity of 8 f·THz ^−1/2 . A pump and probe experiment is conducted to measure the spin precession frequency and relaxation time, which clearly prove that our magnetometer is working in the SERF regime. The sensitivity of our magnetometer ranks among the best in the world, further research and application of the SERF magnetometer will be possible in the near future.