†Corresponding author. E-mail: akudreyko@rusoil.net
*Project supported by the Russian Foundation for Basic Research (RFBR) (Grant No. 14-02-97026).
Helix unwinding in ferroelectric liquid crystals induced by an electric field is theoretically studied on the basis of the continuum theory. By applying a weak electric field tilted to the smectic layers, the contribution of the dielectric interaction energy density to the total free energy density is increased. Approximation methods are used to calculate the free energy for different tilt angles between the electric field and the smectic layers. The obtained results suggest selecting the optimal number of pitches in the film that matches to the minimum of the free energy.
Liquid crystals with spontaneous electric polarization were discovered in 1975, and researchers managed to transform them into ferroelectric samples five years later.[1] A considerable interest in the study of smectic liquid crystals has been expressed by the scientific society in the present time. In particular, this interest is related with the fact that the so-called smectic C* (SmC*) exhibits spontaneous polarization.[2, 3]
Due to their helical structures and absence of the flexoelectric effects, [4] ferroelectric liquid crystals (FLC) represent promising functional materials in systems for visualization of information and light modulators.[5] In addition, the smectic films are model objects for the study of frustration effects, [6] which are responsible for the formation of multilayer structures, ultra-thin films, and self-organization of particles in two-dimensional systems.
The applied electric field to a helical SmC* gains energy of spontaneous polarization, which generates unwinding of the helix. The corresponding electric field for the complete helix unwinding, Eu, is called the critical field. In order to obtain a suitably tractable model for simulations, many approaches have been developed for understanding helix unwinding, which take several simplifications.[7, 8] For example, the contribution of the dielectric interaction energy density is suggested by Uto[9] to be neglected in the description of the unwinding process generated by an electric field. However, the dielectric contribution into the free energy density is proportional to the squared electric field. Therefore, there seems to be no unified understanding of the interplay between the dielectric contribution and the spontaneous polarization.
The developments shown in the present article are motivated by the earlier results given by Uto.[9] Following the idea, we analyze the equilibrium structure of FLC in the SmC* phase consisting of n pitches. In this study, we show that the effect of the dielectric anisotropy on the free energy can be increased by applying a weak electric field tilted to the smectic layers.
The article is organized as follows. In Section 2, the geometry of the problem and the associated free energy density of FLC in a generally inclined electric field are given. In Section 3, we show the difference in helix unwinding when the electric field is perpendicular and tilted to the smectic layers. The influence of the dielectric interaction energy generated by the electric field is included in the total free energy density. The corresponding energy states for several pitches and tilt angles of the electric field are presented in Section 4. The main results are summarized in Section 5. A more detailed treatment of the computations is given in the appendix.
Consider a slab of thickness d filled with ferroelectric liquid crystal in the SmC* phase, as shown in Fig. 1. The average orientation of the liquid crystal molecules is characterized by a unit vector field known as the director

where φ is the azimuthal angle around the smectic cone angle θ .[3, 10]
 | Fig. 1. Schematic representation of SmC* helical pitches in the electric field E. The director is uniform within each xy cross-section. |
To evaluate the free energy, we adopt a Cartesian coordinate system, where the y axis is along the slab, and the z axis is normal to the plates and the smectic layers. Adopting the elastic part of the free energy density, [11] we have

where

Here the elastic constants K2 and K3 are associated with the twist and bend types of deformations; qt and qb are the twist and bend wavenumbers. In the following, we will treat the problem with one constant approximation, i.e., K2 = K3 = K.
The dielectric interaction energy density[10] is given by

where the relation between the electric displacement D and the generally applied electric field has the form

In the above relation, ε ∥ and ε ⊥ are unitless dielectric constants measured along and normal to the smectic axis. In view of relations (1) and (2), fdiel is given by

where ε a = ε ∥ – ε ⊥ is the dielectric anisotropy, and ε 0 is the dielectric constant.
The interaction of the electric field with the spontaneous polarization of FLC is expressed by

where Ps is the polarization vector. The physical interpretation of Ps can be found in Ref. [10].
In many examples, fdiel is considerably smaller than fp.[3] Therefore, it is common to neglect the dielectric contribution of the free energy. When high electric fields Eu ≳ E are applied, both contributions should be considered. In order to increase the contribution of fdiel in the total free energy density when E ≪ Eu, let the electric field be given by E = E (0, sin α , cos α ), which makes an angle α with the z axis as shown in Fig. 1. Then fp takes the form

where Ps is the magnitude of the polarization vector, and φ is the angle between vectors Ps and E. Then the total free energy f (φ ) of FLC is given by

where V is the volume of the sample. The above formulation of the free energy assumes that the surface effects can be neglected.
If fdiel cannot be neglected in Eq. (3), the free energy density divided by constant B can be represented as

Euler’ s corresponding equation for functional fB can be written as

Since fB does not depend on z explicitly, i.e., fB (φ , φ , z), equation (5) can be reduced to the Beltrami identity

where C1 is an integral constant. The solution of Eq. (6) is represented by the relation

where the ± sign is related with the bistability of FLC, [12] and 
In order to determine the relation between Q0 and the electric field E, we set boundary conditions in such a way that φ is fixed as π or – π on the boundary plates. Then the length of a helical pitch p can be expressed as

where n is the number of pitches between the plates. From the condition φ (0) = 0, it follows that integral constant C2 = 0. Once q0 is fixed, the behavior of Q0 is governed by constant C1 and the applied field. We note that the numerical solution of Eq. (7) does not require an expression for C1.
Our model is simulated for a film of thickness d = 12 μ m with typical parameters for FLC in the SmC* phase: q0 = 2π /(1.4 × 10− 6) rad· m− 1, K = 6.8 × 10− 11 N, θ = 22.5° , and Ps = 5 × 10− 5 C· m− 2.[9, 14]
Figure 2 shows the raise of the modified Q0 with the increase of the electric field E. The induced polarization and dielectric interaction interplay with the applied field, which rotates the directors to align with the field and eventually generate helix unwinding. When the tilt angle α decreases, the spontaneous polarization energy decreases accordingly, and the helix unwinds less readily. Examination of the results reveals that the helix unwinding in FLC can be controlled by changing the tilt angle of the electric field.
To simulate the response of the free energy to the electric field, we must obtain function ψ (z) from Eq. (7). For the following computations, we assume the common parameters of SmC*, i.e., ε a = 1.9, ε ⊥ = 4, and p = 1.4 μ m.[3, 14] Computational details of ψ (z) and the free energy are given in the appendix.
Due to the ± sign in Eq. (7), for both calculated free energies, we choose the absolute minimum. Thus, the free energy changes are computed semi-analytically, and the corresponding curves for various α and n are plotted in Fig. 3. The plots indicate the preferable number of pitches in the film.
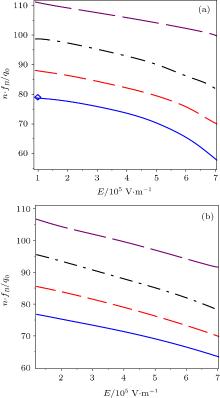 | Fig. 3. The free energy of SmC* as a function of E for n = 6 (solid line), 7 (dashed line), 8 (dash-dotted line), and 9 (long dashed line), with (a) α = π /2 and (b) α = π /100. |
The curves in Fig. 3 give the estimation of the free energy in freely suspended smectic films, and show the control by a single layer change in the thickness. This finding offers a new insight that the bulk properties of the ferroelectric SmC* phase vary in different forms when the field is inclined to the smectic layers.
Smectic films represent special objects for the study of ordered structures in a limited geometry. The helix unwinding process induced by a weak electric field, which makes various angles with the layer of SmC*, is numerically investigated within the continuum theory. Calculations show that the ratio Q0/q0 increases less steeply when the influence of fp is reduced. The differences in helix unwinding can be distinguished by x-ray spectroscopy and optical measurements.
Applying Euler’ s equation for the free energy density, we obtain a relation for the azimuthal angle ψ (z). The substitution of ψ (z) into the free energy density and further computation of the free energy for the system with n helical pitches show its dependence on the electric field (Fig. 3).
Summarizing, we set the relation between a wave number and the electric field when it is tilted to the smectic layers. Then the corresponding energy states of FLC are represented. Applying this model, one can theoretically analyze the contribution of linear and quadratic terms with respect to the operating field E in the relation for the free energy. Based on this analysis, one can select the optimal number of pitches in the film that corresponds to the minimum of the free energy.
| 1 |
|
| 2 |
|
| 3 |
|
| 4 |
|
| 5 |
|
| 6 |
|
| 7 |
|
| 8 |
|
| 9 |
|
| 10 |
|
| 11 |
|
| 12 |
|
| 13 |
|
| 14 |
|
| 15 |
|




