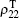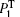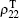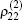†Corresponding author. E-mail: peiliya@ucas.ac.cn
‡Corresponding author. E-mail: pmfu@aphy.iphy.ac.cn
*Project supported by the National Natural Science Foundation of China (Grant Nos. 11404330, 11274376, 61308011, and 11474347), the NSAF, China (Grant No. U1330117), the National Basic Research Program of China (Grant Nos. 2013CB922002 and 2010CB922904), and the China Postdoctoral Science Foundation (Grant No. 119103S239).
We study the relationship between electromagnetically-induced transparency (EIT) and Autler–Townes (AT) splitting in a cascade three-level Doppler-broadened system. By comparing the absorption spectrum with the fluorescence excitation spectrum, it is found that for a Doppler-broadened system, EIT resonance cannot be explained as the result of quantum interference, unlike the case of a homogeneously broadened system. Instead, the macroscopic polarization interference plays an important role in determining the spectra of EIT and AT splitting, which can be explained within the same framework when being detected by the absorption spectra.
To study the important phenomenon of electromagnetically-induced transparency (EIT), [1– 11 people usually use the theoretical model of a three-level system, which is also employed in analysis of Autler– Townes (AT) splitting.[12] Physically, the two effects of EIT and AT splitting are very similar that, dressed states are induced by the so-called coupling or pump field, and then probed through a transition to or from a third level. Furthermore, when a probe field is tuned to the zero-field resonance frequency, both phenomena of EIT and AT splitting can give rise to transparency in an absorption profile.
Recently, the relationship between EIT and AT splitting has been reconsidered by many authors. It is found that depending on the strength of the coupling field, the linear absorption can be expressed either as the difference between two Lorentzian profiles centered at zero frequency with one broad and the other narrow or as the sum of two well-separated Lorentzian profiles with similar widths.[13, 14] Using this theory, Anisimov et al.[15] introduced a method based on Akaikes information criterion to describe the transition from AT splitting to EIT as the pump power decreases. Relevant experimental tests were performed by Giner et al.[16] In this paper we shall study the relationship between EIT and AT splitting in a Doppler-broadened system by comparing the absorption spectrum with the fluorescence excitation spectrum. The motivation for this study is that the absorption spectrum shows a transition from AT splitting to EIT, while the fluorescence excitation spectrum exhibits simply the AT effect. As is well known, quantum interference is a characteristic of a single atom. However, in a Doppler-broadened system there are thousands of atoms with different velocities, so the role of quantum interference in EIT is not clear in this system. It is found that for the Doppler-broadened system the macroscopic effect is more crucial in comparison with the quantum interference. Moreover, it requires to consider the destructive polarization interference between atoms of different velocities.
It is worth mentioning that the problem of EIT in Doppler-broadened systems is not new and has been studied extensively both theoretically and experimentally. For example, Gea-Banacloche et al.[17] discussed the conditions for obtaining EIT with a weak coupling field in a Doppler-broadened cascaded system. Shepherd et al.[18] studied the wave-number-ratio dependence of the EIT resonance. The narrowing of this resonance in a Doppler-broadened medium has been studied by Javan and co-workers.[19, 20] On the experimental side, the subnatural linewidth for probe absorption in an EIT medium has been observed by Iftiquar.[21] On the other hand, fluorescence excitation spectroscopy has been employed widely to study the AT effect. For example, Ahmed and Lyyra[22] analyzed theoretically the AT splitting in a Doppler-broadened three-level cascade system. Relevant experiments have been performed in a three-level Na2 open molecular cascade system, where, for moderate Rabi frequencies, the fluorescence line shape from the uppermost level in the system depends strongly on the wave-number ratio of the two laser fields.[23]
Let us consider a closed cascade three-level system (Fig. 1), where the states between | 0〉 and | 1〉 and between | 1〉 and | 2〉 are coupled by dipolar transitions with resonant frequencies 
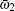

where 

from which we obtain the following equations:

Here Ω i = μ iEi/ħ are the coupling coefficients, Γ ij is the transverse relaxation rate between states | i〉 and | j〉 , and γ 1 (γ 2) is the relaxation rate from | 1〉 to | 0〉 (| 2〉 to | 1〉 ). Here, we have the relation Γ ij = (γ i+ γ j)/2.
 | Fig. 1. Energy-level diagram for EIT resonance and fluorescence excitation spectroscopy in a cascade three-level system. |
In the EIT resonance experiment, the absorption of the probe beam is related to the off-diagonal matrix element ρ 10. On the other hand, in the fluorescence excitation spectroscopy, the intensity of the fluorescence is proportional to the population ρ 22. We first calculate ρ 10 and ρ 20 to the first order in Ω 1 but to all orders in Ω 2. By setting dρ 10/dt = dρ 20/dt = 0 and using the conditions: 


We then calculate ρ 22 to the second order in Ω 1. By setting dρ 21/dt = dρ 12/dt = dρ 11/dt = dρ 22/dt = 0, we obtain

As mentioned in Ref. [22], the polarization P1 = Nμ 1ρ 10 which is responsible for the EIT resonance can be decomposed into linear and nonlinear parts, i.e.,

Here,

The EIT resonance reflects mainly the characteristic of the nonlinear polarization 



Considering a Doppler-broadened system, the total population and polarization are given by

respectively. Here, υ is the atomic velocity, 



Let us consider the case in which the probe and coupling fields are counterpropagating. By setting k1 = − k1z and k2 = k2z, we have 



Here,
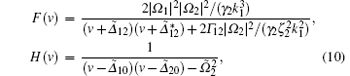
with 




where

Now, let us consider the polarization, which according to Eq. (6) can be expressed as 

with η = Nμ 1/k1 and 

While, for ζ 2 = 1 we have

Let us first consider the case of ζ 2 ≠ 1. We study the pole structure of H(ν ), which appears in Eqs. (9) and (14). By solving the equation

we obtain

where

These poles are related to the resonance with the dressed state.[25] Physically, as a result of the Doppler frequency shift, the atoms with velocities ν ± will be in resonance with the dressed states, where ν ± are the real parts of ṽ ± . When ζ 2 < 1, the velocities ν + and ν − originate from the resonances of the dressed states | + 〉 and | − 〉 , respectively. By contrast, we have both ν + and ν − from the same dressed states when ζ 2 > 1.[25] One consequence is that there is a gap within which no ν ± exists when ζ 2 > 1. Now, we consider the case of ζ 2 = 1. According to Eqs. (11) and (15) the poles of 

Let us now consider the pole structure of the common factor F(ν ) appearing in Eqs. (9) and (11). By solving

we obtain

Here,

This pole corresponds to the case that atoms are in resonance with the transition from | 1〉 to | 2〉 through Doppler frequency shift.
Now, we consider the pole structure of the nonlinear polarization 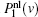


In this section, we shall investigate the absorption and fluorescence excitation spectra in a Doppler-broadened system. For comparison, we first present the spectra in a homogeneously broadened system. Figure 2 presents the imaginary part of the polarization P1 and the population 
Let us now consider a Doppler-broadened system with parameters Γ 10/k1u = 0.02 and Γ 20/Γ 10 = 0.1. Figures 3– 5 present the imaginary part of the polarization 

Let us first consider the case of ζ 2 = 1.2, as shown in Fig. 3. By contrast to the homogeneously broadened case, here both absorption and fluorescence excitation spectra exhibit window when the coupling field is weak (solid curves in Fig. 3). Furthermore, both spectra show similar characteristics of AT splitting. Specifically, corresponding to the case of fluorescence excitation spectra, there are two absorption peaks at the edges of the EIT window. The only difference between them is that the absorption spectrum has a Doppler-broadened background, while the fluorescence excitation spectrum is background-free.
The absorption spectrum behaves quite differently when ζ 2 = 1 (Fig. 4(a)). Although the transparency window is still Doppler-free, no doublet structure appears in the absorption spectrum. On the other hand, the fluorescence excitation spectrum shows a single peak when the coupling field is weak (solid curve in Fig. 4(b)). As the strength of coupling field increases, the spectrum becomes broader. In addition, a window appears at the center of the spectrum. The width of the window increases with the increase of the coupling field strength. Moreover, there is no indication of AT splitting in the fluorescence excitation spectrum.
Finally, let us consider the case of ζ 2 = 0.8 (Fig. 5). In general, both the absorption and fluorescence excitation spectra are similar to those in the homogeneously broadened system. For example, in the weak coupling field regime the absorption spectrum shows a transparency window, which does not appear in the fluorescence excitation spectrum; while in the strong coupling field regime, both spectra exhibit AT splitting. On the other hand, these spectra are no longer Doppler-free. Therefore, to obtain the doublet structure, a much higher coupling field is required.
The wave-number-ration dependence of the absorption and fluorescence excitation spectra can be explained by the dressed-state model. The solid curves in Fig. 6 present the resonant velocities of the transitions from | 0〉 to the dressed states as a function of the frequency detuning Δ 1/Γ 10 with Δ 2/Γ 10 = 0, Ω 2/Γ 10 = 5, and ζ 2 = 1.2, 1, and ζ 3 = 0.8. We also present the resonant velocity of the transition from | 0〉 to the | 1〉 (dashed curves). Let us first consider the case of ζ 2 > 1. According to Eq. (18) there is no solution for ν ± in the regime 

In a Doppler-broadened system, although the total population and polarization are given by the integration over the whole velocity distribution, the main contributions originate from the atoms with resonant velocities, shown as poles of P1(ν ) and 





Let us consider the case of ζ 2 > 1. We first analyze the resonant condition for 


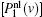





Comparing Figs. 6(a) and 6(b), the main difference between the cases of ζ 2 > 1 and ζ 2 = 1 is that the Δ 1 dependence of the resonant velocity exhibits no gap for the later case. Let us first analyze the absorption spectrum when ζ 2 = 1. As shown in Fig. 8(b), when Δ 1/Γ 10 = 0, no atom in the Doppler profile can be in resonance with the dressed state. On the other hand, due to the pole at ṽ 10, Im

Now, let us consider the fluorescence excitation spectrum. First, 



Finally, the absorption and fluorescence excitation spectra are relatively easy to understand for the case of ζ 2 < 1 (Fig. 5). Since within the Doppler profile there are always atoms of velocities ν = ν + and ν = ν − which are in resonance with the dressed state, as shown in Fig. 6(c), the spectrum exhibits a characteristic of Doppler broadening.
Traditionally, EIT is explained as the result of quantum Fano interference. The requirement for this explanation is based on the fact that when the coupling field is very weak so the AT doublet structure becomes overlapped, a sharp transmission window is still observable. One way to clarify the role of quantum interference is to compare the absorption spectrum with the fluorescence excitation spectrum. For the homogeneously broadened case, both spectra exhibit AT splitting when the strong coupling field is strong. However, when the coupling field is weak the absorption spectrum shows a sharp window, which is absent in the fluorescence excitation spectrum (Fig. 2). Now, let us consider the Doppler-broadened system. Although the absorption spectrum depends strongly on the wave-number ratio ζ 2, the transparency window exists in all cases (Figs. 3(a), 4(a), 5(a)). It may imply that the quantum interference plays a similar role to that in the case of homogeneously broadened system. However, by comparing the fluorescence excitation spectra in the Doppler-broadened system with those in the homogeneously broadened system, it is found that for the former case, instead of quantum interference, here the macroscopic effect plays a more crucial role. Specifically, for the case of ζ 2 > 1 (Fig. 3) both absorption and fluorescence excitation spectra show window when the coupling field is weak. This window is related to the existence of a gap within which no atoms can be in resonance with the dressed states through Doppler frequency shifting (Fig. 6(a)). Similar to the case of ζ 2 > 1, the transparency window in the case of ζ 2 = 1 (Fig. 4(a)) is due to fact that ν d approaches ± ∞ as Δ 1→ 0, so no atoms in the Doppler profile can be in resonance with the dressed state (Fig. 6(b)). On the other hand, no gap exists in this case. As a result, we see no indication of AT splitting in both the absorption and the fluorescence excitation spectra when ζ 2 = 1.
In conclusion, we have studied the relationship between the EIT resonance and the AT splitting in a cascade three-level Doppler-broadened system, and have pointed out the importance of the polarization interference in the Doppler-broadened system. Previously, we have studied resonant stimulated Raman gain and loss spectroscopy in the varLambda-type three-level system and found that in the presence of a coupling field the probe beam can exhibit either gain or loss, depending on the frequencies of the incident beams.[24] In the cascaded three-level system, the gain originates from the stimulated emission due to the scattering of the coupling field by the two-photon coherence. By contrast, the loss is due to the transition from the ground state to the dressed state. In a Doppler-broadened system, gain and loss can exist simultaneously, resulting in destructive polarization interference between atoms of different velocities, as shown in Figs. 7(b) and 8(b). Here, when we use the absorption spectrum instead of the fluorescence excitation spectrum to detect the AT splitting, the two phenomena of EIT and AT splitting can be explained within the same framework.
| 1 |
|
| 2 |
|
| 3 |
|
| 4 |
|
| 5 |
|
| 6 |
|
| 7 |
|
| 8 |
|
| 9 |
|
| 10 |
|
| 11 |
|
| 12 |
|
| 13 |
|
| 14 |
|
| 15 |
|
| 16 |
|
| 17 |
|
| 18 |
|
| 19 |
|
| 20 |
|
| 21 |
|
| 22 |
|
| 23 |
|
| 24 |
|
| 25 |
|