To investigate the formation and isomerization of C cluster, MD simulation is performed for isolated C atoms in He buffer gas. The interaction between C atoms is described by the Brenner potential, and Leonard-Jones potential is applied to the He– He and C– He interactions. N isolated C atoms and 160 He atoms are randomly placed in a cubic with a side length of 40  Å (the pressure of He gas is about 100  atm (1  atm = 1.01325× 10⁵  Pa) at 300  K) and periodical boundary condition is used. Both the C and He atoms are initially set to be at 2500  K, and a thermal bath at the same temperature was applied to the He atoms. In time steps of 0.2  fs, the simulation takes 300  ns. During the evolution, the structure of the C_N system is sampled once every 5  ps and cooled down to 0  K. For a given N, the formation probability of each isomer can be derived by counting the sampling number in running simulations many times.

It is worth noting that the statistic results are taken from simulations taking only 300  ns. To verify whether the simulation time is long enough for the system to arrive at equilibrium, we perform the same MD simulations starting from an isomer, instead of starting from C atomic gas. Such simulations are also run for 300  ns. If such simulations provide the same isomer formation probability distribution as the simulations from C atomic gas, such an isomer formation probability distribution will no longer change considerably even if the simulations are extended to infinite time.

To obtain the reaction path and corresponding rate of transformation between isomers, MD simulations are performed using the technique in Subsection  2.1, starting from a given isomer instead of starting from C atomic gas. The time taken by the transformation from the isomer to another one is recorded. By repeating the simulations a thousand times, probable reaction paths from a given isomer to other isomers are all obtained, and the average reaction rate of each path is derived. Finally, the energy profiles of minimum energy paths are calculated by the nudged elastic band (NEB) method.^{[21, 22]}

In equilibrium, the formation probability of cluster isomer is corresponding to the free energy. In an isothermal-isobaric ensemble, the Gibbs free energies of isomer a and b satisfy

where T is the temperature, and N_a and N_b are the molecule numbers of a and b respectively. Similarly, in an isothermal-isovolumic ensemble the Helmholtz free energies satisfy

When the cluster molecules are treated as an ideal gas, then

and the ratio N_b/N_a in isothermal– isobaric equals that in the isothermal– isovolumic ensemble. Then, by F = − kT lnQ (where Q is the molecular partition function) the ratio reads

In the following discussion we focus on the classical partition function Q and compare it with classical MD simulations.

At low temperature, by the rigid-rotor and harmonic-oscillator approximation Q can be decomposed into

where E₀ is the potential energy (PE) of isomer at 0  K; Q_T, Q_R, and Q_V are the translational, rotational, and vibrational partition functions, respectively. Here,

where M is the molecular mass and V is the volume of simulation box, and

where I_x, I_y, and I_z are the molecular principal moments of inertia, and δ is the rotational symmetry number. The quantum mechanical expression for the vibrational partition function reads

where ν _i is the canonical vibrational frequency of mode i. In the classical limit, it becomes

At high temperature, Q can be calculated numerically. For the atoms located at r₁ ∼ r_n with mass m₁ ∼ m_n and momentum p₁ ∼ p_n, the total energy reads

where U is the interaction potential, and the classical partition function reads

Then, to separate the translational motion new coordinates are employed. According to the translational symmetry the final factor in Eq.  (11) reads

in which the Jacobian

Next, the rotational motion is separated by another transformation stated as follows. Starting from and arbitrary any molecular orientation can be produced by a 3-2-3 Euler rotation. Let us rotate by ζ about the z axis, and by θ about the y axis, and then by ϕ about the z axis. The produced are presented by the rotation matrix

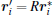
By

and

the integral element in Eq.  (12) reads

Then, according to the rotational symmetry the final factor in Eq.  (12) becomes

Combining Eqs.  (11), (12), and (17), we have

and

in which the term on the right-hand side can be treated as the average value of e^(U/k)(1/T₁ − 1/T₂) in the canonical ensemble at T₁.

Based on the above theory, a technique is developed to calculate the partition function Q of an isomer at a given temperature. At T = 300  K, Q is calculated by Eqs.  (5)– (7), and (9). Then, equation  (19) is employed to accurately calculate Q values from low to high temperature. By the Metropolis Monte Carlo method, the calculation temperature T₂ is increased to 500, 700, 900, … , 2500  K while keeping T₁ = T₂– 200  K, and then the formation probability of each isomer at 2500  K, i.e., the free energy criterion is evaluated by Eq.  (4). Note, for isomers with chirality, Q is taken as the sum of both enantiomers.

In MD simulations for N = 10, the C atoms condense into a C₁₀ cluster in about 0.3  ns. Figure  1(a) shows the evolution of isomer PE sampled in MD, in which the points with the same PE correspond to the same isomer. In most of the time, the C₁₀ system stays in the isomer form with the lowest PE. Sometimes it transforms to a state with higher PE and then falls down immediately. By counting the sampling number of each isomer, the four isomers with the lowest PEs are found to be most probable, denoted as 1, 2, 3, and 4 in Fig.  1(b) with their PEs and symbols of molecular point group. In the MD simulations, the sum of the formation probability of these four isomers is about 99.5%, while other isomers with higher PEs seldom appear. The relative formation probabilities of isomers 1, 2, 3, and 4 are 0.990, 5.53× 10^{− 3}, 1.80× 10^{− 3}, and 2.32× 10^{− 3}. According to free energy calculations and the free energy criterion Eq.  (4), the predicted formation probabilities of isomers 1, 2, 3, and 4 are 0.991, 3.01× 10^{− 3}, 4.57× 10^{− 3}, and 1.60× 10^{− 3}, which are in proximity to the MD values [Fig.  1(c)]. The above results indicate that the C₁₀ system is in thermal equilibrium at 2500  K.

Fig.  1.

	Figure Option ViewDownloadNew Window
	Fig.  1. (a) The PE of C10 isomers sampled during the MD simulation, in which the lowest PE is set to be 0. (b) The four C10 isomers of the lowest PE, with corresponding PEs and symbols of the molecular point group marked. (c) The relative formation probabilities of the isomers 1, 2, 3, 4 in panel  (b) from the MD simulations and free energy calculations.

In MD simulations for N = 20, the C atoms condense into a C₂₀ cluster in less than 0.3  ns, then frequently transform between isomers. In the evolution at T = 2500  K, more than 5000 isomers are found. These isomers can be classified into bowls, sheets, and irregular shapes. By sampling isomer PEs, it is found that the isomerization of C₂₀ is much more frequent than that of C₁₀, and the C₂₀ system seldom stays at the state of the lowest PE [Fig.  2(a)]. The structures of the seven isomers of the lowest PE (denoted as 1– 7) are shown in Fig.  2(b) with their PEs and symbols of molecular point group. By sampling isomers in MD simulations, the relative formation probabilities of the sixteen isomers of the lowest PE (denoted as 1– 16) are derived (shown by the black columns in Fig.  2(c)). The result indicates that the isomer formation probabilities are obviously unrelated to the level of PE. To confirm that the system is in equilibrium, MD simulations are performed by starting from 1, 2, and 3 instead of starting from C atomic gas. By running the simulations many times, the same isomer formation probability spectrum as the simulations from C atomic gas is reproduced. It indicates that such an isomer formation probability spectrum will no longer change even if the simulations are extended to infinite time.

According to free energy calculations and the free energy criterion Eq.  (4), the predicted formation probabilities of isomers 1– 16 (shown by the white columns in Fig.  2(c)) are not in accordance with the MD results. For some isomers, the difference between the formation probability by the free energy criterion and that by MD simulations is even in one order of magnitude. It is worth noting that the cage fullerene (denoted as cage in Fig.  2(b)), which is considered as the smallest fullerene, ^[20] is not found in MD simulations. However, with a free energy of 0.651  eV higher than 1, a considerable formation probability of cage is predicted to be N_cage/N₁ = 4.86× 10^{− 2}. The above facts indicate that the free energy criterion is not suitable for the C₂₀ system at 2500  K. However, the free energies of some sheet isomers (e.g. 4, 6, 10, and 16) are almost lower than those of bowls (e.g. 1, 2, 3, 5, and 7), and the formation probabilities of some sheet isomers are actually higher than those of bowls. Generally speaking, the free energy criterion can only roughly give the formation probabilities of different isomer classes, but fails to quantitatively predict the formation probability.

Fig.  2.

Figure Option ViewDownloadNew Window

Fig.  2. (a) The PE of C20 isomers sampled during the MD simulation, in which the lowest PE is set to be 0. (b) The 7 C20 isomers of the lowest PE (denoted as 1– 7) from the MD simulations and the fullerene (denoted as cage) of C20, with corresponding PE and symbols of molecular point group for symmetric structures marked. (c) The relative formation probability of the 16 C20 isomers of the lowest PE (denoted as 1– 16) from the MD simulations (black column), and the corresponding theoretical values from the free energy calculation (white column). (d) The potential energy profile of the reaction path from isomer 6 to b via an intermediate state a, with corresponding isomer structure shown. (e) The potential energy profile of the reaction path from isomers 1 to 3 via an intermediate state 2, with corresponding isomer structure shown.

Then, the detailed balance between C₂₀ isomers is investigated. As an example, we choose the most probable isomer 6 and perform the simulation introduced in Subsection  2.2, and a most probable reaction path to an isomer b via an intermediate state a is found. By the NEB method, the potential energy profile of 6– a– b along the reaction coordinate is obtained and shown in Fig.  2(d). In MD simulations, we derive a rate k_{6→ a} = 4.79× 10¹⁰  s^{− 1} for the transformation from isomer 6 to isomer a, and k_{a→ 6}= 2.45× 10¹²  s^{− 1} for the transformation from isomer a to isomer 6. However, the ratio N_a/N₆= 3.80× 10^{− 2} of the formation probability of 6 isomer and isomer a in MD is far from k_{6→ a}/k_{a→ 6} = 1.96× 10^{− 2}, which means detailed balance is not reached. Such a situation is also found for the transformation between isomers a and b, for which N_b/N_a = 2.30 is also far from k_{a→ b}/k_{b→ a} = 11.7 (k_{a→ b} = 2.53× 10¹²  s^{− 1}, k_{b→ a} = 2.17× 10¹¹  s^{− 1}). The transformation from isomer 1 to isomer 3 via isomer 2 as an intermediate (corresponding potential energy profile is shown in Fig.  2(e)) is found as another example. It is found that N₂/N₁ = 1.03, k_{1→ 2}/k_{2→ 1} = 0.278, and N₃/N₂ = 0.558, k_{2→ 3}/k_{3→ 2} = 0.807. Such a deviation between the ratio of formation probabilities and isomerization rate further indicates that the free energy criterion is not suitable for the C₂₀ system.

In the evolution at 2500  K, more than 9000 C₃₀ isomers are found, which can be classified into cages, bowls, and sheets. Generally, cages have lower PEs than bowls and sheets, but the C₃₀ system seldom stays at cage states. Figure  3(b) presents the structure of isomer a (which has the lowest PE in all isomers) and the six most probable isomers b– g. By sampling isomers in MD simulations, it can be clearly seen that during the evolution the PE of C₃₀ system is always about 4  eV higher than the lowest value (the upper panel of Fig.  3(a)). The relative formation probability of a– g in MD is shown by the black columns in Fig.  3(c). To confirm that the system is in equilibrium, we also perform MD simulations starting from a instead of starting from C atomic gas, and find that a quickly transformed into sheet isomer with increasing the PE (the lower panel in Fig.  3(a)) in about 2  ns via irregular isomer as an intermediate state. In the following evolution, an isomer formation probability spectrum similar to the previous MD simulations is reproduced. This result indicates that the C₃₀ system is in equilibrium and isomer formation probability spectrum will no longer change even if the simulations are extended to infinite time.

Then, free energy calculations are performed for isomers a– g to examine the free energy criterion Eq.  (4). The predicted formation probabilities are shown by white columns in Fig.  3(c). Although the free energy of isomer a is not the lowest, it is not a stable structure due to some dynamic reason. Comparing the MD simulations with the free energy criterion, it is clearly shown that the free energy criterion fails to quantitatively predict the formation probability. However, its criterion can roughly give the formation probabilities of different types of isomers (cages, bowls, and sheets). For example, the free energies of isomers e, f, and g (sheets) are lower than those of isomers a (cage) and b, c, and d (bowls). And the formation probabilities of isomers e, f, and g are actually higher than those of isomers a, b, c, and d.

Fig.  3.

Figure Option ViewDownloadNew Window

Fig.  3. (a) Upper: the PE of C30 isomers sampled during the MD simulation, lower: the PE of C30 isomers sampled during the MD simulation starting from the isomer of the lowest PE. In both panels the lowest PE is set to be 0. (b) The isomers of C30:a represent the one of the lowest PE and b– g refer to the 6 most probable isomers, with corresponding PE and symbols of molecular point group for symmetric structures marked. (c) The relative formation probabilities of a– g from the MD simulations (black column), and corresponding theoretical values from the free energy calculation (white column).

For C₃₆ system at 2500  K, the isomers can be also classified into cages, bowls, sheets, and irregular shapes. According to the sampling in MD simulations, the PE of the system jumps in a short time [Fig.  4(a)], but for a long time, i.e., the simulation duration 300  ns, the ergodicity is achieved. This point can also be proved by MD simulations starting from a chosen isomer instead of starting from C atomic gas. By any chosen isomers, the same formation probability spectrum as that from C atomic gas is reproduced, indicating that the system is in equilibrium. In the above simulations, the isomer of the lowest PE is isomer 1 with D6h symmetry in Fig.  4(b), i.e., just the one found in experimental preparation.^[23] In Fig.  4(b), the eight most probable C₃₆ isomers are shown, in which isomers 1– 6 are of cages and isomers 7 and 8 are of sheets. The most probable one is isomer 2 with D2d symmetry. According to the sampling in MD simulations, the corresponding formation probability is shown by the black columns in Fig.  4(c).

Fig.  4.

	Figure Option ViewDownloadNew Window
	Fig.  4. (a) The PE of C36 isomers sampled during the MD simulation. (b) Isomers 1– 8 represent the 8 most probable isomers, with corresponding PE and symbols of molecular point group for symmetric structures marked. (c) The relative formation probabilities of isomers 1– 8 from the MD simulations (black column), and corresponding theoretical values from the free energy calculation (white column).

Then, free energy calculations are performed for isomers 1– 8 to examine the free energy criterion Eq.  (4). The predicted formation probabilities are shown by white columns in Fig.  4(c), which are not in agreement with MD simulation results either. At 2500  K, the isomer 1 has the lowest free energy. But in MD simulations it is not the most probable one. In summary, the free energy criterion fails to quantitatively predict the formation probability. However, its criterion can roughly give the formation probabilities of different types of isomers. For example, the free energy of isomer 1 (cage) is lower than those of isomers 7 and 8 (sheets). And the formation probabilities of 7 and 8 are actually higher than that of isomer 1. Overall, for mesoscopic nanosystems the free energy criterion may not provide a good prediction for isomer formation probability. In future research, a new theoretical criterion should be investigated.