As shown in Fig.  2, it is easy to obtain a short second harmonic when the class-F PA is designed and implemented. A quarter wavelength microstrip line was used at the output terminal of the PA. Because of the ground capacity, the second harmonic has an impedance of 0 at the point A; while a quarter wavelength microstrip line means a half wavelength for the second harmonic, so the impedance at the point B is also short for the second harmonic and the circuit behind has no influence on the point B. Hence, a simple quarter wavelength microstrip line provides a DC bias and implements a short second harmonic at the same time. Although the third harmonic does not attract enough attention in the literature, it is exactly the third harmonic that the class-F PA differs from other PAs. The class-F PA needs a short second harmonic and an open third harmonic. In contrast, a normal PA needs all of the harmonics to be short.

Fig.  2.

	Figure Option ViewDownloadNew Window
	Fig.  2. Structure of the class-F PA.

In ordinary research, harmonic impedance is regarded as an independent variable. Different harmonic impedances corresponding to different waveforms have different performance in the class-F PA. However, in the ideal case where the third harmonic is open, the voltage cannot be calculated precisely because of zero current and infinite impedance. We study this problem from another point of view to analyze the influence of the third harmonic on the performance of the class-F PA. Since the third harmonic amplitude is regarded as an independent variable, output power, drain efficiency, and the third harmonic impedance are functions of the third harmonic amplitude. The third harmonic amplitude with optimized performance is exactly determined by a mathematical method and the corresponding impedance is calculated by virtue of Ohm’ s law.

First, we shorten the second harmonic by using a quarter wavelength microstrip line to achieve a second harmonic voltage of 0. The third harmonic voltage is only studied. In order to remove the influence of the DC voltage, all of the harmonic components are normalized to DC, supposing a relative third harmonic voltage amplitude k = V₃/V₁. Therefore, equation  (1) can be rewritten as

only taking the second and third harmonics into consideration. In order to find the maximum value of V_d, we take the derivative of Eq.  (3) and let it be 0, and then the solution is

It can be seen that the solution is a function of k, so the maximum value of V_d(V_d, max) is

Generally, V_dc is half of the break down voltage, so V_{d, max} cannot be greater than the breakdown voltage whose value is 2. Let V_{d, max} be equal to 2, the fundamental voltage V₁ will be a function of k. Figure  3 shows how V₁ varies with k. Obviously, V₁ is 1 without the third harmonic voltage. It can be seen from Fig.  3 that the normalized fundamental voltage amplitude V₁ achieves its maximum value of 1.156 when k is 0.165, while the normalized third harmonic voltage V₃ is 0.19. We can find from Fig.  3 that a suitable out-phase third harmonic voltage contributes to an improvement of the fundamental voltage, thus improving the output power and the drain efficiency.

Fig.  3.

	Figure Option ViewDownloadNew Window
	Fig.  3. Curve between the relative third harmonic voltage k and the fundamental voltage V1.

The relationship between the voltage waveform and k is shown in Fig.  4. It can be seen that as k increases, the voltage waveform is closer to a square wave. However, the waveform will have a serious distortion if the value of k becomes too large.

Fig.  4.

	Figure Option ViewDownloadNew Window
	Fig.  4. Drain voltage waveform at diffident values of k.

As known to all, the drain current of the ideal class-B PA can be written as

where I_q is the normalized quiescent  drain current, and I_max is the normalized maximum radio frequency (RF) current. Since the drain voltage is positive and the input voltage is less than a threshold value, the drain current is also above 0. The drain current almost has the same wave shape as the input voltage, and is not related to the output voltage. However, in practice, when a high-efficiency PA is designed, the I– V knee effect cannot be ignored, so the drain current is not only a function of the input voltage but also a function of the output voltage, which can be corrected by

where V_knee is the voltage at which the drain current reaches 67% of its saturated value, ^[13] and V_d is given by Eq.  (3). The influence of the I– V knee effect on the waveform can be seen in Fig.  5. It can be seen that the current wave will be seriously compressed when V_d is lower than V_knee, so the influence of the I– V knee effect on the PA performance cannot be neglected.

Fig.  5.

	Figure Option ViewDownloadNew Window
	Fig.  5. Influence of the I– V knee effect on a waveform.

According to the Fourier expansion of Eq.  (8), because the current waveform is an even function, the DC and the n-th harmonic component of the drain current can be given by

Since the current and voltage are normalized, the output power of the ideal class-B PA is given by

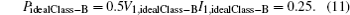
The above equation shows that the fundamental voltage of the ideal class-B V_{1, idealClass− B} is 1, and the fundamental current I_{1, idealClass− B} is 0.5, which are obtained by using the Fourier expansion of I_{d, ideal}. The normalized output power with the I– V knee effect is then given by

The drain efficiency reads

According to Eqs.  (7)– (13), both the drain efficiency and the output power have relativity to V_knee, whose relationship is shown in Fig.  6. The drain efficiency and the output power will decrease as V_knee increases. Suppose that V_knee is less than 1/5 of V_dc, then the drain efficiency of the class-F PA will reduce from 90% to about 70%, while the output power will decrease by 6  dB.

Fig.  6.

	Figure Option ViewDownloadNew Window
	Fig.  6. Influence of the I– V knee effect on the drain efficiency and the output power.

Without the I– V knee effect, the relative third harmonic voltage amplitude k = V₃/V₁ is regarded as an independent variable. According to Eqs.  (4)– (13), the variations of drain efficiency and output power with k are shown in Fig.  7. When k is equal to 0.17, the drain efficiency will achieve its maximum value of 90.77% and the output power will be 0.63  dB higher than that of the ideal class-B PA. In this situation, we can find that the fundamental voltage amplitude equals 1.157, and the third harmonic voltage amplitude equals 0.197 according to Eqs.  (5) and (6). However, the third harmonic current is 0 according to Eq.  (10), and the third harmonic impedance is equal to

We can conclude from the above analysis that the optimized third harmonic impedance of a class-F PA is open without the I– V knee effect.

Fig.  7.

	Figure Option ViewDownloadNew Window
	Fig.  7. Variations of drain efficiency and output power with k without the I– V knee effect.

With the I– V knee effect, according to Eqs.  (4)– (13), the variations of drain efficiency and output power with k for different V_knee are shown in Figs.  8 and 9. As shown in Figs.  8 and 9, for different V_knee, there is one optimized third harmonic voltage which makes the drain efficiency achieve its maximum value with little power compression. On the one hand, both the drain efficiency and the output power decrease with V_knee; while on the other hand, the optimized relative third harmonic voltage k increases and then the optimized third

Fig.  8.

	Figure Option ViewDownloadNew Window
	Fig.  8. Variation of drain efficiency with k for different Vknee.

Fig.  9.

	Figure Option ViewDownloadNew Window
	Fig.  9. Variation of output power with k for different Vknee.

harmonic impedance changes. The influence of V_knee on the performance of a class-F PA is shown in Table  1.

Table 1.

Table 1.

Table 1. Optimized third harmonic impedance for different Vknee. Vknee Optimal k DE/% Pout/dB V3 I3 R3 0 0.17 90.77 0.63 0.193 0 ∞ 0.05 0.26 87.77 − 1.11 0.288 0.062 4.56 0.10 0.29 86.39 − 1.99 0.314 0.070 4.67 0.15 0.30 85.45 − 2.61 0.331 0.071 4.81 0.20 0.32 84.77 − 3.07 0.342 0.069 4.92

Table 1. Optimized third harmonic impedance for different Vknee.

We can see from Table  1 that as V_knee increases, the drain efficiency and output power decrease, the third harmonic voltage fluctuates a little, and the third harmonic current is no longer 0. Correspondingly, the optimized third harmonic impedance is no longer open. The above analysis leads to the following conclusion: the optimized third harmonic impedance is not open with the I– V effect.