Understanding many-body physics in one dimension from the Lieb–Liniger model
Jiang Yu-Zhua), b) , Chen Yang-Yanga), b) , Guan Xi-Wena), b)† 
Understanding many-body physics in one dimension from the Lieb–Liniger model |
|
Jiang Yu-Zhu
|
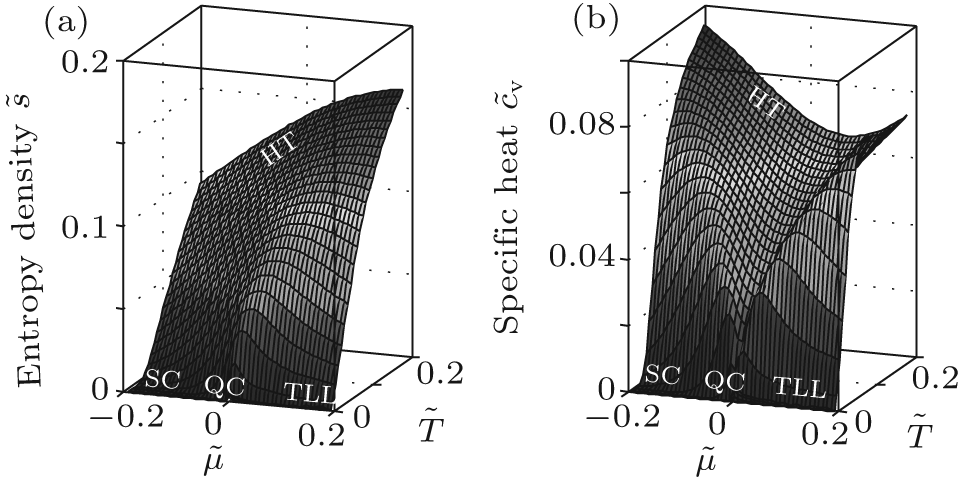
| Quantum critical regimes for the Lieb–Liniger model. (a), (b) Dimensionless entropy and specific heat in the T̃ – μ̃ plane, respectively. For T̃ ≫1, it is the HT regime. The TLL with the dynamical exponent z = 2 and correlation length exponent v = 1 lies in the region T̃ ≪1 and μ > μ c. For T̃ ≫ | μ − μ c|, the QC regime with z = 2, d = 1, and v = 1/2 fans out near the quantum phase transition point μ̃ c = 0. It is obvious that the entropy and the specific heat have singularity properties near the critical point μ c = 0. |
| |