The optomechanical system has attracted much attention because of its potential applications in high precision measurements^[1] and quantum information processing.^[2] In a typical optomechanical system, a movable mirror couples to the cavity field via the radiation pressure, ^{[3, 4]} and this coupling can lead to many remarkable effects, for example, the optomechanically induced transparency (OMIT), ^{[5, 6]} the quantum ground state cooling of the nanomechanical resonators, ^{[7, 8]} entanglement^{[9, 10]} and squeezing.^{[11, 12]}

Entanglement is one of the most striking properties of quantum mechanics and has become a significant resource for quantum information processing. The entanglement beyond the microscopic level has inspired long-standing interest. It is widely accepted that this kind of entanglement should exist if the decoherence induced by the environment can be sufficiently suppressed. So in recent years there has been considerable interest in studying entanglement in mesoscopic and even macroscopic systems, and cavity optomechanical systems have become important candidates for generating quantum entanglement at the macroscopic level.^{[9, 10]} Besides the basic entanglement between the cavity field and the vibrational mirror, ^[9] there are also entanglement between the two vibrational mirrors.^[13]

The optical squeezing is intimately linked to quantum-limited displacement sensing, ^[1] and the optomechanical method of manipulating the quantum fluctuations has been named as the ponderomotive squeezing.^[14] Just like the interaction between electromagnetic radiation and atoms which has led to an interesting quantum feature of squeezing, many are devoted to achieving the ponderomotively squeezed light because of its applications in ultrahigh precision measurements.^{[15– 17]}

The variations beyond the standard optomechanics are abundant, such as a semitransparent membrane within a standard Fabry– Pé rot cavity, ^[18] nanoelectromechanical systems formed by a microwave cavity capacitively coupled to a nanoresonator, ^{[19, 20]} and ultracold atoms interacting with an optical cavity.^{[21– 23]}

In this paper, we study the squeezing spectrum of the transmitted field and the entanglements in a ring cavity with two vibrational mirrors. This system is similar to that proposed in Ref. [13]. In such a system, the two vibration mirrors owning the same or different frequencies will present distinct features. One of the features is double-optomechanically induced transparency.^[24] Here we study the features of the squeezing spectrum and show how the degree of squeezing changes with the external parameters such as the temperature and the input laser power. As the entanglement between the two vibration mirrors has been demonstrated in Ref. [13] using the criterion proposed in Ref. [25], here we mainly study the degree and the robustness of entanglement with respect to the environment temperature.^[9]

The organization of this paper is as follows. In Section 2 we describe the model and present the equations of motion in the frequency domain and in the time domain. We study the ponderomotive squeezing in the frequency domain in Section 3, and the entanglements in the time domain in Section 4, respectively. Section 5 presents the conclusions.

The system we considered is shown in Fig. 1, in which a single mode optical ring cavity consists of two vibrational perfectly reflecting mirrors (M1 and M2) and two fixed mirrors (F1 and F2), one of the fixed mirrors (labeled as F1) is a partially transmitting mirror and the other (labeled as F2) is a perfectly reflecting mirror. The vibrational mirrors M1 and M2 are treated as quantum mechanical oscillators with the same effective mass m, and their frequencies are ω _m1 and ω _m2, respectively. When a classical laser beam with frequency ω ₀ is injected into the cavity through the partially transmitting mirror F1, the Hamiltonian of this system can be described as^[13]

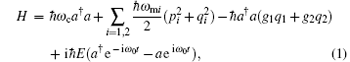
where the first term is the Hamiltonian of the cavity field, whose annihilation and creation operators are a and a^† , respectively, and ω _c is the resonant frequency of the cavity in the absence of the injected laser beam. The second term is the Hamiltonian of the vibrational mirrors with dimensionless position operators q_i and momentum operators p_i (i = 1, 2). The third term describes the nonlinear coupling of the vibrational mirrors to the cavity field via radiation pressure, where is the coupling rate, with β the angle between the incident light and the reflected light at the surfaces of the movable mirrors and L the cavity length in the absence of the intracavity field. The last term describes the interaction of the cavity field with the pump field, with E the amplitude related to the input laser power P by , here κ is the cavity decay rate.

Fig. 1.

	Figure Option ViewDownloadNew Window
	Fig. 1. A ring cavity with four mirrors. A laser beam enters into the ring cavity through the fixed partially transmitting mirror F1. The fixed mirror F2 and the two vibrational mirrors (M1 and M2) are perfectly reflecting.

A proper analysis of the system must include photon losses in the cavity and the Brownian noise acting on the mirrors. This can be accomplished by considering the following set of nonlinear Langevin equations, written in the interaction picture with respect to ω ₀:

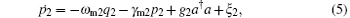
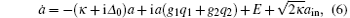
where Δ ₀ = ω _c – ω ₀, and γ _mi (i = 1, 2) is the mechanical damping rate. a_in is the input vacuum noise operator and its nonzero correlation function is^[26]

and ξ is the thermal Langevin force, resulting from the coupling of vibrational mirrors to the environment, with correlation function^[9]

where n_th = [exp(ħ ω _m/k_BT) – 1]^{− 1} is the mean number of thermal excitation with k_B being the Boltzmann constant.

From Eqs. (2)– (6) we can obtain the following steady-state mean-values:

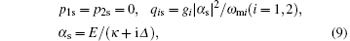
where Δ is the effective cavity detuning given by Δ = Δ ₀ − (g₁q_1s + g₂q_2s).

We can write each Heisenberg operator as a sum of its steady-state mean-value and an additional fluctuation operator with zero mean-value, i.e., a = α _s + δ a, q_i = q_is + δ q_i, p = p_is + δ p_i (i = 1, 2). When we substitute these expressions into Eqs. (2)– (6), and take into account the fact that for generating optomechanical entanglement the input laser power P is usually very large, this means | α _s| ≫ 1, then we can neglect some small quantities and obtain following linearized Langevin equations:

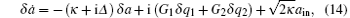
where G_i = g_iα _s(i = 1, 2) is the effective coupling strength.

Sometimes, it is more convenient to work in the frequency domain because fluctuations of the electric field are more convenient to be measured in the frequency domain than in the time domain. For calculating in frequency domain, we introduce the Fourier transform for an operator u (u = δ a, δ p_i, δ q_i, a_in, and ξ _i) as

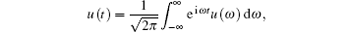
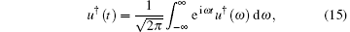
in which [u^† (ω )]^† = u(− ω ). Then we rewrite Eqs. (10)– (14) in the frequency domain, which can be written in matrix form as

where

and

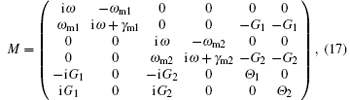
in which we have defined Θ ₁ = κ + i(Δ + ω ) and Θ ₂ = κ + i(− Δ + ω ). By solving Eq. (16), we obtain

where the coefficients are given as follows:

in which we have defined

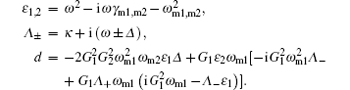
In the following two sections we will study, respectively, the ponderomotive squeezing in the frequency domain, and the entanglements in the time domain.

We first analyze the squeezing properties of the transmitted field, which is accessible to experiment and useful for practical applications.^{[15– 17]} The squeezing spectrum of the transmitted field is given by^[27]

where .^[28] Here, in the input-output relation, the δ a_out is used to describe the output quantum field which is the squeezed light in this system and the a_in is the vacuum noise instead of the input laser. Therefore,

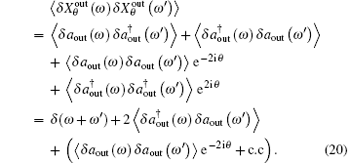
By using the results of Eq. (18) and the following nonzero correlation functions in the frequency domain:^{[9, 26]}

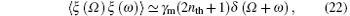
we can further write Eq. (20) to be

in which

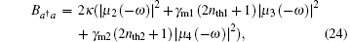
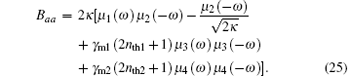
Substituting Eq. (23) into Eq. (19), we can obtain the squeezing spectrum in a simple form

As the phase angle θ is an adjustable parameter, we choose appropriate θ by solving dS_θ (ω )/(dθ ) = 0, then we obtain

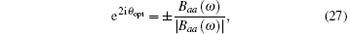
here we choose the minus sign to optimize the degree of squeezing. Then the optimal squeezing spectrum can be written as

In order to obtain squeezing, the term (B_{a^† a} − | B_aa| ) in the right-hand side must be negative, ^[27] i.e., S_opt(ω ) < 1. To illustrate the numerical results, we use the following set of experimentally realizable parameters throughout the paper:^{[29, 30]}λ = 2π c/ω ₀ = 1064 nm, ω _m = 2π × 10⁷ Hz, κ = π × 10⁷ Hz, γ _m1 = γ _m2 = π × 400 Hz, L = 6 mm, m = 12 ng and we set the detuning and the angle as Δ ₀ = 2π × 10⁷ Hz and β = π /3, respectively. Then we show how the squeezing spectrum changes with respect to the external parameters.

Figure 2(a) shows that when the frequencies of the two vibrational mirrors are the same, the squeezing spectrum presents one peak at the frequencies ω = ω _m which is similar to the spectrum of a typical optomechanical cavity. However, once we set the frequencies of the two vibrational mirrors with a difference, the original one peak splits into two peaks at the frequencies ω = ω _m1 and ω _m2 as shown in Fig. 2(b). This frequency difference also leads to a split in the optomechanically induced transparency window, as reported in Ref. [24].

Fig. 2.

	Figure Option ViewDownloadNew Window
	Fig. 2. The squeezing spectrum with (a) ω m1 = ω m2 = ω m; (b) ω m1 = ω m + 0.1 × 2π × 107 Hz, ω m2 = ω m− 0.1 × 2π × 107 Hz. The other parameters are P = 6 mW, T1 = T2 = 3 mK, λ = 2π c/ω 0 = 1064 nm, ω m = 2π × 107 Hz, κ = π × 107 Hz, γ m1 = γ m2 = π × 400 Hz, L = 6 mm, m = 12 ng and we set the detuning and the angle as Δ 0 = 2π × 107 Hz and β = π /3, respectively.

From the expressions of the squeezing spectrum, it is easy to find that the degree of squeezing is related to the mean thermal phonon number n_thi(i = 1, 2), which means that the environment temperature has important effects, and we show how the temperatures change the degree of the squeezing in such a system in Fig. 3. In Fig. 3(a), compared with Fig. 2(b), if we increase the temperature of mirror 1 from 3 mK to 10 mK and keep the temperature of mirror 2 at 3 mK, we find that the degree of squeezing at frequency ω = ω _m1 decreases while there is no effect on the frequency ω = ω _m2. In Fig. 3(b), when we increase the temperature of mirror 1 to 20 mK and still do not change the temperature of mirror 2, the frequency at ω = ω _m1 will present no squeezing. Then we change the temperature of mirror 2 and keep the temperature of mirror 1 at 20 mK, and show the squeezing spectrum in Figs. 3(c) and 3(d). In this case, only the degree of squeezing at frequency ω = ω _m2 changes. We can discuss the above phenomena physically as follows. As the noise reduction is a result of mechanical motion, ^[27] there is no squeezing when the coupling g_i(i = 1, 2) is zero. Once the squeezing emerges, it occurs at the frequency corresponding to the mechanical frequency which we have shown in Figs. 2 and 3. In other words, each vibrational mirror has a direct effect on the degree of squeezing related to its own frequency.

Fig. 3.

	Figure Option ViewDownloadNew Window
	Fig. 3. The squeezing spectrum with (a) T1 = 10 mK, T2 = 3 mK; (b) T1 = 20 mK, T2 = 3 mK; (c) T1 = 20 mK, T2 = 10 mK; (d) T1 = 20 mK, T2 = 20 mK. The other parameters are P = 6 mW, λ = 2π c/ω 0 = 1064 nm, ω m = 2π × 107 Hz, κ = π × 107 Hz, γ m1 = γ m2 = π × 400 Hz, L = 6 mm, m = 12 ng and we set the detuning and the angle are Δ 0 = 2π × 107 Hz and β = π /3, respectively.

At last, we show the effects of the power of laser on the degree of squeezing in Fig. 4. To clearly see these effects, we set the temperature of mirrors at T₁ = T₂ = 15 mK. In Figs. 4(a) and 4(b), we can find that as we increase the power of the laser, the degree of squeezing at both frequencies is enhanced. This is because the laser interacts with the two vibrational mirrors at the same time and a larger power will input more photons, which will effectively overcome the effects of thermal noise.

Fig. 4.

	Figure Option ViewDownloadNew Window
	Fig. 4. The squeezing spectrum with (a) P = 8 mW; (b) P = 20 mW. The other parameters are T1 = T2 = 15 mK, λ = 2π c/ω 0 = 1064 nm, ω m = 2π × 107 Hz, κ = π × 107 Hz, γ m1 = γ m2 = π × 400 Hz, L = 6 mm, m = 12 ng and we set the detuning and the angle as Δ 0 = 2π × 107 Hz and β = π /3, respectively.

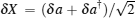
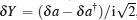
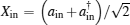
In this section we discuss the entanglement between one of the vibrational mirrors and the cavity field (the mirror– cavity entanglement), and the entanglement between the two vibrational mirrors (the mirror– mirror entanglement). We define the cavity field quadratures and , and the corresponding Hermitian input noise operators and , then equations (10)– (14) can be rewritten as the matrix form

in which the transposes of the column vectors f(t) and χ (t) can be expressed as

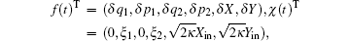
respectively, and the matrix A is

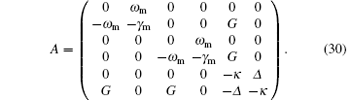
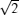
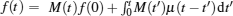
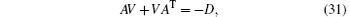
Here, we have introduced the effective optomechanical coupling G = gα _s, and assumed ω _m1 = ω _m2 = ω _m, and γ _m1 = γ _m2 = γ _m for simplicity. The solution of Eq. (29) is , here M(t) = e^At. The system reaches its steady state as t → ∞ only if the real parts of all the eigenvalues of matrix A are negative so that M(∞ ) = 0. The stability conditions for the system can be found by employing the Routh– Hurwitz criterion.^[31] When the system is stable, it reaches a unique steady state. Owing to the quantum noises, ξ and a_in are zero-mean quantum Gaussian noises and the dynamics is linearized, thus the quantum steady states for the fluctuations are a zero-mean tripartite Gaussian state, which is completely determined by the 6 × 6 correlation matrix V_ij = 〈 f_i(∞ )f_j(∞ ) + f_j(∞ )f_i(∞ )〉 /2, here f^T(∞ ) = (δ q₁(∞ ), δ q₂(∞ ), δ p₁(∞ ), δ p₂(∞ ), δ X(∞ ), δ Y(∞ )) is the vector of continuous variables fluctuations operators at the steady state (t → ∞ ). When the stability conditions are satisfied, the steady-state correlation matrix (CM) satisfies a Lyapunov equation

where D = Diag[0, γ _m(2n̄ _th + 1), 0, γ _m(2n̄ _th + 1), κ , κ ] is a diagonal matrix and n̄ _th is the mean thermal excitation number.

In order to investigate the behavior of the continuous variables (CV) entanglement between the elements of the tripartite system, we consider the logarithmic negativity E_mc, a quantity which has already been proposed as a measure of entanglement, ^[32] to measure the entanglement between the cavity field and one of the mirrors (here we choose the left mirror of this system). We define^[33]

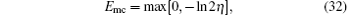
where η = 2^{− 1/2}{Σ (Γ ) − [Σ (Γ )² − 4detΓ ]^1/2}^1/2 with Σ (Γ ) = detL_m + detL_f − 2detL_mf, and we have used the 2 × 2 block form of the correlation matrix

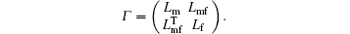
Here, Γ is obtained by tracing out the mirror– mirror mode, i.e., removing the rows and columns of V corresponding to mirror– mirror. L_m, L_f, and L_mf are 2× 2 matrices, where L_m corresponds to the mechanical mode, L_f refer to the field mode and L_mf describes the correlation between these two modes. Similarly, we can quantify the bipartite entanglement of the mirror– mirror subsystem. To illustrate the numerical results, we choose the realistic parameters of the entanglement systems to be the same as in Section 3.

Fig. 5.

Figure Option ViewDownloadNew Window

Fig. 5. (a) Plot of the logarithmic negativity Emc versus the environment temperature. The red-dot-dashed, solid, blue-dashed curves refer to P = 1 mW, 5 mW, 10 mW, respectively. (b) Plot of the logarithmic negativity Emm versus the environment temperature. The red-dot-dashed, solid, blue-dashed curves refer to P = 6 mW, 8 mW, 10 mW, respectively. The other parameters are λ = 2π c/ω 0 = 1064 nm, ω m = 2π × 107 Hz, κ = π × 107 Hz, γ m1 = γ m2 = π × 400 Hz, L = 6 mm, m = 12 ng and we set the detuning and the angle are Δ 0 = 2π × 107 Hz, and β = π /3, respectively.

In Fig. 5(a) we plot the mirror– cavity entanglement E_mc with respect to the environment temperature T. It can be seen that (i) E_mc decreases with the increase of T; (ii) E_mc increases with the increase of the laser power P; (iii) E_mc disappears at almost the same temperature (about 0.4 mK) for different laser powers. The third point is an interesting phenomenon, which means there is nearly the same critical temperature for different powers. This is because when the temperature reaches the critical temperature, the effect of thermal noise on E_mc is greater than that of increasing the laser power.

The mirror– mirror entanglement E_mm as a function of the environment temperature is shown in Fig. 5(b). It can be seen that E_mm has the features that are similar to the first two points of E_mc. However, the robustness of E_mm against T can be remarkably improved when we increase the laser power, this is very different from E_mc. That is to say, for the mirror– mirror entanglement E_mm, when the laser power is larger than 8 mW, it can effectively overcome the effect of thermal noise compared to Fig. 5(a).

In conclusion, we have studied the properties of the ponderomotive squeezing and the entanglements in a ring cavity with two vibrational mirrors. We have found that we can obtain a two-mode squeezed light in this system and can control the degree of squeezing flexibly by adjusting the related temperature or the laser power. We have also shown that the robustness of the mirror– mirror entanglement E_mm with respect to the temperature is enhanced obviously when we increase the laser power. However, the mirror– cavity entanglement E_mc has no such feature.