†Corresponding author. E-mail: clyuan@jxnu.edu.cn
*Project supported by the National Natural Science Foundation of China (Grant Nos. 11164008, 51461019, 51361013, 11174226, and 51371129).
The strain distributions of Au/Ag and Ag/Au nanoparticles confined in the Al2O3 matrix with different core sizes are investigated by using the finite element method, respectively. The simulation results clearly indicate that the compressive strains exerted on the Au/Ag and Ag/Au nanoparticles can be induced by the Al2O3 matrix. Moreover, it can be found that the strain gradient existing in a Au/Ag nanoparticle is much larger than that in a Ag/Au nanoparticle, which could be due to the larger Young’s modulus of Au than that of Ag. With the core size increasing, the strain gradient existing in the Au/Ag nanoparticle becomes larger, while the strain gradient existing in the Ag/Au nanoparticle keeps constant. These different strain distributions may have significant influences on the structures and morphologies of the Au/Ag and Ag/Au nanoparticles, leading to the different physical properties for potential applications.
The strain phenomenon in nanoscale materials is considerably different from those in their conventional bulk counterparts. These “ unusual” phenomena not only allow these materials to possess excellent mechanical properties but also enable them to tune their band structures and relevant novel electronic, magnetic, optical, photonic, and catalytic properties.[1– 4] The core/shell nanoparticles have received much attention because of their special and multiple properties in physics and chemistry, and they have been used intensively in many different fields of research and technology.[5, 6] Compared with the corresponding monometallic particles, gold (Au)/silver (Ag) and Ag/Au nanoparticles with a core/shell structure have been used intensively in many different fields of research and technology including catalysis, nanophotonics, nanomedicine, and nonlinear devices because of their interesting properties.[7, 8] Many research efforts have been devoted to the preparation and investigation of Au/Ag and Ag/Au core/shell nanoparticles due to their unique size-dependent physical, chemical, and optical properties.[8– 10] For example, the optical properties of these core/shell nanoparticles can be tuned by changing the size of the core and the thickness of the shell. It has been demonstrated that the peak resonance of core/shell nanoparticles can be adjusted over a broad range of the optical spectrum by varying the relative core size and shell.[8– 11] However, there have been too few studies on the interplay between the core and shell layers, especially their dynamical behavior and mechanical properties.
Moreover, the noble metal nanoparticles confined in a solid matrix have received much attention because of their special and multiple properties in physics and chemistry.[12– 14] However, it has been illustrated that substantial strain can be induced for the nanoparticles confined in a host matrix.[15– 17] The strain may be relaxed through the mismatched process of nanoparticles and subsequently modify the interplay between the core and shell layers, which plays an important role in determining the physical properties of the nanoparticles. For the core/shell nanoparticles confined in the solid matrix, the interface between the core and shell layer is particularly important because the substantial strain field can lead to extra strain-relaxing defects at the heterostructure interface, which can tune the optical properties of core/shell nanoparticles significantly.[18– 20] However, systematic study on the strain distribution of the confined Au/Ag and Ag/Au nanoparticles is lacking. Therefore, there is strong motivation to investigate the strain distributions of the confined Au/Ag and Ag/Au nanoparticles for their better applications.
In this article, the strain distributions of core/shell nanoparticles composed of Au and Ag with different core sizes and confined in the Al2O3 matrix are investigated by the finite element (FE) calculations. The simulation results clearly indicate that the compressive strain exerted on the Au/Ag and Ag/Au nanoparticles can be induced by the Al2O3 matrix. The strain existing in the core is homogenous, in contrast, the strain existing in the shell is inhomogeneous. With the increase of the core size, the strain gradient existing in the Au/Ag nanoparticle becomes larger, while the strain gradient existing in the Ag/Au nanoparticle keeps constant. Moreover, for the Au/Ag nanoparticles, the strain existing in the core is smaller than that at the interface between core and shell, while for the Ag/Au nanoparticles, the strain existing in the core is larger than that at the interface between core and shell. These different strain distributions of core and shell can lead to different physical properties, which may have significant influences on the technological potential applications of the Au/Ag and Ag/Au nanoparticles, respectively.
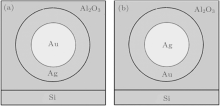
A significant strain can be introduced when the nanomaterials are confined in a matrix, which is most likely to account for the changes in the microstructure and morphology of nonomaterial.[21, 22] In order to investigate the interplays between the strain and the morphology of confined Au/Ag and Ag/Au nanoparticles, an FE simulation is performed to simulate the dynamic strain distributions of confined Au/Ag and Ag/Au core/shell nanoparticles with different core sizes. The FE calculation is a versatile computer simulation technique used for continuum modeling of deformation, [23] which is performed with a commercial software package ANSYS.[24, 25] The simulations are taken to account for the physical properties of many materials, including elastic anisotropy, thermal expansion, and three-dimensional object shape, etc. Moreover, general qualitative agreement between atomistic strain calculations and continuum elastic models has been demonstrated in nanomaterials.[26, 27] Recently, using the FE calculation to simulate the strain distribution of nanoparticles has been widely reported.[28, 29] In our simulation, the FE model for the strain calculations is based on the following assumptions: a spherical, linear-elastic Au/Ag and Ag/Au core/shell nanoparticle is confined in the isotropic and the linear-elastic matrix, repectively. The whole region of the model is divided into a mesh of triangle-shaped elements. These triangles are next to each other, where the adjacent elements share an edge. Figures 1(a) and 1(b) show the cross-sectional views of the core/shell structures of Au/Ag and Ag/Au nanoparticles embedded in the Al2O3 matrix, respectively. The thickness values of the shells are both 2.5 nm and the core sizes are 2.5 nm, 5 nm, 7.5 nm, and 10 nm for each nanoparticle. The Au/Ag or Ag/Au nanoparticle surface is welded to the matrix. Supposing that the nanoparticle that resides in the matrix cavity is too small, this volumetric difference may be due to the fact that matrix atoms are unable to move promptly enough to adapt to the growing nanoparticle, which leads to compressive strain on the nanoparticle. The strain distribution can be generated by the thermal expansion mismatch by the confined Au/Ag or Ag/Au nanoparticles in an Al2O3 matrix. The Young’ s moduli are taken to be 170 GPa, 76 GPa, and 360 GPa for Au, Ag, and Al2O3, while the Poisson’ s ratios are taken to be 0.42, 0.38, and 0.24 for Au, Ag, and Al2O3, respectively.
Figures 2(a)– 2(d) show the cross-sectional strain distributions for Au/Ag nanoparticles confined in Al2O3 thin film with the core sizes of 2.5 nm, 5 nm, 7.5 nm, and 10 nm, respectively. Correspondingly, figures 2(e)– 2(h) show the x– y plane strain profiles of confined Au/Ag nanoparticles with core sizes of 2.5 nm, 5 nm, 7.5 nm, and 10 nm, respectively. Figures 2(a)– 2(h) indicate that a compressive strain exerted on the Au/Ag nanoparticle can be induced by the Al2O3 matrix. The strain existing in the core is homogenous; in contrast, the strain existing in the shell is inhomogeneous. Further, the strain existing in the core is smaller than that at the interface between core and shell. Obviously, all the strains of the Au/Ag nanoparticles become larger with the increase of the core size.
Figures 3(a)– 3(d) show the cross-sectional strain distributions for Ag/Au nanoparticles confined in Al2O3 thin films with the core sizes of 2.5 nm, 5 nm, 7.5 nm, and 10 nm, respectively. Correspondingly, figures 3(e)– 3(h) show the x– y plane strain profiles of confined Ag/Au nanoparticles with the core sizes of 2.5 nm, 5 nm, 7.5 nm, and 10 nm, respectively. Figures 3(a)– 3(h) indicate that a compressive strain exerted on the Ag/Au nanoparticles can be also induced by the Al2O3 matrix. The strain existing in the core is homogenous; in contrast, the strain existing in the shell is inhomogeneous. However, the strain decreases monotonically from the core to the shell, which is quite different from the strain distribution of the Au/Ag nanoparticles. Obviously, the whole strain of the Ag/Au nanoparticles also becomes larger with the increase of the core size.
Figure 4 shows the strain distributions in the core and at the interface between Au/Ag and Ag/Au nanoparticles for the same thermal expansion coefficient with the core sizes being 2.5 nm, 5 nm, 7.5 nm, and 10 nm, respectively. Obviously, the strains in Au/Ag and Ag/Au nanoparticles become larger with the increase of the core size. For the Au/Ag nanoparticle, it can be found that the strain existing in the core is smaller than that at the interface between the core and shell, and the strain gradient increases with the increase of the Au core size. However, for the Ag/Au nanoparticle, the strain existing in the core is larger than that at the interface, and the strain gradient keeps almost constant when the Ag core size increases. Moreover, it should also be noticed that the strain in the core of the Au/Ag nanoparticle is smaller than the strains at the interface and in the core of the Ag/Au nanoparticle. Meanwhile, the strain at the interface of the Au/Ag nanoparticle is larger than the strains at the interface and in the core of the Ag/Au nanoparticle. This phenomenon can be attributed to the fact that the Young modulus of Au is larger than that of Ag. The strain distribution can be generated by the thermal expansion mismatch of the confined Au/Ag or Ag/Au nanoparticles. The strained state of the nanoparticle results from a gradual volume contraction. Because of the thermal expansion mismatch, the Al2O3 matrix exerts a compressive strain on the Au/Ag or Ag/Au nanoparticles. At the same time, the matrix is also compressively strained in the radial direction surrounding the Au/Ag or Ag/Au nanoparticles because of the volume expansion of the Au/Ag or Ag/Au nanoparticles. Since the Young modulus of Au is larger than that of Ag, the strain gradient existing in the Au/Ag nanoparticle is much larger than that in the Ag/Au nanoparticle. The different strain distributions of Au/Ag and Ag/Au nanoparticles confined in the matrix may have significant influences on the structures and morphologies of Au/Ag and Ag/Au nanoparticles, and thus influence the physical properties of Au/Ag and Ag/Au nanoparticles.
The strain distributions of Au/Ag and Ag/Au nanoparticles confined in the Al2O3 matrix with different core sizes and the same shell thickness are systematically investigated by using the FE method, respectively. Since the Young modulus of Au is larger than that of Ag, the strain gradient existing in the Au/Ag nanoparticle is much larger than that in the Ag/Au nanoparticle. With the increase of the core size, the strain gradient existing in the Au/Ag nanoparticle becomes larger, while the strain gradient existing in the Ag/Au nanoparticle keeps constant. These different strain distributions may have significant influences on the structures and morphologies of the Au/Ag and Ag/Au nanoparticles, leading to the different physical properties for potential applications.
| 1 |
|
| 2 |
|
| 3 |
|
| 4 |
|
| 5 |
|
| 6 |
|
| 7 |
|
| 8 |
|
| 9 |
|
| 10 |
|
| 11 |
|
| 12 |
|
| 13 |
|
| 14 |
|
| 15 |
|
| 16 |
|
| 17 |
|
| 18 |
|
| 19 |
|
| 20 |
|
| 21 |
|
| 22 |
|
| 23 |
|
| 24 |
|
| 25 |
|
| 26 |
|
| 27 |
|
| 28 |
|
| 29 |
|