†Corresponding author. E-mail: zhcheng@iphy.ac.cn
*Project supported by the National Basic Research Program of China (Grant Nos. 2010CB934202, 2011CB921801, and 2012CB933102) and the National Natural Science Foundation of China (Grant Nos. 11174351, 11274360, and 11034004).
We have investigated the anisotropic magnetocaloric effect and the rotating field magnetic entropy in DyFeO3 single crystal. A giant rotating field entropy change of
Recently, the magnetic refrigeration based on magnetocaloric effect (MCE) that uses an external magnetic field to change the magnetic entropy of magnetic materials, has attracted much attention due to its higher energy efficiency and lower environmental influence than the conventional gas compression refrigeration.[1– 3] Although new materials with giant MCE near room temperature have been studied for applications, materials with giant MCE in the low-temperature region from about 30 K down to sub-Kelvin temperatures are also essential for utilization in certain fields, such as gas liquefaction.[1] Helium plays a significant role in low-temperature scientific research and cryogenic engineering.[4] Generally, helium gas needs to be cooled down to liquid form for its storage and transportation. Therefore, it is significant to develop advanced magnetic refrigerants with large MCE around the liquid helium temperature (4.2 K). In addition, most of the permanent magnets in present market can only provide a maximum field about ∼ 20 kOe. This fact indicates that a large MCE under low magnetic field change is desirable for the fulfillment of a magnetic refrigerator. Low magnetic field induced giant magnetic entropy change has been observed in some rare-earth based intermetallic compounds, such as ErMn2Si2, [5]ErCr2Si2, [6] ErRu2Si2, [7]HoCuSi, [8] ErFeSi, [9] and TmGa, [10] whereas it has not been observed in rare-earth oxides. Alternatively, magnetic refrigeration can also be achieved by a rotating field MCE based on changing the magnetic anisotropy energy in a constant magnetic field, [11] which is attractive due to the simplification and possible miniaturization of the device.[12] Recently, rotating field entropy change due to magnetocrystalline anisotropy has been observed in TbMnO3, TmMnO3, ErFeO3, HoMn2O5, and PrSi at low temperatures.[13– 17] The exploration of magnetic refrigerant materials with large anisotropic MCE under low magnetic field has practical application value.
In the present work, we report a detailed study on the low-field MCE of DyFeO3 single crystal around liquid helium temperature. The reason for choosing DyFeO3 single crystal is three-fold. Firstly, DyFeO3 shows a complex magnetic transformation. In this system, iron ions order in a weakly canted antiferromagnetic structure below the Né el temperature TN= 645 K, and exhibit characteristic spin-reorientation (Morin) transitions Γ 4(Gx, Ay, Fz) → Γ 1(Ax, Gy, Cz), [18– 21] at TM∼ 50 K upon cooling, [22] which is only observed in DyFeO3 within RFeO3 (where R is rare-earth elements) series.[21] The Dy3 + sublattice remains paramagnetic until the temperature reaches its ordering temperature 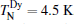
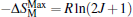
DyFeO3 ceramic was prepared with the starting material Dy2O3 (> 99.9%)and Fe2O3 (> 99.9%) by using the solid-state reaction method, and was pressed into pellets and sintered in air atmosphere for 48 hours at 1300 ° C. The crystal structure of the sample was examined by powder X-ray diffraction. Seed rods for crystal growth were prepared under the hydrostatic pressure and sintered at 1400 ° C for 48 hours in air atmosphere. DyFeO3 single crystal was grown with four ellipsoidal mirrors (Crystal Systems Inc, FZ-T-10000-H-VI-VP) by the floating zone method. Back-reflection Laue X-ray diffraction experiments were carried out to check the single crystallinity and determine the crystallographic direction. Measurements of magnetization as functions of temperature and magnetic field were performed on commercial superconducting quantum interference device (SQUID) magnetometer (quantum design MPMS-XL).
XRD patterns and back-reflection Laue XRD patterns (not shown) confirmed that DyFeO3 single crystal has an orthorhombically distorted pervoskite structure with Pbnm symmetry, which presented a high single crystallinity of the studied crystals. The Fe magnetic sublattices undergo a paramagnetic-antiferromagnetic transition around 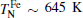
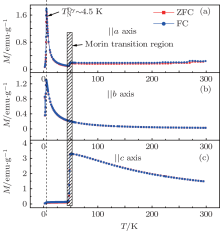 | Fig. 1. Zero-field-cooled (ZFC) and field-cooled (FC) thermal magnetization curves of DyFeO3 along the (a) a, (b) b, and (c) c axes from 2 K to 300 K under a magnetic field of 100 Oe. |
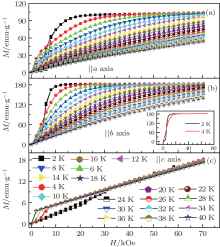
The isothermal magnetization curves as functions of field along different crystal directions were measured to investigate the anisotropic magnetic entropy change in DyFeO3 single crystal. Figures 2(a)– 2(c) illustrate the isothermal magnetization curves along the a, b, and c axes of the DyFeO3 single crystal in the temperature range of 2– 40 K with an interval of 2 K, respectively. The isothermal magnetization curves along the a and c axes of the DyFeO3 single crystal in the Fe3 + moments spin-reorientation temperature range of 40– 70 K are also measured (not shown). There is no obvious increase or peak in the curves. This is because the magnetization change in the Morin transition region is quite small since the magnitude of the WFM component is about 0.01 percent of the G-type component in an Fe sublattice. The magnetization curves for these three directions are different either in shape or in magnetization values. A spin-flip phenomenon can be observed along the a, b, and c axes of the DyFeO3 single crystal at 2 K due to the antiferromagnetic interaction of R– R being similar to TbFeO3.[27– 29] A complete reversibility of MCE requires that no hysteresis of either the temperature or the magnetic field appears in the vicinity of a magnetic ordering temperature. As shown in the inset of Fig. 2(b), a negligible hysteresis loop was observed only at 2 K and 4 K for the b axis. When the temperature is above 4 K, no hysteresis loops can be observed from the isothermal magnetization curves. From the data we can see a significant difference in the isothermal magnetization curves along the a, b, and c axes of the DyFeO3 single crystal, especially between the b axis and the c axis at low field, which implies that a giant anisotropy of MCE can be expected in the bc plane. Magnetic field-induced entropy change -Δ SM was calculated from the isothermal curves with the following equation based on a Maxwell relation:
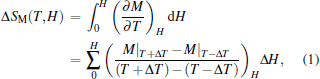
where the slope of two adjacent data points is approximatively used for the numerical calculation of the gradient of (∂ M/∂ T)H. In this case, Δ T = 1 K and Δ H = 2 kOe.
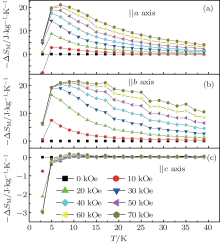
The calculated -Δ SM as a function of temperature is shown in Figs. 3(a)– 3(c) for a field along the a, b, and c axes, respectively. Peaks appear at 9 K for the a axis with a value of 21.10 J/kg· K and at 11 K for the b axis with a value of 21.48 J/kg· K in a field of 70 kOe, whereas the values of -Δ SM for the c axis are smaller than those of the other two axes in the temperature range above the ordering of Dy3 + moments 
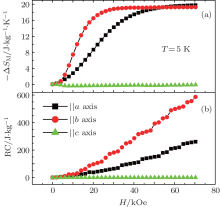 | Fig. 4. Calculated -Δ SM and refrigeration capacity (RC). Field dependence of (a) magnetic entropy changes of DyFeO3 and (b) refrigeration capacity along the a, b, and c axes at 5 K. |
The field-dependent -Δ SM of different axes of DyFeO3 single crystal at 5 K is shown in Fig. 4(a). A large anisotropy of MCE is observed in DyFeO3 single crystal among the ab plane and c axis. It is noteworthy that there is a giant change below 20 kOe along the b axis at 5 K. For magnetic refrigeration application, not only a large entropy change value, but also a large refrigeration capacity is needed. The characteristic parameter that determines the magnetic cooling efficiency of a magnetocaloric material is the refrigeration capacity (RC) and is defined as
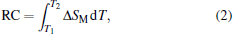
where T1 and T2 are the temperatures corresponding to both sides of the half-maximum value of -Δ SM peak. The RC is the measurement of the amount of heat transfer between the cold and hot reservoirs in an ideal refrigerator as a function of field. The field-dependent refrigeration capacity of different crystallines of the DyFeO3 single crystal is shown in Fig. 4(b). The three directions of DyFeO3 single crystal manifest obvious anisotropy with RC value, which is equal to 260.48 J/kg, 586.08 J/kg, and 10.1 J/kg in a field of 70 kOe for a, b, and c axes, respectively. It is interesting that DyFeO3 single crystal exhibits a strong magnetocrystalline anisotropy between b and c axes. Single crystal with giant anisotropic MCE at a relatively small magnetic field is a promising choice for the new type of magnetic refrigeration by simply rotating the magnetic field or refrigerants.
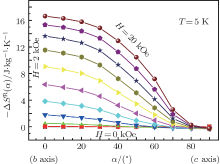
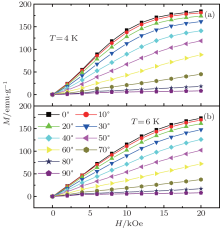 | Fig. 5. Isothermal magnetization curves within the bc plane of DyFeO3 single crystal at (a) 4 K and (b) 6 K. 0° and 90° correspond to the direction of b and c axes, respectively. |
The rotating magnetic entropy change 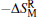

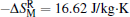
In conclusion, a large anisotropic entropy change and RC were observed in DyFeO3 single crystal. Owing to the strong magnetocrystalline anisotropy between b and c axes, there was a giant rotating magnetic entropy change 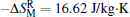
| 1 |
|
| 2 |
|
| 3 |
|
| 4 |
|
| 5 |
|
| 6 |
|
| 7 |
|
| 8 |
|
| 9 |
|
| 10 |
|
| 11 |
|
| 12 |
|
| 13 |
|
| 14 |
|
| 15 |
|
| 16 |
|
| 17 |
|
| 18 |
|
| 19 |
|
| 20 |
|
| 21 |
|
| 22 |
|
| 23 |
|
| 24 |
|
| 25 |
|
| 26 |
|
| 27 |
|
| 28 |
|
| 29 |
|