Cite this Article
Yang Li-Jun†, Cao Jun-Peng, Yang Wen-Li. Exact solution of Heisenberg model with site-dependent exchange couplings and Dzyloshinsky–Moriya interaction. Chinese Physics B, 24(10): 107502  
Permissions
Exact solution of Heisenberg model with site-dependent exchange couplings and Dzyloshinsky–Moriya interaction
Yang Li-Jun†a), Cao Jun-Penga),b), Yang Wen-Lic),d)
Beijing National Laboratory for Condensed Matter Physics, Institute of Physics, Chinese Academy of Sciences, Beijing 100190, China
Collaborative Innovation Center of Quantum Matter, Beijing, China
Institute of Modern Physics, Northwest University, Xian 710069, China
Beijing Center for Mathematics and Information Interdisciplinary Sciences, Beijing 100048, China

†Corresponding author. E-mail: chortley@iphy.ac.cn

*Project supported by the National Natural Science Foundation of China (Grant Nos. 11174335, 11375141, 11374334, and 11434013) and the National Program for Basic Research of China and the Fund from the Chinese Academy of Sciences.

Abstract

We propose an integrable spin-1/2 Heisenberg model where the exchange couplings and Dzyloshinky–Moriya interactions are dependent on the sites. By employing the quantum inverse scattering method, we obtain the eigenvalues and the Bethe ansatz equation of the system with the periodic boundary condition. Furthermore, we obtain the exact solution and study the boundary effect of the system with the anti-periodic boundary condition via the off-diagonal Bethe ansatz. The operator identities of the transfer matrix at the inhomogeneous points are proved at the operator level. We construct the T– Q relation based on them. From which, we obtain the energy spectrum of the system. The corresponding eigenstates are also constructed. We find an interesting coherence state that is induced by the topological boundary.

PACS: 75.10.Pq; 03.65.Vf; 71.10.Pm
Keyword: Bethe ansatz; Yang–Baxter equation; T– Q relation
1. Introduction

Quantum integrable systems have attracted considerable interest because they can provide an exact insight into one-dimensional strongly correlated systems. Based on Yang’ s[1, 2] and Baxter’ s[3, 4] pioneering works, the Leningrad group proposed the quantum inverse scattering method (QISM) or the algebraic Bethe ansatz method[5– 9] for integrable systems with the periodic boundary condition (PBC). Later, this method was generalized to the open boundary cases.[10– 12] In the past several decades, the QISM has become one of the most powerful methods in solving the spectrum problem of integrable systems. In particular, the development of the nested algebraic Bethe ansatz[13– 16] enables one to diagonalize the multicomponent integrable models in a systematic way. However, the QISM fails when considering the anti-periodic boundary condition (APBC) because the particle number is not conserved and the U(1) symmetry is broken in this case. The APBC is physically interesting because it is related to the topological properties of the matter, with Jordan– Wigner transformation, it describes a p-wave Josephson junction in a spinless Luttinger liquid. For example, the APBC in the XX spin chain corresponds to a boundary coupling term of 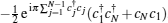, where cj and are the annihilation and creation operators of fermions, which induces some topological excitations.[17] Recently, a systematic method, i.e. the off-diagonal Bethe ansatz (ODBA), [17] was proposed with which the authors successfully obtained the exact solutions for models without the U(1) symmetry. The ODBA overcomes the obstacle of the absence of a reference state, which is crucial in the conventional Bethe ansatz methods. Several long-standing problems will be solved exactly very soon.[18– 22]

The Dzyaloshinsky– Moriya interaction (DMI)[23– 25]HDM = D· (S × S) is an antisymmetric spin-spin interaction which plays an essential role in various fields.[26– 29] The historical study of the Hamiltonian[30] of the Heisenberg model with the DMI is

where J is the exchange integral, Δ is the anisotropic parameter, D is the Dzyaloshinsky vector, and are the spin-1/2 operators. The parameters J, Δ , and D are usually independent of the sites.

In this paper, we propose an integrable Heisenberg model with exchange couplings and DMI that are dependent on the sites. (In the following we call them the site-dependent exchange couplings and DMI.) We obtain exact solutions, including the energy spectrum and eigenstates of the system with different boundary conditions. The rest of this paper is organized as follows. In Section  2, we introduce the Hamiltonian and the rotation transformation which is essential in understanding the physics. In Section  3, we use the QISM to solve the Hamiltonian with the PBC. In Section  4, the ODBA is used to study the system with the APBC and we find a coherence reference state that is induced by the topological boundary. Our concluding remarks are discussed in Section  5.

2. The Hamiltonian

We consider a one-dimensional Heisenberg model with the site-dependent exchange couplings and DMI. The Hamiltonian is

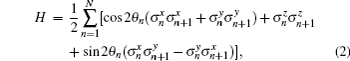
where N is the length of the system, σ α (α = x, y, z) is the 2 × 2 Pauli matrix, and {θ n, n = 1, … , N} are arbitrary site-dependent parameters, which are real to ensure the hermiticity of the Hamiltonian. When all of the site-dependent parameters {θ n} are equal to a constant, the system is reduced to the Hamiltonian (52) up to scaling factor.

We first introduce a rotation of the spins in the x– y plane with the transformation matrix

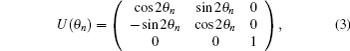
which is a 3 × 3 orthogonal matrix and satisfies U (θ n)TU (θ n) = id, U (θ n)T = U (− θ n) (T means the transposition). We can easily derive the following relations,

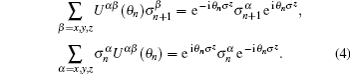
Under the transformation (3), the Hamiltonian  (2) can be expressed as

Furthermore, we define

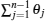
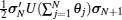
which means that we rotate each of the spins at site n with an angle with respect to the spin at site n = 1. The interaction in the bulk then reads and the boundary coupling arrives at . We note that there would be a phase accumulation at the boundary which determines the physics of the system  (2). In the PBC case, i.e. σ N+ 1 = σ 1, the boundary coupling is

Therefore, if (n is integer), the eigenvalues of system  (2) are the same as that of the isotropic XXX Heisenberg spin chain but the eigenstates of these two systems are different by some rotations. In the following, we consider the most general case that all the {θ j} are arbitrary.

3. Periodic boundary condition

In this section we show the integrability of the Hamiltonian  (2) with the PBC. The R-matrix of the system is R0, n(u) = u + P0, n, where u is the spectral parameter and is the permutation operator which acts on the quantum space Vn and the auxiliary space V0. The permutation operator possesses the following properties for arbitrary operator Am,

Below we list some important properties of the R-matrix,

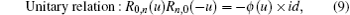
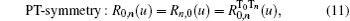
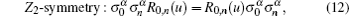
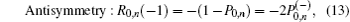
where ϕ (u) = u2 − 1, Tj is the transposition in the space j and 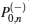is the antisymmetric projection operator. Besides Eqs.  (8)– (13), the R-matrix and arbitrary constant matrix M satisfy the following commutation relation,

The Lax operator is defined as L0, n(u) = R0, n(u). Considering two Lax operators L0, n(u) and L0′ , n(ν ) with the same quantum space Vn and different auxiliary spaces V0 and V0′ , the product of L0, n(u) and L0′ , n(ν ) acts in the space Vn ⊗ V0 ⊗ V0′ and satisfies the Yang– Baxter equation (YBE)

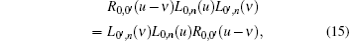
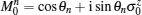
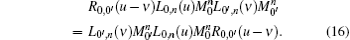
which defines the underlying algebraic structure and is the cornerstone of solving the quantum integrable models. We consider a constant matrix which is defined in the auxiliary space V0. With the help of the commutation relation (14) and the YBE (15), we can prove that

Define the monodromy matrix T0(u) as

which is a matrix in the auxiliary space V0 with its matrix elements being the operators acting on the quantum spaces. The transfer matrix is the trace of the monodromy matrix in the auxiliary space, i.e. t(u) = tr0T0(u). Obviously,

From Eqs.  (16) and (18), we can prove that the monodromy matrix T0(u) satisfies the YBE

Left multiplying Eq.  (19) with 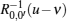and taking the trace in the auxiliary spaces 0 and 0′ , we obtain

which means that the transfer matrices with different spectral parameters are mutually commutative. In such a sense, the transfer matrix serves as a generating functional of conserved quantities and ensures the integrability of the system. The Hamiltonian  (2) is the first derivative of the logarithm of the transfer matrix

We denote the monodromy matrix as

From the YBE (19), the elements of the monodromy matrix satisfy the following commutation relations

We define the vacuum state of the system as

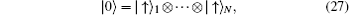
where | ↑ 〉 n is the spin up state of site n. The monodromy matrix T0(u) acting on the vacuum state gives

We see that the elements A(u) and D(u) acting on the vacuum state give the eigenvalues a(u) and d(u), respectively. The element C(u) acting on the vacuum state is zero and B(u) is the spin flipping operator, which are used to generate the eigenstates of the system.

Assume that the eigenstate of the system takes the form of

where {μ j, j = 1, … , M} are the Bethe roots and M is the number of flipped spins. With the notation (22), the transfer matrix is

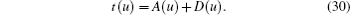
Acting t(u) on the Bethe state (29), we should exchange the positions of A(u), D(u) and {B(μ j)} until A(u) and D(u) arrive at vacuum state. Repeat using the commutation relations  (24) and (25), we then obtain

With the help of the commutation relations  (31) and (32), the transfer matrix acting on the assumed Bethe state gives

where the eigenvalue term Λ (u) is

and the “ unwanted term” Λ j(u) is

If all of the unwanted terms {Λ j(u)} are zero, then the assumed Bethe state  (29) is indeed the eigenstate of the transfer matrix, which requires that the Bethe roots {μ j} should satisfy the following Bethe ansatz equation (BAE),

For convenience, we put μ j = λ j − 1/2 and the BAE becomes

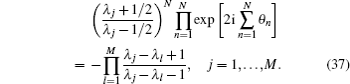
The eigenvalue of the Hamiltonian is

4. Anti-periodic boundary condition

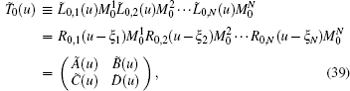
Now we consider the Hamiltonian (2) with the APBC, i.e. . In this case, the corresponding monodromy matrix is defined as

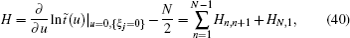
where {ξ j, j = 1, … , N} are the inhomogeneous parameters and à (u), B̃ (u), C̃ (u), D̃ (u) also satisfy the commutation relations  (23)– (26). The corresponding transfer matrix is . Using the same method in the PBC case, we can prove that the transfer matrix with different spectral parameters commute with each other, [t̃ (u), t̃ (ν )] = 0, which indicates that the system with the APBC is also integrable. The Hamiltonian is given by the first order derivative of the logarithm of the transfer matrix as

with the boundary relation

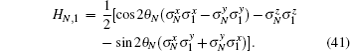
We first consider the effect of the boundary Eq.  (41) and introduce a global rotation

We define

and then we have

With the global transformation (42) on each spin, the couplings in the bulk become . Therefore, the energy spectrum of the system (40) should be equal to the XXX spin chain with the APBC but the corresponding eigenstates, which are related with the local rotation  (3) and the global transformation (42), are different.

4.1. The operator product identities of the transfer matrix

Due to the APBC, the state (27) is no longer a reference state of the system and the QISM does not work. We adopt the ODBA developed in Ref.  [17] to solve the problem. The main idea of the ODBA is as follows. First we should find the operator production identities of the transfer matrix. Based on these identities, we can construct the inhomogeneous T– Q relation. From which we can obtain the eigenvalues and eigenstates of the transfer matrix and the Bethe ansatz equations. In order to obtain the operator identity, we evaluate the transfer matrix t̃ (u) at the point ξ j,

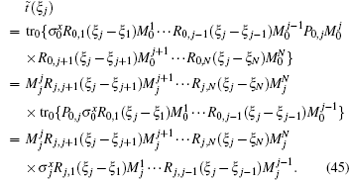
From Eq.  (45), we see that t̃ (ξ j) is a reduced monodromy matrix by treating the j-th quantum space as the auxiliary space. The transfer matrix t̃ (u) at another point ξ j − 1 reads

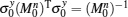
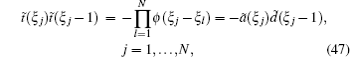
In the derivations, we have used the crossing relation  (10) of the R-matrix and . From Eqs.  (45) and (46) and the unitary property (9) of the R-matrix, we obtain that the transfer matrix satisfies the following operator product identities

where

Correspondingly, the eigenvalues Λ ̃ (u) of the transfer matrix t̃ (u) should satisfy the functional relation

We note that the eigenvalue Λ ̃ (u) is a polynomial with degree N − 1, which can be completely determined by the N identities [Eq.  (49)] of {Λ ̃ (u)}.

4.2. Inhomogeneous T-Q relation and Bethe ansatz equation

Based on Eq.  (49), we parameterize the eigenvalue of the transfer matrix as

where ã (u) and d̃ (u) are defined in Eq.  (48), the function Q̃ (u) is

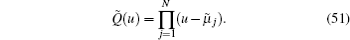
Notice that d(ξ j) = a(ξ j − 1) = 0, the ansatz equation  (50) satisfies Eq.  (49) automatically. As the eigenvalue of the transfer matrix is a polynomial, the residual of Eq.  (50) must be zero, which gives the constraint of the values of the Bethe roots 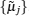. Taking the homogenous limit {ξ j} → 0, the BAE can be written as

where . The eigenvalue of the Hamiltonian is

We find that the site-dependent parameters {θ j} do not enter the BAE and the eigenvalue, which means that the effects of the site-dependent coupling is canceled by the APBC and is consistent with the analysis in Section  4.

4.3. Orthogonal basis

We introduce the state | 0〉 with all of the spins up and its dual state 〈 0| ,

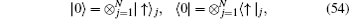
the elements of the monodromy matrix T̃ 0(u) that act on them are

where ã (u) and d̃ (u) are given by Eq.  (48). Now we introduce the left and right states parameterized by the N inhomogeneous parameters {ξ j},

It is easy to prove that equations  (56) and (57) are eigenstates of D̃ (u) that

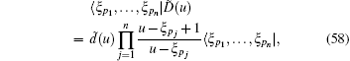
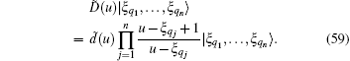
The orthogonal relation between the left state and the right state is

where

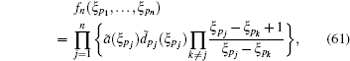
with f0 = 〈 0| 0〉 = 1. The function d̃ l(u) is defined as

Thus the left/right states in Eq.  (56)/Eq.  (57) form a 2N-dimensional orthogonal left/right basis of the dual/the Hilbert space, and any left/right state can be decomposed as a unique combination of these basis.

4.4. The Bethe states

Since the left states {〈 ξ p1, … , ξ pn| , n = 0, … , N, 1 ≤ p1 < p2 < … < pn ≤ N} given by Eq.  (56) form a basis of the dual Hilbert space, the left eigenstate 〈 Ψ | can be expressed as

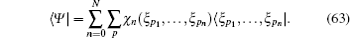
The orthogonal relation Eq.  (60) allows us to determine the coefficientχ n (ξ p1, … , ξ pn) as

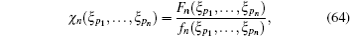
where Fn(ξ p1, … , ξ pn) is defined as

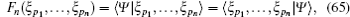
with F0 = 〈 Ψ | 0〉 = 1.

As 〈 Ψ | is an eigenstate of the transfer matrix t̃ (u), i.e., 〈 Ψ | t̃ (u) = 〈 Ψ | Λ ̃ (u), with Λ ̃ (u) given by Eq.  (50), we can obtain the following relation by considering the quantity 〈 Ψ | t̃ (ξ pn+ 1)| ξ p1, … , ξ pn〉 ,

We consider the following Bethe state

where {λ j| j = 1, … , N} are the Bethe roots of the BAE  (52) and | Ω ; {ξ j}〉 is a generalized reference state to be determined so that the condition  (66) is fulfilled. For an eigenvalue Λ ̃ (u) given by the T– Q relation  (50), its value at the inhomogeneous point ξ j takes

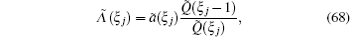
thus from 〈 ξ p1, … , ξ pn| λ 1, … , λ N〉 = Fn(ξ p1, … , ξ pn) we can prove that

We propose the following ansatz for the reference state | Ω ; {ξ j}〉

where the operator B̃ − is defined as

From the definition of the monodromy matrix T̃ (u), we can find the explicit expression of the operator B̃ − as

To prove that | Ω ; {ξ j}〉 given by Eq.  (70) indeed satisfies the relation (66), we introduce the inner product

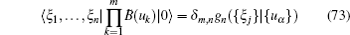
with g0 = 〈 0| 0〉 = 1. We can derive the following recursive relation for gn({ξ j}| {uα })

Define

the initial condition of ḡ n({ξ pj}) is ḡ 0 = 1. Thus, with the recursive relation  (74) of gn({ξ j}| {uα }) and the following identity

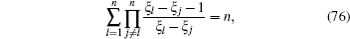
we can derive the recursive relation of ḡ n({ξ j}),

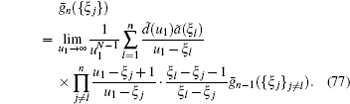
which determines the functions {ḡ n({ξ j})| n = 0, … , N} as

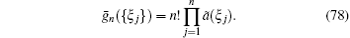
By substituting Eq.  (78) into Eq.  (75), we find that the state | Ω ; {ξ j}〉 given by Eq.  (70) indeed satisfies the relation (66). In the homogeneous limit {ξ j → 0}, the reference state  (70) becomes

This implies that the homogeneous limit of the Bethe state  (67) with the reference state  (70) gives rise to the eigenstate of the corresponding homogeneous transfer matrix, which provides that the parameters {λ j| j = 1, … , N} satisfy the BAE  (52).

5. Conclusion

In summary, we proposed an integrable spin-1/2 Heisenberg model with site-dependent exchange coupling and DM interaction. We studied the system with different boundary conditions. By employing the QISM method, we obtained the eigenvalues and the Bethe ansatz equation of the system with PBC. The site-dependent parameters enter the BAE, thus affecting the eigenvalues and physics of the system. We also obtained the exact solution of the system with APBC and studied the boundary effect. The operator identities of the transfer matrix at the inhomogeneous points are proven at the operator level. We constructed the T– Q relation based on them. From it, we obtained the energy spectrum of the Hamiltonian. The corresponding eigenstates are also constructed. We find that due to the topological nature of APBC, the reference state is a perfect coherence state, which is very different from that of PBC, where the reference state is a direct product state.

Reference
1 Yang C N 1968 Phys. Rev. 168 1920 DOI:10.1103/PhysRev.168.1920 [Cited within:1]
2 Yang C N 1967 Phys. Rev. Lett. 19 1312 DOI:10.1103/PhysRevLett.19.1312 [Cited within:1]
3 Baxter R J 1971 Phys. Rev. Lett. 26 832 DOI:10.1103/PhysRevLett.26.832 [Cited within:1]
4 Baxter R J 1971 Phys. Rev. Lett. 26 834 DOI:10.1103/PhysRevLett.26.834 [Cited within:1]
5 Sklyanin E K and Faddeev L D 1978 Sov. Phys. Dokl. 23 902 [Cited within:1]
6 Takhtadzhan L A and Faddeev L D 1979 Rush. Math. Surveys. 34 11 DOI:10.1070/RM1979v034n05ABEH003909 [Cited within:1]
7 Sklyanin E K, Takhtajan L A and Faddeev L D 1980 Theor. Math. Phys. 40 688 DOI:10.1007/BF01018718 [Cited within:1]
8 Faddeev L D 1980 Sov. Sci. Rev. Math. C 1 107 [Cited within:1]
9 Sklyanin E K 1982 J. Sov. Math. 19 1546 DOI:10.1007/BF01091462 [Cited within:1]
10 Cherednik I 1984 Theor. Math. Phys. 61 977 DOI:10.1007/BF01038545 [Cited within:1]
11 Alcaraz F C, Barber M N, Batchelor M T, Baxter R J and Quispel G R W 1987 J. Phys. A: Math. Gen. 20 6397 DOI:10.1088/0305-4470/20/18/038 [Cited within:1]
12 Sklyanin E K 1988 J. Phys. A: Math. Gen. 21 2375 DOI:10.1088/0305-4470/21/10/015 [Cited within:1]
13 Kulish P P and Reshetikhin N 1981 JHTP 53 108 [Cited within:1]
14 De Vega H J and Lopes E 1911 Phys. Rev. Lett. 67 489 [Cited within:1]
15 Lopes E 1992 Nucl. Phys. B 370 636 DOI:10.1016/0550-3213(92)90426-C [Cited within:1]
16 Sutherland B 1975 Phys. Rev. B 12 3795 DOI:10.1103/PhysRevB.12.3795 [Cited within:1]
17 Cao J P, Yang W L, Shi K J and Wang Y P 2013 Phys. Rev. Lett. 111 137201 DOI:10.1103/PhysRevLett.111.137201 [Cited within:3]
18 Cao J P, Yang W L, Shi K J and Wang Y P 2013 Nucl. Phys. B 877 152 DOI:10.1016/j.nuclphysb.2013.10.001 [Cited within:1]
19 Li Y Y, Cao J P, Yang W L, Shi K J and Wang Y P 2014 Nucl. Phys. B 884 17 DOI:10.1016/j.nuclphysb.2014.04.010 [Cited within:1]
20 Cao J P, Yang W L, Shi K J and Wang Y P 2014 Nucl. Phys. B 886 185 DOI:10.1016/j.nuclphysb.2014.06.026 [Cited within:1]
21 Cao J P, Yang W L, Shi K J and Wang Y P 2014 J. High Energy Phys. 04 143 [Cited within:1]
22 Nepomechie R I 2013 J. Phys. A: Math. Theor. 46 442002 DOI:10.1088/1751-8113/46/44/442002 [Cited within:1]
23 Dzyaloshinsky I E 1958 J. Phys. Chem. Solids 4 241 DOI:10.1016/0022-3697(58)90076-3 [Cited within:1]
24 Moriya T 1960 Phys. Rev. Lett. 4 228 DOI:10.1103/PhysRevLett.4.228 [Cited within:1]
25 Moriya T 1960 Phys. Rev. 120 91 DOI:10.1103/PhysRev.120.91 [Cited within:1]
26 Yi L, Büttner G, Usadel D and Yao K L 1993 Phys. Rev. B 47 254 DOI:10.1103/PhysRevLett.71.467 [Cited within:1]
27 Koshibae W, Ohta Y and Maekawa S 1993 Phys. Rev. Lett. 71 467 DOI:10.1103/PhysRevLett.71.467 [Cited within:1]
28 de Sousa J R, de Albuquerque D F and Fittipaldi I P 1994 Phys. Lett. A 191 275 DOI:10.1016/0375-9601(94)90139-2 [Cited within:1]
29 Daniel M and Amuda R 1996 Phys. Rev. B 53 R2930 DOI:10.1103/PhysRevB.53.R2930 [Cited within:1]
30 Zhang D G, Cheng L and Li B Z 1999 Phys. Rev. B 59 8379 DOI:10.1103/PhysRevB.59.8379 [Cited within:1]