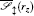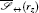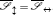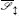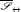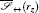†Corresponding author. E-mail: jfjiang@mail.ustc.edu.cn
‡Corresponding author. E-mail: luhj9404@mail.ustc.edu.cn
*Project supported by the National Natural Science Foundation of China (Grant Nos. 11305074, 11135002, and 11275083).
In the analysis of quantum discord, the minimization of average entropy traditionally involved over orthogonal projective measurements may be attained at more optimal decompositions by using the positive-operator-valued measure (POVM) measurements. Taking advantage of the quantum steering ellipsoid in combination with three-element POVM optimization, we show that, for a family of two-qubit X states locally interacting with Markovian non-dissipative environments, the decay rates of quantum discord show smooth dynamical evolutions without any sudden change. This is in contrast to two-element orthogonal projective measurements, in which case the sudden change of the decay rates of quantum and classical decoherences may be a common phenomenon. Notwithstanding this, we find that a subset of X states (including the Bell diagonal states) involving POVM optimization can still preserve the sudden change character as usual.
Quantum correlation can exist even without entanglement.[1] This deducing was further presented independently by Henderson et al.[2] and Ollivier et al., [3] who demonstrate that, the two equivalent definitions of classical mutual information entropy can be generalized to the quantum version.[4] In the quantum case of composite systems 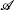
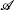
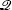
The quantum discord, as a measure to quantify the quantum correlation, can capture much more non-classical correlation than that of the entanglement measure. Meanwhile, the dynamical evolutions of quantum discord under the Markovian and non-Markovian noises have also been investigated in Refs. [18]– [21]. It is shown that discord, contrary to entanglement, behaves much more robustly in resisting decoherence. In addition, the dynamics of discord conducted by non-dissipative Markovian environments result in a variety of interesting decoherence phenomena, [22– 29] in which a freeze and sudden transition of classical and quantum correlation dynamics can emerge for the Bell diagonal states of two qubit states. The sudden transition behaviors show that the quantum and classical correlations remain constant and unaffected by decoherence before and after the transition point, respectively, while the sudden change behaviors just mean that the dynamics of quantum and classical correlations are non-smooth. Obviously, the behaviors of the sudden transition are included in the cases of the sudden change.
It has been known that the sudden change behaviors result from the minimization procedure over the complete set of orthogonal projective measurements. Furthermore, the sudden change point can be reflected in the detailed calculation, in which the minimization will give rise to a non-smooth decay rate of discord due to the different choices of optimal observables.[30] Nevertheless, the minimization procedures involved over all possible measurements bring some difficulties in the analysis of discord. For bipartite mixed states, the analytical solution for the three-parameter family of Bell diagonal states was first obtained in Ref. [31], and lately Ali et al.[32] attempted to generalize the analytic form to the X states, in which the optimal observable is limited to a set of Pauli operators. Recently, researchers[33– 35] have made clear that the optimization for some two-qubit X states is state-dependent and out of the finite set as mentioned above. Meanwhile, the authors[36– 40] found that, in the cases of POVM measurements, the minimization procedures may lead to more optimal quantities of discord than that given by orthogonal measurements for a family of X states.
In this paper, by using the visualized tool of the quantum steering ellipsoid, [41] we show that choosing three-element POVM measurements rather than orthogonal ones to analyze discord and classical correlation may obtain more optimal quantities for a family of X states. Furthermore, we find that, for some initial X states undergoing two-sided phase damping (PD), the dynamics of discord and classical correlation in the optimization over three-element POVM measurements exhibit two smooth evolving curves without any sudden change point, in contrast to the cases of the two-element orthogonal measurements. However, for the Bell diagonal states it turns out that the two alternative optimal procedures would lead to the same dynamics of discord and classical correlation, and that the sudden change point still exists.
For a bipartite quantum system 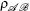
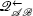
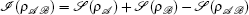
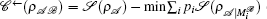
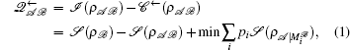
where S(ρ ) = − Trρ log2ρ is the von Neumann entropy and in the quantum case the minimum is taken over a complete set of POVMs 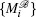

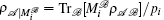
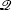
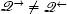
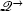
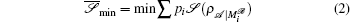
in Eq. (1). For convenience of analysis, we only consider a class of X states with positive real matrix elements
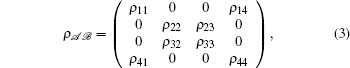
for which the positivity requires 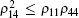
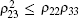
With the aid of a geometric picture of the steering ellipsoid abbreviated as 𝔈 , one can visualize the optimal measurement of interest through finding an optimal convex combination of post-measurement ensemble 
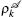
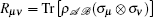
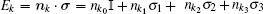
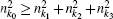

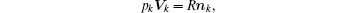
where Vk = (1, xk, yk, zk)T is the four-component vector of normalized marginal state 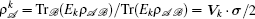
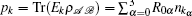
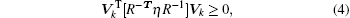
where the Minkowski metric tensor η = diag[1, – 1, – 1, – 1]. One can write out its steering ellipsoid equation[30]
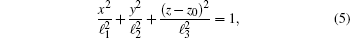
where the three semi-axis lengths along the x, y, z axes and the location of the center of the 𝔈 correspond to

respectively. The reduced state ρ A corresponds to point A as shown in Fig. 2(a), and its Bloch vector is (0, 0, Rz) with Rz = ρ 11 + ρ 22 − ρ 33 − ρ 44. The decomposed ensemble 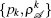


A horizontal chord passing through the point A intersects with E, on which the two crossing points of E and F correspond to the decomposed two states induced by the two-element projective measurements (I ± σ x)/2 acting on partition ℬ with probabilities pE = pF = 1/2. The coordinates of E and F are 

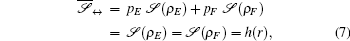
where

for x ∈ [0, 1] is a binary entropy function and
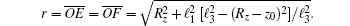
Similarly, the average entropy 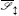
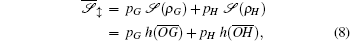
where
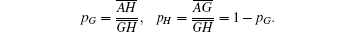
The intersections of E and z axis correspond to the two vertices of G and H, whose coordinates are labeled as (0, zG) and (0, zH), respectively.
Specifically, introducing a local filtering operation (LFO)[39, 40] of the form
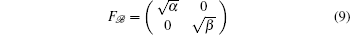
satisfies 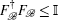
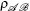
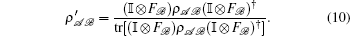
In the Bloch vector representation, equation (10) can be transformed to
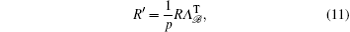
where 
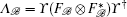
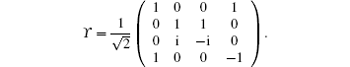
Under LFO, the 𝔈 remains invariant and the three semi-axis lengths and the location of center will be the same as Eq. (6), whereas the Bloch vector of 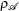
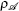
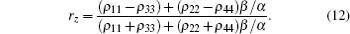
Consequently, we have
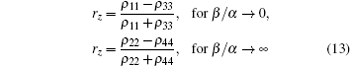
corresponding to the σ z component of G or H in the Bloch representation, respectively. Thus, the σ z component of 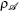
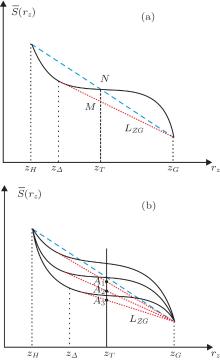
Remark 1 When the ellipse center is located at the origin, the function 
Immediately, one can reduce Eq. (8) to 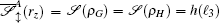
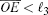
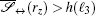
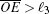
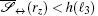
The two functions of 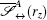
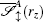
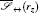
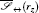
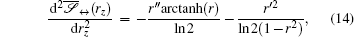
where 
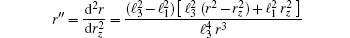
with 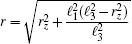
(i) For ℓ 1 > ℓ 3, one can obtain an inequality

where the inequality (15) follows the Taylor expansion of 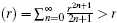
(ii) For ℓ 1 < ℓ 3, similarly one can get

Thus the function of 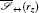
Having proved that, for the trivial case of ℓ 1 < ℓ 3 with z0 = 0, the function of 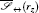
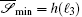
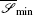
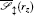
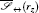
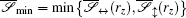
Let us now turn to the discussion of a non-trivial case that the center of E departs from the origin O in the x– z plane, three-element POVM measurements will be introduced by means of the geometric picture approaches.[30, 37] Given an E with z0 ≠ 0 (i.e., ρ 11ρ 22 ≠ ρ 33ρ 44), one can use LFO to move the point A along the z axis and leaves E unchanged.[30] It is possible that the z coordinate of the moving point A corresponding to a certain value zT makes 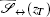
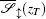

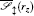
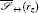
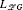
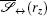
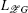
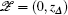

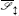

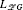
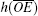

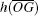
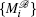
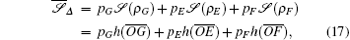
where 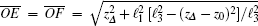
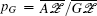
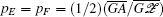
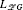
In terms of the three-element POVM measurements, we set out to study the dynamical evolution of discord of the X states and reinvestigate the sudden change. Specifically, we shall introduce a typical kind of unital noise channel, [4] i.e., phase damping (PD) channel, which is a decoherence model with no energy exchange between the system and environment. This channel can take the identity operator to itself so that 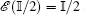


where γ represents the decay rate as a function of time t. There is an associated two-sided quantum map 
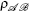
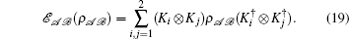
In the PD channel, equation (5) is transformed to the form of
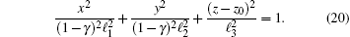
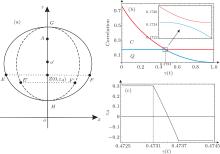
On the scaled down surface of the 𝔈 , each point shrinks to the z axis. Without loss of generality, one only needs to consider the E in the x– z plane as shown in Fig. 1(a).
Remark 2 For the X states with ρ 11ρ 22 = ρ 33ρ 44 satisfying ℓ 1 > ℓ 3, when passing through the PD channels, the dynamics of discord and classical correlations will exhibit a sudden change.
The PD channels keep the center coordinate O and 
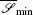
Remark 3 For a family of initial X states with ρ 11ρ 22 ≠ ρ 33ρ 44 and 
For the decay rate γ (t), the time instant t0 of 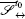
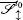
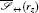
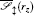
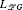
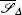




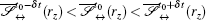

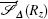
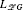
A concrete example of dynamical behaviour is revealed by Fig. 1(b) to show that the entire evolution process from classical to quantum decoherence is smooth and does not manifest any sudden change. In Fig. 1(c), the value zΔ corresponding to z axis coordinate of point 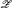
In this work, we have combined the convex analysis with the geometric picture of the available decomposition of local state in the steering ellipsoid, and proved that choosing the three-element POVM measurements instead of two-element orthogonal measurements can give more optimal quantities of discord for a family of X states. Furthermore, by means of the three-element POVM measurements we presented that, except for those initial states with ρ 11ρ 22 = ρ 33ρ 44, there does not exist any sudden change for a family of X states under the phase damping channel. One interesting conclusion is that the decoherence of some initial states with the sudden transition character becomes a smooth transition due to more optimal three-element POVM measurements. The physical mechanics of the sudden and smooth transition may be valuable for further considerations. In addition, the revised measure of discord defined by the three-element POVM measurements can be used to reinvestigate the correlation dynamics of a composite system under the dissipative environments, and may reveal the conditions of presence or absence of the sudden change points compared to that given by two-element orthogonal measurements.
| 1 |
|
| 2 |
|
| 3 |
|
| 4 |
|
| 5 |
|
| 6 |
|
| 7 |
|
| 8 |
|
| 9 |
|
| 10 |
|
| 11 |
|
| 12 |
|
| 13 |
|
| 14 |
|
| 15 |
|
| 16 |
|
| 17 |
|
| 18 |
|
| 19 |
|
| 20 |
|
| 21 |
|
| 22 |
|
| 23 |
|
| 24 |
|
| 25 |
|
| 26 |
|
| 27 |
|
| 28 |
|
| 29 |
|
| 30 |
|
| 31 |
|
| 32 |
|
| 33 |
|
| 34 |
|
| 35 |
|
| 36 |
|
| 37 |
|
| 38 |
|
| 39 |
|
| 40 |
|
| 41 | [Cited within:1] |