Cite this Article
Xue Chan, Gao Fei, Liu Yong-Xin, Liu Jia, Wang You-Nian. Phase shift effects of radio-frequency bias on ion energy distribution in continuous wave and pulse modulated inductively coupled plasmas. Chinese Physics B, 2018, 27(4): 045202  
Permissions
Phase shift effects of radio-frequency bias on ion energy distribution in continuous wave and pulse modulated inductively coupled plasmas
Xue Chan1, Gao Fei1, †, Liu Yong-Xin1, Liu Jia2, Wang You-Nian1, ‡
Key Laboratory of Materials Modification by Laser, Ion, and Electron Beams (Ministry of Education), School of Physics, Dalian University of Technology, Dalian 116024, China
Shanghai Institute of Space Propulsion, Shanghai 201112, China

 

† Corresponding author. E-mail: fgao@dlut.edu.cn ynwang@dlut.edu.cn

Abstract

A retarding field energy analyzer (RFEA) is used to measure the time-averaged ion energy distributions (IEDs) on the substrate in both continuous wave (CW) and synchronous pulse modulated radio-frequency (RF) inductively coupled Ar plasmas (ICPs). The effects of the phase shift θ between the RF bias voltage and the RF source on the IED is investigated under various discharge conditions. It is found that as θ increases from 0 to π, the IED moves towards the low-energy side, and its energy width becomes narrower. In order to figure out the physical mechanism, the voltage waveforms on the substrate are also measured. The results show that as θ increases from 0 to π, the amplitude of the voltage waveform decreases and, meanwhile, the average sheath potential decreases as well. Specifically, the potential drop in the sheath on the substrate exhibits a maximum value at the same phase (i.e., θ = 0) and a minimum value at the opposite phase (i.e., θ = π). Therefore, when ions traverse across the sheath region above the substrate, they obtain less energies at lower sheath potential drop, leading to lower ion energy. Besides, as θ increases from π to 2π, the IEDs and their energy widths change reversely.

PACS: ;52.80.Yr;;52.70.-m;;52.20.Hv;;52.40.Kh;
Keyword:;ion energy distribution;;phase shift;;synchronous pulse modulated;;inductively coupled plasmas;
1. Introduction

As is well known, the energy and flux of ions bombarding the substrate can be controlled independently by applying a radio-frequency (RF) bias to the substrate in radio-frequency (RF) inductively coupled plasmas (ICPs).[1–3] Therefore, ICP sources can be extended to the application of material modification.[4] Moreover, since the biased substrate can affect the ion energy distribution (IED), which will further influence the surface reactions and the etching rate,[3] and provide additional freedom degrees of plasma modulation, a series of investigations have been carried out for better controlling the IED in etching processing.[2,5]

By using a floating ion energy analyzer and a hybrid model, Edelberg and Aydil investigated the effect of the bias frequency on the IEDs in Ar and Ne ICPs.[3] It was found that a lower bias frequency ( ) can cause a bimodal IED, while a higher bias frequency ( ) can lead to a single-peaked IED. This is because ions can respond to the RF oscillation of the sheath field instantaneously at a lower RF frequency. Furthermore, Edelberg and Aydil[3] also studied the effects of the electron density, electron temperature, bias power and source power on the IEDs, and they found that by increasing the electron density/temperature or reducing the bias/source power, the IEDs can become narrower.

By employing a quadrupole mass spectrometer, Gudmundsson[6] found that in H2 and Ar/H2 plasmas, a higher pressure can cause a narrower IED and consequently lower mean ion energy. There are two different explanations for the lower mean ion energy at a higher pressure: 1) at a higher pressure, the sheath thickness is comparable to the ion mean free path, and thus the elastic and charge exchange collisions in the sheath may occur more frequently,[6–8] which will lead to a lower mean ion energy; 2) a higher pressure can lead to a higher sheath boundary potential and lower wall potential. As a result, the mean ion energy is lower at a higher pressure.[9]

Agarwal et al.[10] discussed the influences of the pulse modulated scheme on IEDs in Ar/Cl2 ICPs. They showed that when the time-averaged power is fixed, the IED slightly moves to the low-energy side with the increase of the peak power. They[11] also found that in synchronously pulsed ICPs, as the pulse phase lag (i.e., the shift of the pulse modulated signals between the source and bias powers) increases, the ion energy and angular distributions (IEADs) of ions bombarding the substrate exhibit significant variations at different pulse phases (including initial active-glow, late active-glow, initial after-glow, and late after-glow). In addition, Banna et al.[12] discussed the effect of the phase lag on the synchronous pulse modulated Ar ICP in simulation, and found that when the phase lag is not zero, the IED may exhibit a multi-peak profile, which is caused by the shift of the pulse modulated signals between the source power and bias power.

Furthermore, recent researches have shown that the synchronous pulse modulations of both source and biased powers have the potential to provide additional degrees of freedom,[10] increase plasma uniformity,[13,14] and reduce charge damage[12] in the etching process. Therefore, the effects of the RF phase shift θ (i.e., the phase shift of the RF bias with respect to the RF source, which has been little studied so far) on the IEDs are investigated in synchronous pulse modulated discharges in this paper. First of all, the effect of the phase shift θ on the IED in CW discharge is investigated, and then the experimental results in pulse modulated discharge are also given. It is found that the energies of the ions striking the substrate exhibit a dependence somewhat on phase shift θ, and hence the voltage waveform on the substrate is studied as well.

The rest of this paper is structured as follows. In Section 2, the experimental setup is briefly described. The experimental results are discussed in CW and pulse modulated discharge in Section 3. In the Section 4, some conclusions are drawn.

2. Experimental setup

The schematic diagram of the experimental setup is shown in Fig. 1. A two-turn planar coil, cooled by recycling water, is placed on the quartz window on the top of the chamber. The RF power is delivered to the coil through a matching network. The substrate, driven by a biased RF voltage, is located at 10.5 cm below the quartz window. In experiment, the RF frequencies of both source and bias voltages are both fixed to be 13.56 MHz. The stainless cylindrical chamber is grounded. More details about the chamber have been given elsewhere.[15–20] A retarding field ion energy analyzer (RFEA) is inset in the center of the substrate, and used to measure the time-averaged IEDs. In synchronous pulse modulated discharge, the source and bias voltages are both pulse modulated. The phase shift θ indicates the RF phase shift of the applied bias voltage waveform with respect to the source voltage waveform in an RF period. The scenarios of phase shifts in CW discharge and pulse modulated discharge are shown in Figs. 2(a) and 2(b), respectively. The working gas in this experiment is Ar.

Fig. 1. (color online) Schematic diagram of plasma reactor, equipped by an REFA diagnostic system.
Fig. 2. (color online) Phase shifts between source and bias voltage waveforms in (a) CW discharge and (b) pulse modulated discharge.
3. Results and discussion
3.1. In CW discharge

Since the characteristics of the pulse modulated plasma in steady state is similar to that in CW discharge[5] and, for simplicity, the influence of the phase shift θ on the IED is first discussed in CW discharge. The dependence of the IED on phase shift θ for the cases of θ =0, π/2, and π at the bias voltage (the peak-peak value) of 200 V is illustrated in Fig. 3. The source power is 200 W, and the pressure is 10 mTorr (1 Torr = 1.33322×102 Pa). We can see that the IEDs generally exhibit bimodal structures. This is because the response time of ions in the sheath (about ) is smaller than the RF period of the bias voltage (about ). As a result, the ions can gain different energies from the sheath field when traversing across the sheath region at different phases, and thus leading to the bimodal structures of the IEDs.[3,6,21]

Fig. 3. (color online) Dependence of the normalized IED on phase shift θ for the cases of θ = 0, π/2, and π at bias voltages of 200 V, pressure of 10 mTorr, and source power of 200 W.

From Fig. 3, it can be found that as the phase shift increases from 0 to π, the IED moves towards the low-energy side and, meanwhile, the energy width shrinks. In order to find out the physical reason, the voltage waveform on the substrate is measured by a high-voltage probe and displayed by a digital oscilloscope, and the results are shown in Fig. 4. We can see that as the phase shift increases from 0 to π, the voltage waveform moves to the positive part and its amplitude decreases. Therefore, the sheath potential, which is primarily determined by the negative part of the voltage waveform, decreases with the phase angle increasing from 0 to π (see the short-dash lines in Fig. 4). Specifically, the potential drop in the sheath reaches its maximum at θ = 0, while it comes up to its minimum at θ = π. As a result, when ions traverse across the sheath region, they could gain less energy from the sheath field at a higher phase angle θ, and thus leading to a shift of the IED towards the low-energy side. This explains the results shown in Fig. 3. As for the variation of bias voltage with phase shift, it can be attributed to the modifications of the electron density and electron temperature. Actually, as the phase shift increases from 0 to π, the electron density and effective electron temperature change slightly, which will modify the sheath above the substrate. Consequently, the bias voltage and self-bias voltage on the substrate will be changed by the modified sheath. Moreover, since the sheath potential decreases with phase shift (see the short-dash lines in Fig. 4(b), which are the amplified dash lines in Fig. 4(a)), as a result, when ions traverse across the sheath to arrive at the substrate, the reduced bias voltage leads to ions getting less energy, which indicates the energy width shrinking in IED.

Fig. 4. (color online) (a) Measured voltage waveforms and (b) sheath potentials on biased substrate at different phase angles θ = 0, π/2, and π. The other conditions are as follows: source power is 100 W, pressure is 10 mTorr, and applied bias voltage (peak–peak voltage) is 200 V.

Figures 5(a1)–5(a3) illustrate the IEDs (at θ = 0, π/2, and π) at different bias voltages with a fixed source power of 100 W and pressure of 10 mTorr. It is found that with the increase of the phase shift, the IED generally moves towards the low-energy region and, meanwhile, the energy width of IEDs shrinks for all bias voltages. This has been discussed above in detail. In addition, as the bias voltage increases, the IED moves towards the high-energy region and, meanwhile, the IED is broadened. Obviously, as the RF bias voltage increases, the potential drop in the sheath will increase. As a result, when the ions traverse across the sheath region, they gain more energies from the sheath field, and thus leading to the higher ion energy. In addition, according to Refs. [7] and [22], the energy width of the IED can be expressed as

Here, is the RF period of the bias voltage and τi is the transit time of ions in the sheath region. Therefore, as the bias voltage decreases, the energy width becomes narrower (since τi shows much weaker dependence on the bias voltage than Vrf).

Fig. 5. (color online) Normalized IED evolutions with phase shift at different bias voltages: (a1) 150 V, (a2) 200 V, and (a3) 250 V, when the source power is 100 W and the pressure is 10 mTorr, at different source powers (b1) 100 W, (b2) 200 W, and (b3) 300 W, when the bias voltage is 200 V and discharge pressure is 10 mTorr, and at different pressures: (c1) 10 mTorr, (c2) 30 mTorr, and (c3) 50 mTorr, when applied bias voltage is 200 V and source power is 100 W.

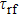
The IEDs (at θ = 0, π/2 and π) at different source powers with a fixed bias voltage of 200 V and pressure of 10 mTorr are displayed in Figs. 5(b1)–5(b3). It is found that with the increase of the source power, the high-energy peak does not exhibit a conspicuous move, while the low-energy peak shifts towards the low-energy region. This may be caused by a thinner sheath thickness at higher electron density (a higher source power),[3] which generally determines/affects the energies of low-energy ions. As a result, the low-energy peak moves towards the low-energy side at a higher source power. On the other hand, the high-energy peak is primarily determined by the RF bias voltage,[3] so the position of the high-energy peak is almost independent of the source power. Furthermore, as the source power increases, the IEDs become increasingly sensitive to the phase shift, i.e., the IED moves more significantly with the phase angle at a higher source power. This may be caused by the thinner sheath thickness, which will lead to the reduction of the transit time of ions in the sheath region, accompanied by the stronger modulation of IED by the sheath.

Besides, the IEDs (at θ = 0, π/2 and π) at different discharge pressures at a fixed bias voltage of 200 V and source power of 100 W are displayed in Figs. 5(c1)–5(c3). It is clear that at various pressures, the IED changes with the phase angle in the same manner as the above. As the pressure increases, the IED moves towards the low-energy side, and the energy width of IED becomes narrower. This is evident since a higher pressure will cause more intensive collisions, including the charge exchange and elastic collisions in the sheath,[6,7] thereby resulting in lower ion energy. Besides, the IED evolves from bimodal structure to single-peaked structure with the increase of pressure. This is taken for granted since the higher pressure can lead to smaller energy width of IED.

Besides, it is worth noting that at θ = π, as the pressure increases from 10 mTorr to 50 mTorr, the IED transforms from bimodal structure to single-peak structure and then the single-peak structure converts back to bimodal structure. In order to find out the physical mechanism, the voltage waves at θ = π under different pressures are drawn in Fig. 6. It is found that as the pressure increases from 10 mTorr to 50 mTorr, the average sheath voltage on the substrate first decreases and then increases. This means that as the pressure increases, when the ions traverse across the sheath above the substrate, the average energy they obtained reduces first and increases later. Therefore, as the pressure increases from 10 mTorr to 30 mTorr, the IED varies from bimodal structure to single peak first and the single peak converts back into bimodal structure afterwards. As for the lower effect of the phase shift on IED at higher pressure, it can be attributed to the enhanced collision at higher pressure. More specifically, at higher pressure, the collision processes exist in the sheath as well, the IED will be influenced not only by the sheath voltage, but also by the energy loss through the collision processes, which may weaken the effect of phase shift on IED.

Fig. 6. (color online) Measured voltage waveforms on the biased substrate at different pressures (10 mTorr, 30 mTorr, and 50 mTorr). The other conditions are as follows: source power is 100 W, applied bias voltage (peak-peak voltage) is 200 V, and phase shift θ = π.
3.2. In synchronous pulse modulated discharge

In this section, the effect of the phase shift on the time-averaged IED in synchronous pulse modulated plasma is investigated, when the frequencies of the source and bias voltages are both fixed at 13.56 MHz, the pulse repetition frequency is 1 kHz, and the pulse duty cycle is 50%. At first, the IED in pulsed plasma is compared with that in CW discharge under the same conditions, and then the effects of the bias voltage, source power, and the working pressure on the IED are discussed.

The time-averaged IEDs and their dependence on the phase shift in CW and synchronous pulse modulated discharge are compared and illustrated in Figs. 7(a) and 7(b). The pressure is 10 mTorr, the source power is 200 W, and the bias voltage is 200 V. By comparing the IEDs at different phase shifts in synchronous pulse modulated discharge with those in CW discharge in Fig. 7, the most remarkable difference is that there are evident low-energy peaks ( ) in pulsed discharge, other features are similar to those in CW discharge. The low-energy peak of the IED in the pulsed discharge is due to the fact that the sheath collapses in the after-glow period, ions would reach the substrate with the same energies as those at the end of the pulse-on period. Like CW discharge, when the phase shift increases from 0 to π, the IEDs move towards the low-energy region and their energy widths become narrower. Obviously, during the active-glow period, the pulsed discharge behaves like the CW discharge, so the dependence of the voltage waveform on the phase shift in the pulse-on period is similar to that in CW discharge. So, it is also true that in pulse discharge when the phase shift increases, the voltage waveform moves to the positive part and, meanwhile its amplitude decreases. However, during the afterglow, since both the source and the bias powers are switched off, the sheath collapses immediately and ion energy would remain the same as that at the end of the active-glow period. Therefore, during the active glow, the effect of the phase shift on IED in synchronous pulse modulated discharge is similar to that in CW discharge. As for the appearance of the lowest-energy peak of the IED in synchronous pulse modulated discharge, it is caused by the sheath collapsing during the afterglow. In addition, it is noted that the lowest-energy peak also shifts towards the low-energy region as the phase shift increases. This might be caused by the difference between the voltage waveforms produced during the active glow at different phase angles. Besides, the IED moves to the low-energy side in the synchronous pulse modulated discharge with respect to that in CW discharge (see Fig. 7). This is taken for granted since less energy is input into the plasma in pulsed discharge than that in CW discharge.

Fig. 7. (color online) Evolutions of time-averaged IED with phase shift in (a) CW discharge and (b) synchronous pulse modulated ICP. Source power is 200 W, bias voltage is 200 V, and discharge pressure is 10 mTorr. Pulse repetition frequency is 1 kHz and its duty cycle is 50%.

Figures 8(a1)–8(a3) show the effects of the RF bias voltage on the IED in pulsed discharge at a fixed source power of 200 W and a fixed pressure of 10 mTorr. We can see that as the bias voltage increases, the IED moves to the high-energy region, and the energy width of IED increases as well. The dependence of the IED on source power and that on working pressure are shown in Figs. 8(b1)–8(b3) and Figs. 8(c1)–8(c3), respectively. The other conditions can be found in the captions of corresponding figures. Like the result in CW discharge, as the source power increases, the energy width of IED increases, the high-energy peak almost has no change in position, while the lower-energy peak moves towards the low-energy side. As the discharge pressure increases, the IED shifts to the low-energy region and, meanwhile the energy width becomes narrower. The corresponding explanations have been given in Subsection 3.1.

Fig. 8. (color online) Dependence of the IED on the phase shift under different bias voltages: (a1) 150 V, (a2) 200 V, and (a3) 250 V with source power of 100 W and pressure of 10 mTorr. Dependence of the IED on the phase shift at different source powers: (b1) 100 W, (b2) 200 W, and (b3) 300 W with a fixed bias voltage of 200 V and a fixed pressure of 10 mTorr. Dependence of the IED on the phase shift at different pressures at 10 mTorr, (c2) 30 mTorr, and (c3) 50 mTorr with a fixed bias voltage of 200 V and a fixed source power of 100 W.
4. Conclusions

The time-averaged IEDs are investigated in both CW and synchronous pulse modulated RF Ar ICPs, by using a retarding field energy analyzer (RFEA). The effects of the phase shift, i.e., the phase angle of the RF bias voltage with respect to the RF source voltage, on the IED and on the substrate are discussed under various discharge conditions. It is found that the dependence of the IED on external parameters in CW discharge is quite similar to that in synchronous pulse modulated discharge, i.e., as the phase shift increases from 0 to π, the IEDs move towards the low-energy side, and their energy widths become narrower. This is because as the phase shift increases, the amplitude of the voltage waveform on the substrate decreases and, meanwhile the voltage waveform moves towards the positive part. Therefore, at a larger phase shift, ions gain less energies when they traverse across the sheath region, thereby leading to lower ion energy. In addition, as the bias voltage increases, the IEDs move towards the high-energy region and their energy widths increase as well. Besides, as the source power increases, the low-energy peak moves to the low-energy side while the position of the high-energy peak is almost independent of the source power. Furthermore, as the pressure increases, the IEDs move to the low-energy side. By comparing the IEDs measured in CW discharge with that in synchronous pulse modulated discharge, there are always evident low-energy peaks ( ), which is associated with the sheath collapsing when the power is off in pulsed discharge. Moreover, the IED in ICP has a significant influence on the etching process in the semiconductor industry, especially in pulse modulated discharge conditions. Therefore, this work is quite important for investigating IED in ICP, which can make contributions to the etching process in the semiconductor industry.

Reference
[1] Keller J H Forster J C Barnes M S 1993 J. Vacuum Sci. Technol. A 11 2487
[2] Schulze J Schüngel E Czarnetzki U 2012 Appl. Phys. Lett. 100 024102
[3] Edelberg E A Aydil E S 1999 J. Appl. Phys. 86 4799
[4] Wegner T Küllig C Meichsner J 2017 Plasma Sources Science and Technology 26 025006
[5] Banna S Agarwal A Cunge G Darnon M Pargon E Joubert O 2012 J. Vacuum Sci. Technol. A 30 040801
[6] Gudmundsson J T 1999 Plasma Sources Science and Technology 8 58
[7] Lieberman M A Gottscho R A 1994 Physics of Thin Films New York H. Francombe Maurice & L. Vossen John 1 119
[8] Wild C Koidl P 1991 J. Appl. Phys. 69 2909
[9] Kortshagen U Zethoff M 1995 Plasma Sources Science and Technology 4 541
[10] Agarwal A Stout P J Banna S Rauf S Collins K 2011 J. Vacuum Sci. Technol. A 29 011017
[11] Agarwal A Stout P J Banna S Rauf S Tokashiki K Lee J Y Collins K 2009 J. Appl. Phys. 106 103305
[12] Banna S Agarwal A Tokashiki K Hong C Rauf S Todorow V Ramaswamy K Collins K Stout P Jeong-Yun L Junho Y Kyoungsub S Sang-Jun C Han-Soo C Hyun-Joong K Changhun L Lymberopoulos D 2009 IEEE Trans. Plasma Sci. 37 1730
[13] Cunge G Vempaire D Sadeghi N 2010 Appl. Phys. Lett. 96 131501
[14] Ono K Tuda M 2000 Thin Solid Films 374 208
[15] Xue C Wen D Q Liu W Zhang Y R Gao F Wang Y N 2017 J. Vacuum Sci. Technol. 35 021301
[16] Liu W Wen D Q Zhao S X Gao F Wang Y N 2015 Plasma Sources Science and Technology 24 025035
[17] Gao F Zhao S X Li X S Wang Y N 2010 Phys. Plasmas 17 103507
[18] Gao F Zhang Y R Zhao S X Li X C Wang Y N 2014 Chin. Phys. 23 115202
[19] Gao F Liu W Zhao S X Zhang Y R Sun C S Wang Y N 2013 Chin. Phys. 22 115205
[20] Gao F Li X C Zhao S X Wang Y N 2012 Chin. Phys. 21 075203
[21] Bruneau B Lafleur T Booth J P Johnson E 2016 Plasma Sources Sci. Technol. 25 025006
[22] Kawamura E Vahedi V Lieberman M A Birdsall C K 1999 Plasma Sources Sci. Technol. 8 R45