The field of spinor Bose– Einstein condensates (BECs) has witnessed an explosion of experimental and theoretical research and been developed into one of the most active fields at the interface between condensed matter physics and atomic physics.^{[1– 5]} A broad scope of problems related to the magnetic properties of this Bose system have been investigated, such as the phase transition, ^{[6– 10]} the spin dynamics, ^{[11– 21]} and the spin domain formation.^{[22– 29]} Indeed, there have been many recent experimental endeavors in this field.^{[30– 35]}

Based on the single mode approximation (SMA), the previous theoretical studies on the spin dynamics provide a mean field theory corresponding to that of a non-rigid pendulum. In this context, the Josephson-type spin dynamics driven by the internal spin– spin interactions have been characterized.^[16] More recently, numerical simulation studies showed that the ferromagnetic spinor BEC is dynamically unstable, ^[17] suggesting that the spin dynamics will not last long before spontaneous symmetry breaking sets in. As an important consequence, multiple spin domains will appear. Experiments have shown that a few damped spin oscillations occur as the domains form.^[18]

Remarkably, experiments carried out by the Stamper– Kurn group at Berkeley have confirmed that the domains indeed form in spinor BECs; ^{[22, 23]} the researchers observed that random domains with varying degrees of transverse magnetization form in a ferromagnetic spinor ⁸⁷Rb Bose gas after the system is rapidly quenched. This experiment has stimulated much interest, and various aspects of this experiment have been analyzed, including a three-dimensional numerical simulation of the GP equations in Ref. [24]. In addition, Lamacraft^[25] discussed the growth of the transverse magnetization correlation functions of the 2D spinor condensates. More recently, research carried out by Saito et al.^[26] and Mias et al.^[27] investigated the dynamical formation of these domains in terms of quantum noise.

The domain formation is a new dynamical feature that appears in spinor BECs in addition to the well-studied spin dynamics. Incorporating this new feature and demonstrating its effect on the spin dynamics are an interesting subject. In this paper, we investigate the spin dynamics considering the dynamical effect of this domain structure. A semiclassical theory beyond the SMA is introduced to demonstrate the spin dynamics in domain formation for any given initial spin configuration. Overlap factors are introduced to characterize the symmetry breaking of the M_F = ± 1 components. A phenomenological term is used to take into account the thermal dissipation of the system, and we observe a qualitative consistency of our theory with the experimental picture of coherent spin dynamics.^[18]

This paper is organized as follows. A description of our semiclassical theory is presented in Section 2. Section 3 presents a detailed analysis of the effect of the domain formation on the spin dynamics. Conclusions are presented in Section 4.

The Hamiltonian of a spin-1 Bose– Einstein condensate can be written in terms of the three component field operators ψ ₁, ψ ₀, and ψ _{– 1}, which correspond to the sublevels M_F = + 1, 0, – 1 of the hyperfine state F = 1. Namely, the Hamiltonian can be expressed as^{[3, 4]}

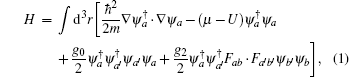where F_α (α = x, y, z) are 3 × 3 spin matrices.^{[3, 4]} for macroscopically occupied Bose systems, it is common to replace the field annihilation operator for the a component by its expectation value, which is called the mean field, i.e., φ _a(r, t) ≡ ⟨ ψ _a (r, t)⟩ . In this context, many theoretical studies have been conducted to investigate the spin dynamics of the ⁸⁷Rb spinor condensate.^{[11, 12, 16– 18]} However, those previous studies have neglected the spontaneous symmetry breaking of the system by assuming that the spatial distributions of the M_F = 0, ± 1 components are the same, the so-called single-mode approximation. Now, if one considers the emergent domain structure, the differences in particle distribution for distinct components should be considered, with different spatial configurations representing the spontaneous symmetry breaking of the system during the domain formation. To incorporate this consideration, we use a different ansatz for the spinor condensates, which can be conveniently expressed as

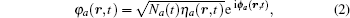where N_a(t) represents the number of condensed particles of the a component, η _a(r, t) denotes the normalized particle distribution with ∫ d³rη _a(r, t) = 1, and ϕ _a(r, t) donates the corresponding phase. The total particle density is expressed as ρ (r, t) = N_a(t)η _a(r, t). By ignoring the excitations, the Hamiltonian is simplified to H = H₀ + H_u + H_s, with

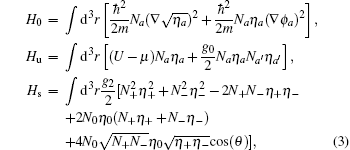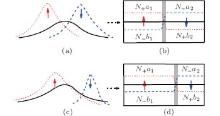
where θ = ϕ ₊ + ϕ _– – 2ϕ ₀ is the relative phase. We proceed to treat the spinor Bose gas with a symmetry-broken state in which a certain magnetic domain structure is formed spontaneously. Within each domain, the atomic magnetic moments are aligned in a preferential direction. A direct consequence of the domain formation is that the M_F = 1 and M_F = – 1 components are spatially separated, as portrayed in Fig.  1(c). The integral ∫ d³rη ₊ η _– measures the overlap between the two components, which is called the overlap factor hereinafter. In general, several overlap factors should be introduced into Eq. 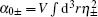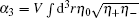(3), e.g., , α ₁ = V ∫ d³rη ₊ η _– , α _2± = V ∫ d³rη ₀η _± , and , where V is the volume of the system. By treating the relative phase θ as a spatially independent constant as in the previous theory, ^{[14, 15, 17, 18, 28]}H_s in Eq.  (3) is rewritten as

Fig. 1.

Figure Option ViewDownloadNew Window

Fig. 1. Schematic two-domain structures for (a) a ferromagnetic spinor BEC with zero total spin and (c) a spin-imbalanced ferromagnetic spinor BEC. Dotted, dashed, and solid lines represent the particle distributions of MF = ± 1 and MF = 0 bosons, respectively. Panels (b) and (d) show the simplified domain structures. Each component has a constant distribution within the domain structure, and the distribution of the MF = 0 bosons is a constant throughout the entire system. The gray region denotes the domain wall. The density distribution shown in the figure is ρ (r, t) = Na(t)η a(r, t), and V+ and V– are the sizes of the two domain structures.

Our model allows the α factor to vary from 1 to 0, corresponding to the case in which the two components are thoroughly mixed to that in which the components are completely separated. The spin-irrelevant term H_u remains a constant because the total density distribution remains approximately unchanged as the domain structure develops; ^{[17, 22, 28]} therefore ρ (r, t)≈ ρ (r, 0). Thus the dynamics of the domain formation are only determined by the Hamiltonian in Eq.  (4).

In the previous experimental study on the spinor dynamics, ^{[18, 32, 33]} it was shown that the initial population imbalance affects much of the dynamical behavior. Therefore, in this paper, we extend our work presented in Ref. [29] to observe the effects of different initial configurations on the spin dynamics in the domain formation process. The Hamiltonian is deduced as follows, with the schematic view of the domain formation shown in Fig.  1(d).

It should be noted that now we have three constraints. First, the particle distribution is normalized. Second, the magnetization in each of the two domains is the same. Third, the total particle profile remains unchanged during the spin domain formation process. Those constraints can be expressed as follows:

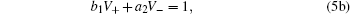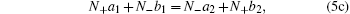where a₁, a₂, b₁, and b₂ are the detailed normalized density distributions of the M_F = 1 and M_F = – 1 components, and V₊ and V_– are the sizes of the two domain structures shown in Fig.  1(d).

By definition, the overlap factors are α ₀₊ = 1 + 1/β – α ₁/β , α _0– = 1 + β – β α ₁, and , where β = N₊ /N_– . For simplicity, let α = α ₁ = V∫ d³rη ₊ η _– in the following discussion. These factors are incorporated into the Hamiltonian, which after some simplification reads

where ℋ _s = H_s/(N| g₂/2| ), the total particle number is N = N₊ + N_– + N₀, n_a=N_a/N represents the fraction of the M_F = a component, and m = M/N indicates the spin imbalance. The spin evolution is described by the equations of motion

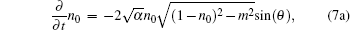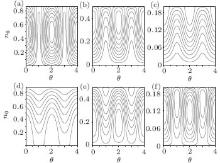
The easiest way to provide a global view of the spin dynamics is through the phase diagram of (n₀, θ ). In Fig.  2, we show phase diagrams with different coefficients m and α . There exist at most three distinctive dynamical regimes. Without initial magnetization (m = 0), only considering the effect of phase separation α , our previous study has already shown that the phase portrait would have two distinctive dynamical regimes, where the open curve, which we call the running phase, lies in the upper regime, and the closed curve representing the libration solution lies in the lower regime. As α decreases, the running phase becomes more dominant in the phase portrait. However, due to the effects of both α and m, a competition is observed based on the analysis of different phase portraits. As shown in Figs.  2(e) and 2(f), a third phase appears. If we had a greater initial spin imbalance (m), we would observe that this new emergent phase would become more dominant in the phase diagram with a fixed overlap factor (with α = 0.1). The emergent new dynamical regime is called the π phase, in which the time-averaged phase is equal to π .^[36]

Fig. 2.

	Figure Option ViewDownloadNew Window
	Fig. 2. Contour plots of the energy surface in the θ – n0 plane with different initial spin configurations and overlap factors: (a)m = 0.1, α = 1.0; (b) m = 0.5, α = 1.0; (c) m = 0.8, α = 0.1; (d) m = 0.1, α = 0.1; (e) m = 0.5, α = 0.1; (f) m = 0.8, α = 0.1.

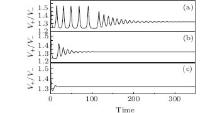
The dynamical evolutions corresponding to different overlap factors and different initial magnetizations are shown in Fig.  3, with all of the evolutions starting with n₀ = 1 – m. There are two overlap factors and two different initial spin configurations chosen: α = 1 corresponds to the former SMA-based theory, and α = 0.1 concerns the domain formation case. It can be observed that the domain formation has dramatic effects on the spin dynamics. For the m = 0 case, the phase separation can cause a large oscillation to the dynamical suppression. Conversely, for m = 0.8, a suppressed oscillation is transformed into a large oscillation with a much longer time period, which is remarkable.

Fig. 3.

	Figure Option ViewDownloadNew Window
	Fig. 3. Population of the spin-0 component as a function of time, initially with n1 = m, n– 1 = 0, and n0 = 1 – m. Different graphs corresponding to different initial magnetizations and overlap factors: (a) m = 0.1, α = 1.0; (b) m = 0.8, α = 1.0; (c) m = 0.1, α = 0.1; (d) m = 0.8, α = 0.1.

Within the framework of mean-field theory, the coherent dynamics of spinor condensates do not lead to the damping of spin oscillations. Therefore, here we use the method developed by Bloch^[37] to introduce a phenomenological term that accounts for the effect of the thermal agitation. This phenomenological term can change the energy of the system, and it also drives the system toward an equilibrium. 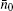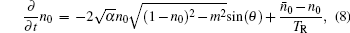Beginning with n₀ far away from its thermal equilibrium value , we assume that the value will relax to exponentially with a characteristic time T_R, called the relaxation time. Suppose that the relaxation velocity is proportional to , then the equation of state should be

where can be determined from the equations of motion ∂ n₀/∂ t = 0 and ∂ θ /∂ t = 0.

By adopting this relaxation mechanism, the spin dynamics of the domain formation with initial magnetization 0.1 and initial configuration ((0.5 + m)/2, 0.5, (0.5 – m)/2) are illustrated in Fig.  4. It can be observed that with two different choices of α , 0.5 and 0.1, the thermal dissipation causes the population oscillation to gradually become stationary, which corresponds to the ground state configuration of Hamiltonian (6) depending on the initial spin imbalance m and overlap factor α we have chosen. Quite remarkably, we note that for the two specific overlap factors we chose, the dynamical evolutions are quite different. For α = 0.1, a dissipation-enhanced coherent effect can be observed, ^[29] which only occurs after the strong oscillation period when the dissipation mechanism sets in. However, for a = 0.5, no such phase evolution period is observed. A closer look at the dynamical behavior reveals that the difference lies in the phase behavior. For α = 0.1, the phase θ is running at first, before it falls into the oscillation regime with energy dissipation. Based on the phase space trajectory analysis, when the thermal dissipation is accounted for, if the state begins within the running phase, the phase space trajectory would cross the phase boundary of the running phase regime to enter the oscillation dynamical regime. This dynamical change is referred to as separatrix crossing. However, for α = 0.5, the initial configuration is already in the oscillation regime, therefore, the phase would simply oscillate, not run (see the inset of Fig.  4). In summary, it is the separatrix crossing that causes the thermally enhanced coherent phase to enter the stabilizing phase.

The equilibrium configuration of spin populations has been discussed by several groups.^{[11, 12, 18]} Numerical simulations showed that the thermal effects cause the final portion of the spin-0 component^[15] to become smaller, which is consistent with the results of our previous study for the initially spin-balanced case, in which we predicted that the spin-0 component could be much less than 1/2 depending on the value of α , ^[29] for the final structure of domains as shown in Fig.  1(d). The V₊ versus V_– can be derived as follows: V₊ /V_– = (0.5t - 2α /t)(0.5t/β – 2α β /t), where β = N₊ N_– , and , the evolution of this ratio is illustrated in Fig.  5. The figure shows that there is a strong oscillation initially (an unsettled stage), and finally, the ratio reaches a constant approximately proportional to . The dynamical stabilization time varies with T_R. For strong dissipation, corresponding to a small T_R, the system reaches an equilibrium configuration faster than that in the case with a large T_R.

Fig. 4.

	Figure Option ViewDownloadNew Window
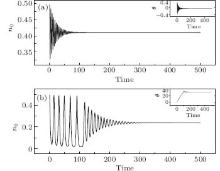	Fig. 4. Dynamical evolutions of the spin-0 component with spin imbalance m = 0.1, TR = 22, and different overlap factors: (a) α = 0.5, (b) α = 0.1.

Fig. 5.

	Figure Option ViewDownloadNew Window
	Fig. 5. The V+ /V– ratios of the two-domain structure for different overlap factors: (a) m = 0.1, a = 0.1, TR = 22; (b) m = 0.1, a = 0.1, TR = 10; (c) m = 0.1, a = 0.1, TR = 2.

In summary, considering the domain formation in ferromagnetic spin-1 condensates, we carried out research on the spin dynamics during the domain formation process with an arbitrary initial spin configuration. Using a phenomenological thermal relaxation term, we showed that the domain formation can be understood as a co-effect of quantum coherence and thermal relaxation. We also observed that a spin-imbalanced system has many different features compared with a spin-balanced one. With new dynamical regimes appearing with changes in the initial spin configuration and overlap factor, these features include π phase oscillations and a new running phase mode. Moreover, it was revealed that the coherent thermal enhancement effect is caused by separatrix crossing.