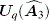
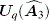
Corresponding author. E-mail: zhengzj@scut.edu.cn
Project supported by the National Natural Science Foundation of China (Grant No. 11475178).
In this paper, we prove one case of conjecture given by Hernandez and Leclerc. We give a cluster algebra structure on the Grothendieck ring of a full subcategory of the finite dimensional representations of affine quantum group
Affine quantum group (or quantum affine algebra) is a Hopf algebra that is a q-deformation of the universal enveloping algebra of an affine Lie algebra. They were introduced independently by Drinfeld and Jimbo as a special case of their general construction of a quantum group from a Cartan matrix.[1, 2] One of their principal applications has been the study of the YangBaxter equation, which is a necessary condition for the solvability of statistical models.
Cluster algebras are a class of commutative rings introduced by Fomin and Zelevinsky.[3, 5] A cluster algebra of rank n is an integral domain 𝒜 , together with some subsets of size n called clusters whose union generates the algebra 𝒜 . The clusters satisfy various conditions.
In Drinfeld's approach, quantum groups are universal enveloping algebras of certain Lie algebras, generally semisimple or affine. Given a Cartan matrix of a Lie algebra, a cluster algebra can be obtained. Naturally, affine quantum groups and cluster algebras are linked by Lie theory.
In recent years, there has been much interest in the studies of categorification of theories in fundamental mathematics and theoretical physics.[6, 12] In general, categorification is a process of replacing set-theoretic theorems by category-theoretic analogues. It replaces sets by categories, functions by functors, and equations between functions by natural transformations of functors. Categorification can be thought of as the process of enhancing an algebraic object to a more sophisticated one, while “ decategorification” is the process of reducing the categorified object back to the simpler original object. Thus, a useful categorification should possess a richer structure not seen in the underlying object. The categorification of physical theories may extend the mathematical structures of existing physical theories and help us solve the remaining problems in fundamental physics. This can also help us better understand the physical essence.
Khovanov and Lauda used the diagrammatic methods to study the categorification of quantum groups.[14] Khovanov has constructed a categorification of the Heisenberg algebra based on a graphical category that can act naturally on the category of representations of all symmetric groups, [13] and Cautis et al. have also carried out many significant works about the categorifications of the quantum Heisenberg algebras.[8]
In 2010, Hernandez and Leclerc defined the monoidal categorifications of cluster algebras and gave a model of monoidal category for certain cluster algebras.[15] An abelian monoidal category ℳ is a monoidal categorification of a cluster algebra 𝒜 if the Grothendieck ring of ℳ and 𝒜 are isomorphic as rings. The cluster monomials of 𝒜 are the classes of all the real simple objects of ℳ and the cluster variables of 𝒜 (including the frozen ones) are the classes of all the real prime simple objects of ℳ .
Hernandez and Leclerc conjectured that a series of certain full subcategories of finite dimensional representations of affine quantum group Uq(ĝ ) for simple Lie algebra g are monoidal categorifications of certain cluster algebras.[15]
We give a proof of the above conjecture in the case of g = sl4 for ℓ = 2 in the present work.
The paper is organized as follows. In Section 2, we give some notations and our main results. Some discussion is given in Section 3. In the appendix, we prove assistant Proposition 1. We give the polynomial ring isomorphism between cluster algebra 𝒜 and Grothendieck ring R2 first. Then, we calculate the truncated q-characters of all real prime objects. Lastly, we check the cluster monomials are the classes of all the real simple objects.
Let g be a simple Lie algebra of type ADE. We denote the vertex set of its Dynkin diagram by I, the corresponding affine quantum group by Uq(ĝ ) (parameter 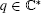

Denote the Grothendieck ring of 𝒞 ℓ by Rℓ , then 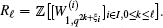
To study the finite-dimensional representations of Uq(ĝ ), Frenkel and Reshetikhin introduced q characters.[16] The morphism of q characters is an injective ring homomorphism from the Grothendieck ring R of 𝒞 to 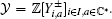
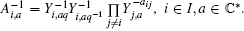
Let V be an object of 𝒞 and any subset J of I, χ q(V) has a unique decomposition[15]
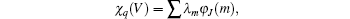
where m is J dominant, 


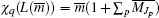
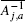

For 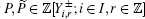
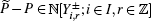
We define a useful polynomial N(m) ≤ χ q(L(m)) for dominant monomial m. We will see later that N(m) = χ q(L(m)) for some simple objects L(m).
(i) ∀ i ∈ I, by definition, φ i(m) can be written as such expression φ i(m) = m(1 + Σ Mip). Denote all such mMip by mip;
(ii) mip is j-dominant for j ∈ Ii, and φ j(mip) = mip (1 + Σ Mipk). Denote the monomials in φ j(mip) by mipk;
(iii) Repeat this procedure until every monomial contains all 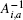
To simplify the notations, we write Yi, r and Ai, r instead of Yi, qr and Ai, qr respectively.
Let V be an object of 𝒞 2, χ q(V) = m(1 + Σ p Mp), where m is a dominant monomial in the variables Yi, ξ i, Yi, ξ i +2, Yi, ξ i+4 (i ∈ I), and the Mp are certain monomials in the 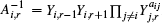
For this reason, we can define the truncated q-character χ q(V)≤ 4 of V as the polynomial obtained from χ q(V) by keeping the monomials Mp which do not contain any 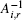
Using the mathematical method of Proposition 6.1 in Ref. [15], it can be proved that, the map 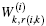
Similarly, we attach a polynomial FM(m)≤ 4 which is obtained from FM(m) by keeping only the monomials which do not contain any 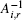
If L(m) is minuscule, then χ q(L(m)) = FM(m). That is, χ q(L(m)) and FM(m) have the same dominant monomials with the same multiplicities. Hence, we have
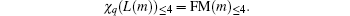
Lastly, we define N(m)≤ 4 as the polynomial which is obtained from N(m) by keeping only the monomials which do not contain any 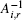
We have the following result of the cluster algebra of rank 6.
Proposition 1 If 𝒜 is the cluster algebra of rank 6 with initial seed (𝑥 , Γ ), where 𝑥 = (x0, … , x5, x6, x7, x8), x6, x7, x8 are frozen variables, Γ is

Then the map ι : 𝒜 → R2,
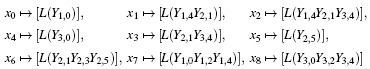
can be extended to a ring isomorphism. Furthermore, 𝒞 2 becomes a monoidal categorification of 𝒜 .
The proof of this proposition will be given in the appendix.
A partition of I = I0 ⊔ I1 is given by choosing I0 = {1, 3}. In order to distinguish the label of the vertices of Dynkin diagram E6 from A3, we denote the vertex set of Dynkin diagram E6 by Ĩ = [0, 5], and choose Ĩ 0 = {1, 3, 5}.
For a finite mutation sequence µ = µ 2µ 1µ 3µ 2µ 5µ 0µ 4, denote Γ 2 = µ (Γ ). If we ignore the label of vertices, Γ 2 is defined by
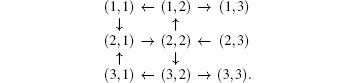
Let 𝒜 2 be the cluster algebra attached to the initial seed (𝑥 , Γ 2), where 𝑥 = (x(i, k) | i ∈ I, 1 ≤ k ≤ 3), x(i, 3) are frozen variables. By definition, 𝒞 2 is a monoidal categorification of 𝒜 if and only if 𝒞 2 is a monoidal categorification of 𝒜 2.
Thus, the above proposition is equivalent to the following statement:
Theorem 1 (Main result)

extends to a ring isomorphism ι from the cluster algebra 𝒜 2 to the Grothendieck ring R2 of 𝒞 2, where 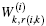
This is just the case of g = sl4 for ℓ = 2 in the conjecture given by Hernandez and Leclerc.[15]
In the case of ℓ = 1, for every simply laced g, the cluster type of Grothendieck ring R1 of 𝒞 1 coincide with the root system of g. However, this does not work for ℓ ≥ 2. In the case of ℓ ≥ 2, one needs to judge the compatibility of any two cluster variables. When a quiver is bipartite of type ADE, two cluster variables are compatible if and only if the corresponding two almost positive roots are compatible. Therefore, Proposition 1 plays an important role to obtain Theorem 2.
We mainly prove one case of Hernandez and Leclerc's conjecture.[15] This is a full subcategory of the finite-dimensional representations of affine quantum group 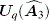
Some other conclusions are obtained in the proof. Since there are 45 cluster variables in the cluster algebra of type E6 with 3 frozen variables, the category 𝒞 2 has 45 real prime simple objects. The class of any simple object of 𝒞 2 in R2 is a cluster monomial. Besides, for every exchange relation of cluster algebra 𝒜 , there exists an exact sequence of 𝒞 2 corresponding to it. The set of such exact sequences includes the T systems and coincides with extended T systems defined in Ref. [18].
For example, for exchange relation x[− α 0]x[α 0]= x[− α 1] + x7, the corresponding exact sequence is
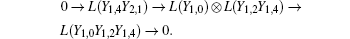
For exchange relation x[− α 2]x[α 0+ α 1+ α 2]= x[α 0+ α 1]x6+ x[α 0]x[− α 3]x[− α 5], the corresponding exact sequence is

Furthermore, L(Y3, 4) ⊗ L(Y2, 1Y2, 3Y2, 5) ≅ L(Y2, 1Y2, 3Y2, 5Y3, 4), L(Y1, 2Y1, 4)⊗ L(Y2, 5)⊗ L(Y2, 1Y3, 4) ≅ L(Y1, 2Y1, 4Y2, 1Y2, 5Y3, 4).
The cluster algebras 𝒜 ℓ defined in the conjecture are of finite type if and only if ℓ and the type of g satisfy one of the conditions: ℓ is arbitrary and g is of type A1; ℓ = 1 and g is of type ADE; g is of type An such that nℓ = 4, 6, 8.[15] In this paper, we consider the case of A3 for ℓ = 2. The remaining finite types can be proved in the same way. When the cluster algebra 𝒜 ℓ is of infinite type, 𝒜 ℓ has an infinite number of cluster variables and there exist simple objects of 𝒞 ℓ which are not real, our method does not work.
Since the calculations of the detailed expressions of cluster variables and compatible roots are mechanical and lengthy, we omit them here. We refer the readers to the expanded version of the present paper for more mathematical details.[19]
These results will help us study the simple objects of affine quantum groups and the categorifications of cluster algebras in mathematical physics.
| 1 |
|
| 2 |
|
| 3 |
|
| 4 |
|
| 5 |
|
| 6 |
|
| 7 |
|
| 8 |
|
| 9 |
|
| 10 |
|
| 11 |
|
| 12 |
|
| 13 |
|
| 14 |
|
| 15 |
|
| 16 |
|
| 17 |
|
| 18 |
|
| 19 |
|
| 20 |
|
| 21 |
|
| 22 |
|

